xFIP

Expected Fielding Independent Pitching (xFIP) is a regressed version of Fielding Independent Pitching (FIP), developed by Dave Studeman from The Hardball Times. It’s calculated in the same way as FIP, except it replaces a pitcher’s home run total with an estimate of how many home runs they should have allowed given the number of fly balls they surrendered while assuming a league average home run to fly ball percentage (between 9 and 10% depending on the year).

Home run rates are generally unstable over time and fluctuate around league-average, so by estimating a pitcher’s home run total, xFIP attempts to isolate a player’s ability level. A pitcher may allow home runs on 12% of their flyballs one year, then turn around and only allow 7% the next year. HR/FB ratios can be very difficult to predict because they contain a lot of noise, so xFIP attempts to correct for that and provide you with a sense of the pitcher’s underlying performance.

To learn more about why we’re interesting in Defense Independent Pitching Statistics (DIPS), check out our primer on FIP. This entry will walk you through the benefits of xFIP, assuming you have a basic understanding of DIPS and FIP.

Calculation:

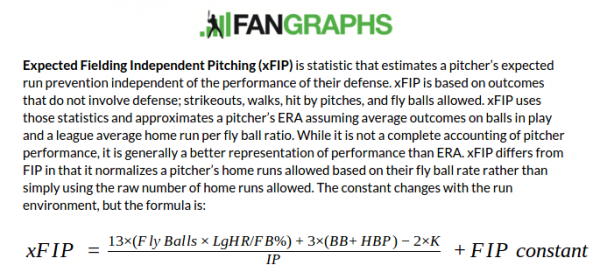
Here is the full formula for xFIP. Notice how it is almost exactly the same as the formula for FIP, with the lone difference being how each accounts for home runs. In traditional FIP, you would use the pitcher’s home run total, but in xFIP, you derive an expected number of home runs by taking the pitcher’s fly balls allowed multiplied by the league average home run per fly ball rate. League average HR/FB% is available here and only dates back to 2002.

xFIP = ((13*(Fly balls * lgHR/FB%))+(3*(BB+HBP))-(2*K))/IP + constant

The constant is solely to bring FIP and xFIP onto an ERA scale and is generally around 3.10. You can find historical FIP constants (which is the same as the xFIP constant) values here, or you can derive the constant yourself. Because FIP is designed so that league average ERA and league average FIP are the same, to find the constant for any year, all you need to do is the following:

FIP Constant = lgERA – (((13*lgHR)+(3*(lgBB+lgHBP))-(2*lgK))/lgIP)

Knowing how to calculate the constant can be especially useful if you’re interested in doing some of your own calculations for data spanning multiple seasons. The individual weights for home runs, walks/HBP, and strikeouts are based on the relative values of those actions with respect to run prevention.

Why xFIP:

While the value of moving from ERA to FIP is that it attempts to strip out defense, luck, and sequencing, moving from FIP to xFIP is useful because it tries to remove some of the randomness in the pitcher’s actual performance. Everything we do to calculate FIP is based on the idea that the pitcher is responsible for strikeouts, walks, hit batters, and home runs while the defense is not. This makes FIP a better indicator of pitcher performance than ERA.

However, we also know that the number of fly balls that go for home runs is very sensitive to sample size meaning that over the course of a season, the number of home runs a pitcher allows may be higher or lower than their true talent indicates. This is not to say pitcher’s aren’t responsible for the home runs they did allow, but rather to say that if you want to judge about how well they pitched, xFIP will remove some of those fluctuations in HR/FB% and will give you a better idea. For this reason, our pitcher Wins Above Replacement (WAR) is based on FIP rather than xFIP. They gave up the home runs so they count against them, but xFIP suggests they probably won’t continue to do so in the future.

To give you an idea, let’s imagine a pitcher who threw 200 innings, struck out 200, walked and hit 60, gave up 24 home runs, and 240 fly balls. This pitcher would have a FIP of 3.56 (if we assume a 3.10 FIP constant). This pitcher has a league average HR/FB%, so we can also say their xFIP is 3.56.

Now imagine if during the course of this season, this pitcher allowed five more home runs to carry the fence. That’s not even one extra home run per month.That turns into a 3.89 FIP, but the pitcher’s xFIP remains 3.56. In the first scenario, the pitcher has a 10% HR/FB% and in the second scenario it’s 12%. That may not seem like a big gap, but it is. And we also know that these rates are not typically very stable over time, which means that there is an awful lot of random noise involved. When discussing a pitcher’s past value, those home runs should count against them, but if you want to evaluate their underlying performance, knowing their fly ball rate is more useful.

As a result, xFIP strips out some of this fluctuation to give you a better view of how well we think a pitcher pitched over a given period of time, while controlling for defense, batted ball luck, and sequencing, and also HR/FB%. In other words, we use xFIP to see how a pitcher might be expected to perform given an average HR/FB% because we do not expect pitchers to have much control over that number. They can control how many fly balls they allow, but only a limited set of pitchers can truly influence their HR/FB%. This makes xFIP a very useful statistic if used properly.

How to Use xFIP:

Using xFIP is both extremely easy and moderately complex. From a simple perspective, xFIP is on an ERA scale, so you can apply what you know about ERA and FIP to xFIP and have a good sense of what a given value means. A player with a 3.00 xFIP is just as good as you think a player with a 3.00 ERA is. The scale is intentionally identical, so reading xFIP is a snap.

Using it appropriately when analyzing players requires a bit more caution. First, it’s not as simple as saying Pitcher A has a 3.40 ERA and FIP and a 3.70 xFIP, so he is due for regression. While xFIP is usually more predictive of future performance, there are reasons why a pitcher might not be expected to pitch to that particular xFIP.

First, some pitchers can control their HR/FB% to some degree. Generally speaking, we expect most pitchers to approach a league average rate (About 10% most years), but some pitchers can consistently posts values around 8% and some can go as high as 12%. This is different than saying HR/FB% bounces around based on random variation. Some pitchers do the the ability to limit their HR/FB%, so being aware of your particular pitcher’s skill set is important. If a pitcher has routinely posted 9% HR/FB%, there’s a decent chance xFIP is underrating him a bit.

Additionally, xFIP is a predictive model based on just one year of data or however many years you incorporate and every event is weighted equally. In this way, it is not better than a legitimate effort to forecast or project a pitcher’s ERA or FIP. You can think of it as a very basic forecast, but a proper forecast will include multiple years of data and will weight recent events differently than older events.

Despite it’s limitations in those two regards, xFIP is a terrific way to get a sense of how well a pitcher’s been throwing the ball. xFIP tells us about a pitcher’s strikeout and walk rates, which are very important, and also inherently provides us with information about their batted ball profiles because fly ball rate is built into the model.

In a very simple sense, FIP tells you how a pitcher has performed (value) independent of their defense while xFIP tells you about how well he has pitched (ability, talent) independent of their defense. Do not rush to assume a pitcher’s xFIP is a better reflection of their talent, but using it to get a sense of their abilities in conjunction with other statistics will make you much better off.

Context:

Please note that the following chart is meant as an estimate, and that league-average xFIP varies on a year-by-year basis so that it is always the same as league-average ERA. To see the league-average xFIP for every year from 1901 to the present, check the FanGraphs leaderboards.

Rating	FIP
Excellent	2.90
Great	3.20
Above Average	3.50
Average	3.80
Below Average	4.10
Poor	4.40
Awful	4.70

Things to Remember:

● xFIP is not park or league adjusted. We carry a park and league adjusted version called xFIP-, found in the “Advanced” tab of the leaderboards and player pages.

● xFIP only dates back to 2002, the first year we have reliable HR/FB% data. Sorry, you can’t find Sandy Koufax’s xFIP.

● While HR/FB ratios are generally unstable over time, some pitchers are still more prone to allowing home runs than others. If a pitcher has a long history of over- or under-performing the league average with their HR/FB rate, then you can reasonably expect them to perform closer to their career average than the league-average. In cases like this, xFIP may overestimate or underestimate a player’s true talent level by assuming a league average HR/FB ratio. For more, see SIERA.

● Ground ball pitchers typically have higher HR/FB ratios than fly ball pitchers.

● xFIP has one of the highest correlations with future ERA of all the pitching metrics.

● While xFIP is more predictive than FIP, we use FIP to build WAR because those home runs were the responsibility of the pitcher and not the defense, which WAR hopes to strip out.

Links for Further Reading:

Batty for Baseball Stats – Hardball Times

Better with Less: ERA Estimators – Baseball Prospectus

Bannister Touts xFIP – Kansas City Star

xFIP Calculator – New English D