Probability theory is an advanced branch of mathematics that deals with measuring the likelihood of events occurring. It provides tools to analyze situations involving uncertainty and helps in determining how likely certain outcomes are. This theory uses the concepts of random variables, sample space, probability distributions, and more to determine the outcome of any situation.
For Example: Flipping a Coin
Flipping a coin is a random event with two possible outcomes: heads or tails. Each time you flip a fair coin, there are exactly two possible outcomes, each with an equal chance of occurring. Therefore, the probability of landing on heads is 1/2, and similarly, the probability of landing on tails is also 1/2.
Different Approaches In Probability Theory
Probability theory studies random events and tells us about their occurrence. The three main approaches for studying probability theory are:
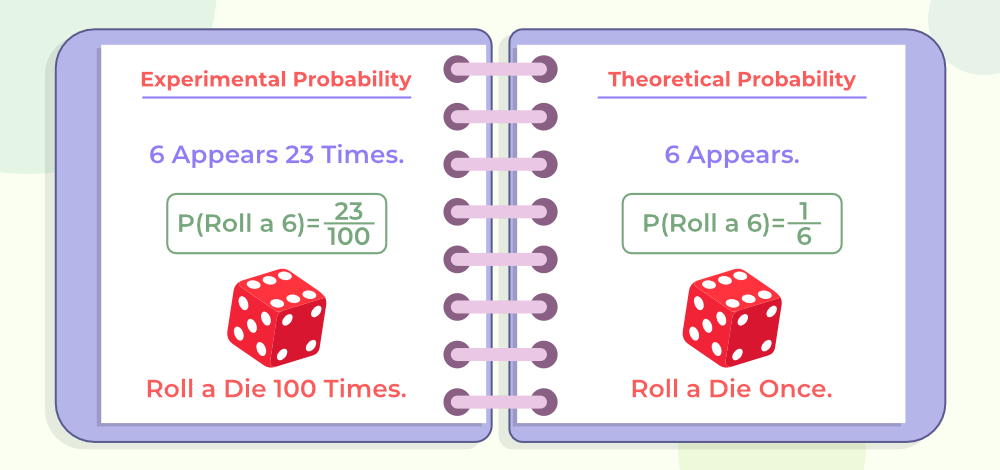
Theoretical Probability
Theoretical Probability deals with assumptions to avoid unfeasible or costly repetition of experiments. The theoretical Probability for an event A can be calculated as follows:
P(A) = (Number of outcomes favorable to Event A) / (Number of all possible outcomes)
The image shown below shows the theoretical probability formula.
.png)
Note: Here we assume the outcomes of an event as equally likely.
Now, as we learn the formula, let’s put this formula in our coin-tossing case. In tossing a coin, there are two outcomes: Head or Tail. Hence, The Probability of occurrence of Head on tossing a coin is P(H) = 1/2
Similarly, The Probability of the occurrence of a Tail on tossing a coin is P(T) = 1/2
Experimental Probability
Experimental probability is found by performing a series of experiments and observing their outcomes. These random experiments are also known as trials. The experimental probability for Event A can be calculated as follows:
P(E) = (Number of times event A happened) / (Total number of trials)
The following image shows the Experimental Probability Formula,
.png)
Now, as we learn the formula, let’s put this formula in our coin-tossing case. If we tossed a coin 10 times and recorded heads for 4 times and a tail 6 times then the Probability of occurrence of Head on tossing a coin: P(H) = 4/10
Similarly, the Probability of Occurrence of Tails on tossing a coin: P(T) = 6/10
Subjective Probability
Subjective probability refers to the likelihood of an event occurring, as estimated by an individual based on their personal beliefs, experiences, intuition, or knowledge, rather than on objective statistical data or formal mathematical models.
Example: A cricket enthusiast might assign a 70% probability to a team’s victory based on their understanding of the team’s recent form, the opponent’s strengths and weaknesses, and other relevant factors.
Basics of Probability Theory
Random Experiment
In probability theory, any event that can be repeated multiple times and its outcome is not hampered by its repetition is called a Random Experiment. Tossing a coin, rolling dice, etc. are random experiments.
Sample Space
The set of all possible outcomes for any random experiment is called sample space. For example, throwing dice results in six outcomes, which are 1, 2, 3, 4, 5, and 6. Thus, its sample space is (1, 2, 3, 4, 5, 6)
Event
The outcome of any experiment is called an event. Various types of events used in probability theory are,
- Independent Events: The events whose outcomes are not affected by the outcomes of other future and/or past events are called independent events. For example, the output of tossing a coin in repetition is not affected by its previous outcome.
- Dependent Events: The events whose outcomes are affected by the outcome of other events are called dependent events. For example, picking oranges from a bag that contains 100 oranges without replacement.
- Mutually Exclusive Events: The events that can not occur simultaneously are called mutually exclusive events. For example, obtaining a head or a tail in tossing a coin, because both (head and tail) can not be obtained together.
- Equally likely Events: The events that have an equal chance or probability of happening are known as equally likely events. For example, observing any face in rolling dice has an equal probability of 1/6.
Random Variable
A variable that can assume the value of all possible outcomes of an experiment is called a random variable in Probability Theory. Random variables in probability theory are of two types which are discussed below,
Discrete Random Variable
Variables that can take countable values such as 0, 1, 2,… are called discrete random variables.
Continuous Random Variable
Variables that can take an infinite number of values in a given range are called continuous random variables.
Various formulas are used in probability theory and some of them are discussed below,
- Theoretical Probability Formula: (Number of Favourable Outcomes) / (Number of Total Outcomes)
- Empirical Probability Formula: (Number of times event A happened) / (Total number of trials)
- Addition Rule of Probability: P(A ∪ B) = P(A) + P(B) – P(A∩B)
- Complementary Rule of Probability: P(A’) = 1 – P(A)
- Independent Events: P(A∩B) = P(A) ⋅ P(B)
- Conditional Probability: P(A | B) = P(A∩B) / P(B)
- Bayes’ Theorem: P(A | B) = P(B | A) ⋅ P(A) / P(B)
Read in Detail: Bayes Theorem
Probability Theory in Statistics
Probability has various applications in Statistics. These are mentioned below:
- Descriptive Statistics: Probability theory helps in understanding and interpreting data summaries and distributions.
- Inferential Statistics: This forms the basis for making inferences about populations from samples, including hypothesis testing and the construction of confidence intervals.
- Regression Analysis: Probability distributions of errors are used to estimate the relationships between variables.
- Bayesian Statistics: Uses probability to represent uncertainty about the parameters of interest and updates this uncertainty as more data becomes available.
Applications of Probability Theory
Probability theory is widely used in our lives, it is used to find answers to various types of questions, such as Will it rain tomorrow? What is the chance of landing on the Moon? What is the chance of the evolution of humans? And others. Some of the important uses of probability theory are,
- Probability theory is used to predict the performance of stocks and bonds.
- In casinos and gambling probability theory is used to find the chances of winning.
- Probability theory is used in weather forecasting.
- Probability theory is used in Risk mitigation.
- In consumer industries, the risk of product failure is mitigated by using the Probability theory.
Solved Examples of Probability Theory
We can study the concept of probability with the help of the example discussed below,
Example 1: Let’s take two random dice and roll them randomly, now the probability of getting a total of 10 is calculated.
Solution:
Total Possible events that can occur (sample space) {(1,1), (1,2),…, (1,6),…, (6,6)}. The total spaces are 36.
Now the required events, {(4,6), (5,5), (6,4)} are all which adds up to 10.
So the probability of getting a total of 10 is = 3/36 = 1/12
Example 2: A fair coin is tossed three times. What is the probability of getting exactly two heads?
Solution:
Total possible outcomes when tossing a coin three times = 23 = 8.
Possible outcomes: HHH, HHT, HTH, THH, HTT, THT, TTH, TTT.
Outcomes with exactly two heads: HHT, HTH, THH (3 outcomes).
Probability of getting exactly two heads:
P(exactly 2 heads)=Number of favorable outcomes/ Total outcomes.
P(exactly 2 heads)=3/ 8.
Example 3: A standard deck of cards contains 52 cards. What is the probability of drawing an Ace or a King from the deck?
Solution:
Total number of cards = 52.
Number of Aces = 4.
Number of Kings = 4.
Total number of favorable outcomes (Aces or Kings) = 4 + 4 = 8.
Probability of drawing an Ace or a King:
P(Ace or King)=Number of favorable outcomes/Total outcomes
P(Ace or King)=Number of Aces or Kings/Total number of cards.
P(Ace or King) = 8/52 = 2/13.
Example 4: Consider a jar with 7 red marbles, 3 green marbles, and 4 blue marbles. What is the probability of randomly selecting a non-blue marble from the jar?
Solution:
Given,
Number of Red Marbles = 7, Number of Green Marbles = 3, Number of Blue Marbles = 4
So, Total number of possible outcomes in this case: 7 + 3 + 4 = 14
Now, Number of non-blue marbles are: 7 + 3 = 10
According to the formula of theoretical Probability we can find, P(Non-Blue) = 10/14 = 5/7
Hence, theoretical probability of selecting a non-blue marble is 5/7.
Example 5: Consider Two players, Naveena and Isha, playing a table tennis match. The probability of Naveena winning the match is 0.76. What is the probability of Isha winning the match?
Solution:
Let N and M represent the events that Naveena wins the match and Isha wins the match, respectively.
The probability of Naveena’s winning P(N) = 0.62 (given)
The probability of Isha’s winning P(I) = ?
Winning of the match is an mutually exclusive event, since only one of them can win the match.
Therefore,
P(N) + P(I) =1
P(I) = 1 – P(N)
P(I) = 1 – 0.62 = 0.38
Thus, the Probability of Isha winning the match is 0.38.
Example 6: If someone takes out one card from a 52-card deck, what is the probability of the card being a heart? What is the probability of obtaining a 7-number card?
Solution:
Total number of cards in a deck = 52
Total Number of heart cards in a deck = 13
So, the probability of obtaining a heart,
P(heart) = 13/52 = 1/4
Total number of 7-number cards in a deck = 4
So, the probability of obtaining a 7-number card,
P(7-number) = 4/52 = 1/13
Example 7: Find the probability of rolling an even number when you roll a die containing the numbers 1-6. Express the probability as a fraction, decimal, ratio, or percent.
Solution:
Out of 1 to 6 number, even numbers are 2, 4, and 6.
So, Number of favorable outcomes = 3.
Total number of outcomes = 6.
Probability of obtaining an even number P(Even)= 1/2 = 0.5 = 1 : 2 = 50%
People Also Read:
Probability Theory – FAQs
What is the concept of Probability Theory?
The branch of mathematics that deals with the odds of happening an event is called the Probability Theory. It tells us about the odds of occurring an event and also all the possible outcomes of any event.
What are the two types of probabilities in Probability Theory?
The two types of probabilities in probability theory are
- Theoretical Probability
- Experimental probability
Who invented probability theory?
The credit for the creation of modern probability theory goes to Intalian mathematics Gerolamo Cardano.
What is a random variable in Probability Theory?
A variable that describes all the possible outcomes of any random experiment is called a random variable. A random variable can either be continuous or discrete.
The main formulas used in probability theory are,
- Theoretical probability: (Number of outcomes favourable to Event A) / (Number of all possible outcomes)
- Experimental Probability: (Number of times event A happened) / (Total number of trials)
Similar Reads
CBSE Class 10 Maths Notes PDF: Chapter Wise Notes 2024
Math is an important subject in CBSE Class 10th Board exam. So students are advised to prepare accordingly to score well in Mathematics. Mathematics sometimes seems complex but at the same time, It is easy to score well in Math. So, We have curated the complete CBSE Class 10 Math Notes for you to pr
15+ min read
Chapter 1: Real Numbers
Real Numbers
Real Numbers are continuous quantities that can represent a distance along a line, as Real numbers include both rational and irrational numbers. Rational numbers occupy the points at some finite distance and irrational numbers fill the gap between them, making them together to complete the real line
10 min read
Euclid Division Lemma
Euclid's Division Lemma which is one of the fundamental theorems proposed by the ancient Greek mathematician Euclid which was used to prove various properties of integers. The Euclid's Division Lemma also serves as a base for Euclid's Division Algorithm which is used to find the GCD of any two numbe
5 min read
Fundamental Theorem of Arithmetic
The Fundamental Theorem of Arithmetic is a useful method to understand the prime factorization of any number. The factorization of any composite number can be uniquely written as a multiplication of prime numbers, regardless of the order in which the prime factors appear. The figures above represent
7 min read
HCF / GCD and LCM - Definition, Formula, Full Form, Examples
The Highest Common Factor (HCF), also known as the Greatest Common Divisor (GCD), and Lowest Common Multiple (LCM) are fundamental concepts in mathematics, especially in number theory and arithmetic. The full form of HCF is the Highest Common Factor while the full form of LCM is the Least Common Mul
11 min read
Irrational Numbers- Definition, Examples, Symbol, Properties
Irrational numbers are real numbers that cannot be expressed as fractions. Irrational Numbers can not be expressed in the form of p/q, where p and q are integers and q ≠ 0. They are non-recurring, non-terminating, and non-repeating decimals. Irrational numbers are real numbers but are different from
12 min read
Decimal Expansions of Rational Numbers
Real numbers are simply the combination of rational and irrational numbers, in the number system. In general, all the arithmetic operations can be performed on these numbers and they can be represented in the number line, also. So in this article let's discuss some rational and irrational numbers an
6 min read
Rational Numbers
Rational numbers are a fundamental concept in mathematics, defined as numbers that can be expressed as the ratio of two integers, where the denominator is not zero. Represented in the form p/q (with p and q being integers), rational numbers include fractions, whole numbers, and terminating or repea
15+ min read
Chapter 2: Polynomials
Algebraic Expressions in Math: Definition, Example and Equation
Algebraic Expression is a mathematical expression that is made of numbers, and variables connected with any arithmetical operation between them. Algebraic forms are used to define unknown conditions in real life or situations that include unknown variables. An algebraic expression is made up of term
8 min read
Polynomial Formula
Polynomial Formula gives the standard form of polynomial expressions. It specifies the arrangement of algebraic expressions according to their increasing or decreasing power of variables. Let's learn about various polynomial formulas and identities in detail. Table of Content What is Polynomial?Type
6 min read
Types of Polynomials (Based on Terms and Degrees)
Types of Polynomials: In mathematics, an algebraic expression is an expression built up from integer constants, variables, and algebraic operations. There are mainly four types of polynomials based on degree-constant polynomial (zero degree), linear polynomial ( 1st degree), quadratic polynomial (2n
9 min read
Zeros of Polynomial
Zeros of a Polynomial are those real, imaginary, or complex values when put in the polynomial instead of a variable, the result becomes zero (as the name suggests zero as well). Polynomials are used to model some physical phenomena happening in real life, they are very useful in describing situation
14 min read
Geometrical meaning of the Zeroes of a Polynomial
An algebraic identity is an equality that holds for any value of its variables. They are generally used in the factorization of polynomials or simplification of algebraic calculations. A polynomial is just a bunch of algebraic terms added together, for example, p(x) = 4x + 1 is a degree-1 polynomial
8 min read
Factorization of Polynomial
Factorization is the process in which we can find factors of either a given number or the algebraic expression using various techniques such as prime factorization, factorization using algebraic identities, and factorization of a quadratic polynomial using the middle term splitting method. Factoriza
12 min read
Division Algorithm for Polynomials
Polynomials are those algebraic expressions that contain variables, coefficients, and constants. For Instance, in the polynomial 8x2 + 3z - 7, in this polynomial, 8,3 are the coefficients, x and z are the variables, and 7 is the constant. Just as simple Mathematical operations are applied on numbers
5 min read
Algebraic Identities
Algebraic Identities are fundamental equations in algebra where the left-hand side of the equation is always equal to the right-hand side, regardless of the values of the variables involved. These identities play a crucial role in simplifying algebraic computations and are essential for solving vari
14 min read
Relationship between Zeroes and Coefficients of a Polynomial
Polynomials are algebraic expressions with constants and variables that can be linear i.e. the highest power o the variable is one, quadratic and others. The zeros of the polynomials are the values of the variable (say x) that on substituting in the polynomial give the answer as zero. While the coef
9 min read
Division Algorithm Problems and Solutions
Polynomials are made up of algebraic expressions with different degrees. Degree-one polynomials are called linear polynomials, degree-two are called quadratic and degree-three are called cubic polynomials. Zeros of these polynomials are the points where these polynomials become zero. Sometimes it ha
6 min read
Chapter 3: Pair of Linear equations in two variables
Linear Equation in Two Variables
Linear Equation in Two Variables: A Linear equation is defined as an equation with the maximum degree of one only, for example, ax = b can be referred to as a linear equation, and when a Linear equation in two variables comes into the picture, it means that the entire equation has 2 variables presen
9 min read
Pair of Linear Equations in Two Variables
Linear Equation in two variables are equations with only two variables and the exponent of the variable is 1. This system of equations can have a unique solution, no solution, or an infinite solution according to the given initial condition. Linear equations are used to describe a relationship betwe
11 min read
Graphical Methods of Solving Pair of Linear Equations in Two Variables
A system of linear equations is just a pair of two lines that may or may not intersect. The graph of a linear equation is a line. There are various methods that can be used to solve two linear equations, for example, Substitution Method, Elimination Method, etc. An easy-to-understand and beginner-fr
8 min read
Solve the Linear Equation using Substitution Method
Solving Linear Equation using the Substitution Method: The equation in which the highest power of the variable is always 1 is called a linear equation (or) the first-order equation. A linear equation's graph will always be a straight line. When the equation has only one variable and the highest degr
12 min read
Solving Linear Equations Using the Elimination Method
If an equation is written in the form ax + by + c = 0, where a, b, and c are real integers and the coefficients of x and y, i.e. a and b, are not equal to zero, it is said to be a linear equation in two variables. For example, 3x + y = 4 is a linear equation in two variables- x and y. The numbers th
10 min read
Chapter 4: Quadratic Equations
Quadratic Equations
A Quadratic equation is a second-degree polynomial equation that can be represented as ax2 + bx + c = 0. In this equation, x is an unknown variable, a, b, and c are constants, and a is not equal to 0. The solutions of a quadratic equation are known as its roots. These roots can be found using method
11 min read
Roots of Quadratic Equation
Roots of Quadratic Equations are the values of the variable for which the quadratic equation gets satisfied. The roots of a quadratic equation are also called zeros of a quadratic equation. A quadratic equation is an equation in which the maximum power of a variable is 2. Hence, there can be a maxim
13 min read
Solving Quadratic Equations
A quadratic equation, typically in the form ax² + bx + c = 0, can be solved using different methods including factoring, completing the square, quadratic formula, and the graph method. While Solving Quadratic Equations we try to find a solution that represent the points where this the condition Q(x)
8 min read
How to find the Discriminant of a Quadratic Equation?
Algebra can be defined as the branch of mathematics that deals with the study, alteration, and analysis of various mathematical symbols. It is the study of unknown quantities, which are often depicted with the help of variables in mathematics. Algebra has a plethora of formulas and identities for th
4 min read
Chapter 5: Arithmetic Progressions
Arithmetic Progressions Class 10- NCERT Notes
Arithmetic Progressions (AP) are fundamental sequences in mathematics where each term after the first is obtained by adding a constant difference to the previous term. Understanding APs is crucial for solving problems related to sequences and series in Class 10 Mathematics. These notes cover the ess
7 min read
Sequences and Series
A sequence is an ordered list of numbers following a specific rule. Each number in a sequence is called a "term." The order in which terms are arranged is crucial, as each term has a specific position, often denoted as an, where n indicates the position in the sequence. For example: 2, 5, 8, 11, 14
7 min read
Arithmetic Progression
Arithmetic progression (AP) is simply a sequence of numbers such that the difference between any two consecutive terms is constant. Some Real World Examples of AP Natural Numbers: 1, 2, 3, 4, 5, . . . with a common difference 1Even Numbers: 2, 4, 6, 8, 10, . . . with a common difference 2Odd Numbers
6 min read
Arithmetic Progression - Common difference and Nth term | Class 10 Maths
Arithmetic Progression is a sequence of numbers where the difference between any two successive numbers is constant. For example 1, 3, 5, 7, 9....... is in a series which has a common difference (3 - 1) between two successive terms is equal to 2. If we take natural numbers as an example of series 1,
5 min read
How to find the nth term of an Arithmetic Sequence?
Answer: Nth term of an Arithmetic Sequence, an = a1 + (N-1)d ⇢ [First term + (Last term - 1)×common difference]Arithmetic is a part of mathematics that works with different types of numbers, and fractions, applying different operations on numbers like addition, multiplication, etc. The word Arithmet
7 min read
Arithmetic Progression | Sum of First n Terms | Class 10 Maths
Arithmetic Progression is a sequence of numbers where the difference between any two successive numbers is constant. For example 1, 3, 5, 7, 9……. is in a series which has a common difference (3 – 1) between two successive terms is equal to 2. If we take natural numbers as an example of series 1, 2,
8 min read
Arithmetic Mean
Arithmetic Mean, commonly known as the average, is a fundamental measure of central tendency in statistics. It is defined as the ratio of all the values or observations to the total number of values or observations. Arithmetic Mean is one of the fundamental formulas used in mathematics and it is hig
13 min read
Arithmetic Progression | Sum of First n Terms | Class 10 Maths
Arithmetic Progression is a sequence of numbers where the difference between any two successive numbers is constant. For example 1, 3, 5, 7, 9……. is in a series which has a common difference (3 – 1) between two successive terms is equal to 2. If we take natural numbers as an example of series 1, 2,
8 min read
Chapter 6: Triangles
Triangles in Geometry
A triangle is a polygon with three sides (edges), three vertices (corners), and three angles. It is the simplest polygon in geometry, and the sum of its interior angles is always 180°. A triangle is formed by three line segments (edges) that intersect at three vertices, creating a two-dimensional re
13 min read
Similar Triangles
Similar Triangles are triangles with the same shape but can have variable sizes. Similar triangles have corresponding sides in proportion to each other and corresponding angles equal to each other. Similar triangles are different from congruent triangles. Two congruent figures are always similar, bu
15+ min read
Criteria for Similarity of Triangles
Things are often referred similar when the physical structure or patterns they show have similar properties, Sometimes two objects may vary in size but because of their physical similarities, they are called similar objects. For example, a bigger Square will always be similar to a smaller square. In
9 min read
Basic Proportionality Theorem (BPT) Class 10 | Proof and Examples
Basic Proportionality Theorem: Thales theorem is one of the most fundamental theorems in geometry that relates the parts of the length of sides of triangles. The other name of the Thales theorem is the Basic Proportionality Theorem or BPT. BPT states that if a line is parallel to a side of a triangl
8 min read
Pythagoras Theorem | Formula, Proof and Examples
Pythagoras Theorem explains the relationship between the three sides of a right-angled triangle and helps us find the length of a missing side if the other two sides are known. It is also known as the Pythagorean theorem. It states that in a right-angled triangle, the square of the hypotenuse is equ
10 min read
Chapter 7: Coordinate Geometry
Coordinate Geometry
Coordinate geometry is the branch of mathematics that deals with plotting the curve on the coordinate axes. Various curves can be plotted on the coordinate plane using coordinate geometry formulas. Co-ordinate geometry uses algebraic equations to plot various curves on the coordinate plane. One of t
11 min read
Distance Formula Class 10 Maths
The distance formula is one of the important concepts in coordinate geometry which is used widely. By using the distance formula we can find the shortest distance i.e drawing a straight line between points. There are two ways to find the distance between points: Pythagorean theoremDistance formulaTa
9 min read
Distance Between Two Points
Distance Between Two Points is the length of line segment that connects any two points in a coordinate plane in coordinate geometry. It can be calculated using a distance formula for 2D or 3D. It represents the shortest path between two locations in a given space. In this article, we will learn how
6 min read
Section Formula
Section Formula is a useful tool in coordinate geometry, which helps us find the coordinate of any point on a line which is dividing the line into some known ratio. Suppose a point divides a line segment into two parts which may be equal or not, with the help of the section formula we can find the c
15 min read
How to find the ratio in which a point divides a line?
Answer: To find the ratio in which a point divides a line we use the following formula [Tex]x = \frac{m_1x_2+m_2x_1}{m_1+m_2} [/Tex][Tex]y = \frac{m_1y_2+m_2y_1}{m_1+m_2}[/Tex]Geo means Earth and metry means measurement. Geometry is a branch of mathematics that deals with distance, shapes, sizes, r
4 min read
How to find the Trisection Points of a Line?
To find the trisection points of a line segment, you need to divide the segment into three equal parts. This involves finding the points that divide the segment into three equal lengths. In this article, we will answer "How to find the Trisection Points of a Line?" in detail including section formul
4 min read
How to find the Centroid of a Triangle?
Answer: The Centroid for the triangle is calculated using the formula[Tex]\left (\frac{[x1+x2+x3]}{3}, \frac{[y1+y2+y3]}{3}\right)[/Tex]A triangle consists of three sides and three interior angles. Centroid refers to the center of an object. Coming to the centroid of the triangle, is defined as the
4 min read
Area of a Triangle in Coordinate Geometry
Coordinate geometry is defined as the study of geometry using the coordinate points on the plane with any dimension. Using coordinate geometry, it is possible to find the distance between two points, divide lines in a ratio, find the mid-point of a line, calculate the area of a triangle in the Carte
8 min read
Chapter 8: Introduction to Trigonometry
Trigonometric Ratios
There are three sides of a triangle Hypotenuse, Adjacent, and Opposite. The ratios between these sides based on the angle between them is called Trigonometric Ratio. The six trigonometric ratios are: sine (sin), cosine (cos), tangent (tan), cotangent (cot), cosecant (cosec), and secant (sec). As giv
4 min read
Unit Circle: Definition, Formula, Diagram and Solved Examples
Unit Circle is a Circle whose radius is 1. The center of unit circle is at origin(0,0) on the axis. The circumference of Unit Circle is 2π units, whereas area of Unit Circle is π units2. It carries all the properties of Circle. Unit Circle has the equation x2 + y2 = 1. This Unit Circle helps in defi
7 min read
Trigonometric Ratios of Some Specific Angles
Trigonometry is all about triangles or to be more precise the relationship between the angles and sides of a triangle (right-angled triangle). In this article, we will be discussing the ratio of sides of a right-angled triangle concerning its acute angle called trigonometric ratios of the angle and
6 min read
Trigonometric Identities
Trigonometric identities play an important role in simplifying expressions and solving equations involving trigonometric functions. These identities, which involve relationships between angles and sides of triangles, are widely used in fields like geometry, engineering, and physics. In this article,
11 min read
Chapter 9: Some Applications of Trigonometry
Chapter 11: Constructions
Chapter 12: Areas related to circles
Chapter 13: Surface Areas and Volume
Surface Area of Cuboid
The surface area of a cuboid is the total space occupied by all its surfaces/sides. In geometry, a three-dimensional shape having six rectangular faces is called a cuboid. A cuboid is also known as a regular hexahedron and has six rectangular faces, eight vertices, and twelve edges with congruent, o
12 min read
Volume of Cuboid | Formula and Examples
Volume of a cuboid is calculated using the formula V = L × B × H, where V represents the volume in cubic units, L stands for length, B for breadth, and H for height. Here, the breadth and width of a cuboid are the same things. The volume signifies the amount of space occupied by the cuboid in three
8 min read
Surface Area of Cube | Curved & Total Surface Area
Surface area of a cube is defined as the total area covered by all the faces of a cube. In geometry, the cube is a fascinating three-dimensional object that we encounter daily, from dice to ice cubes. But have you ever wondered about the total area that covers a cube? This is what we call the surfac
15 min read
Volume of a Cube
Volume of a Cube is defined as the total number of cubic units occupied by the cube completely. A cube is a three-dimensional solid figure, having 6 square faces. Volume is nothing but the total space occupied by an object. An object with a larger volume would occupy more space. The volume of the cu
9 min read
Surface Area of Cylinder | Curved and Total Surface Area of Cylinder
Surface Area of a Cylinder is the amount of space covered by the flat surface of the cylinder's bases and the curved surface of the cylinder. The total surface area of the cylinder includes the area of the cylinder's two circular bases as well as the area of the curving surface. The volume of a cyli
10 min read
Volume of a Cylinder| Formula, Definition and Examples
Volume of a cylinder is a fundamental concept in geometry and plays a crucial role in various real-life applications. It is a measure which signifies the amount of material the cylinder can carry. It is also defined as the space occupied by the Cylinder. The formula for the volume of a cylinder is π
11 min read
Surface Area of Cone
Surface Area of a Cone is the total area encompassing the circular base and the curved surface of the cone. A cone has two types of surface areas. If the radius of the base is 'r' and the slant height is 'l', we use two formulas: Total Surface Area (TSA) of the cone = πr(r + l)Curved Surface Area (C
8 min read
Volume of Cone- Formula, Derivation and Examples
Volume of a cone can be defined as the space occupied by the cone. As we know, a cone is a three-dimensional geometric shape with a circular base and a single apex (vertex). Let's learn about Volume of Cone in detail, including its Formula, Examples, and the Frustum of Cone. Volume of ConeA cone's v
10 min read
Surface Area of Sphere | Formula, Derivation and Solved Examples
A sphere is a three-dimensional object with all points on its surface equidistant from its center, giving it a perfectly round shape. The surface area of a sphere is the total area that covers its outer surface. To calculate the surface area of a sphere with radius r, we use the formula: Surface Are
8 min read
Volume of a Sphere
The volume of a sphere helps us understand how much space a perfectly round object occupies, from tiny balls to large planets. Using the simple volume of sphere formula, you can easily calculate the space inside any sphere. Whether you're curious about the volume of a solid sphere in math or science
8 min read
Surface Area of a Hemisphere
A hemisphere is a 3D shape that is half of a sphere's volume and surface area. The surface area of a hemisphere comprises both the curved region and the base area combined. Hemisphere's Total Surface Area (TSA) = Curved Surface Area + Base Area = 3πr² square units.Curved Surface Area (CSA) = 2πr² sq
13 min read
Volume of Hemisphere
Volume of a shape is defined as how much capacity a shape has or we can say how much material was required to form that shape. A hemisphere, derived from the Greek words "hemi" (meaning half) and "sphere," is simply half of a sphere. If you imagine slicing a perfectly round sphere into two equal hal
6 min read
Volume of Combination of Solids
When two or more two solids are combined and the combination comes out useful, a shape that can be found in reality is called a combination of solids. When Solids are taught, the major focus is always on the point of their real-life use and applications, For example, a cylinder can be seen in Pipes
9 min read
Frustum of Cone
Frustum of a cone is a special shape that is formed when we cut the cone with a plane parallel to its base. The cone is a three-dimensional shape having a circular base and a vertex. So the frustum of a cone is a solid volume that is formed by removing a part of the cone with a plane parallel to cir
10 min read
Conversion of solids - Surface Areas and Volumes
Conversions or changes are now a normal feature of our everyday lives. A goldsmith, for instance, melts a strip of gold to turn it into a gem. Likewise, a kid plays with clay forms it into various toys, a carpenter uses the wooden logs to shape various items/furniture for housekeeping. Likewise, for
4 min read
Surface Areas and Volumes
Surface Area and Volume are two fundamental properties of a three-dimensional (3D) shape that help us understand and measure the space they occupy and their outer surfaces. Knowing how to determine surface area and volumes can be incredibly practical and handy in cases where you want to calculate th
10 min read
Chapter 14: Statistics
How to find Mean of grouped data by direct method?
Statistics involves gathering, organizing, analyzing, interpreting, and presenting data to form opinions and make decisions. Applications range from educators computing average student scores and government officials conducting censuses to demographic analysis. Understanding and utilizing statistica
9 min read
Shortcut Method for Arithmetic Mean
Statistics, in layman's words, is the process of gathering, classifying, examining, interpreting, and finally presenting information in an understandable manner so that one can form an opinion and, if necessary, take action. Examples: A teacher collects students' grades, organizes them in ascending
6 min read
How to Calculate Mean using Step Deviation Method?
Step Deviation Method is a simplified way to calculate the mean of a grouped frequency distribution, especially when the class intervals are uniform. In simple words, statistics implies the process of gathering, sorting, examining, interpreting and then understandably presenting the data to enable o
7 min read
Graphical determination of Median
A measure of central tendency that determines the centrally located value of a given series is known as the Median. The number of values of the series below and above the given series is always equal. To determine the median value of a given series, it is first managed in increasing or decreasing or
5 min read
Ogive (Cumulative Frequency Curve) and its Types
A method of presenting data in the form of graphs that provides a quick and easier way to understand the trends of the given set of data is known as Graphic Presentation. The two types of graphs through which a given set of data can be presented are Frequency Distribution Graphs and Time Series Grap
6 min read
Chapter 15: Probability
Types of Events in Probability
Whenever an experiment is performed whose outcomes cannot be predicted with certainty, it is called a random experiment. In such cases, we can only measure which of the events is more likely or less likely to happen. This likelihood of events is measured in terms of probability and events refer to t
13 min read
Dependent and Independent Events
Dependent and Independent Events are the types of events that occur in probability. Suppose we have two events say Event A and Event B then if Event A and Event B are dependent events then the occurrence of one event is dependent on the occurrence of other events if they are independent events then
8 min read
Experimental Probability
Experimental probability, also known as empirical probability, is a concept in mathematics that deals with estimating the likelihood of an event occurring based on actual experimental results. Unlike theoretical probability, which predicts outcomes based on known possibilities, experimental probabil
8 min read
Probability Theory
Probability theory is an advanced branch of mathematics that deals with measuring the likelihood of events occurring. It provides tools to analyze situations involving uncertainty and helps in determining how likely certain outcomes are. This theory uses the concepts of random variables, sample spac
10 min read
Experimental Probability
Experimental probability, also known as empirical probability, is a concept in mathematics that deals with estimating the likelihood of an event occurring based on actual experimental results. Unlike theoretical probability, which predicts outcomes based on known possibilities, experimental probabil
8 min read
CBSE Class 10th Maths Formulas: Chapter Wise Formula and Points
Mathematics is one of the most scoring subject in CBSE Class 10th board exam. So Students are advised to prepare well for Math in order to score good marks in CBSE Class 10 board exam. GeeksforGeeks has curated the chapter wise Math formulae for CBSE Class 10th exam. These Formulae include chapters
15+ min read
NCERT Solutions for Class 10 Maths 2024-25: Chapter Wise PDF Download
NCERT Solutions for Class 10 Maths are tailored by subject matter experts to assist 10 students in securing top marks in their 10 board exams. As we know mathematics is the highest-scoring subject in the CBSE Class 10th board exam This complete resource includes all questions and answers from the CB
15+ min read
RD Sharma Class 10 Solutions
RD Sharma Class 10 Solutions offer excellent reference material for students, enabling them to develop a firm understanding of the concepts covered. in each chapter of the textbook. As Class 10 mathematics is categorized into various crucial topics such as Algebra, Geometry, and Trigonometry, which
9 min read