Volume of a Cylinder| Formula, Definition and Examples
Last Updated :
30 Sep, 2024
Volume of a cylinder is a fundamental concept in geometry and plays a crucial role in various real-life applications. It is a measure which signifies the amount of material the cylinder can carry. It is also defined as the space occupied by the Cylinder. The formula for the volume of a cylinder is πr2h where r is the radius of the base and h is the height of the cylinder.
In this article, we will explain the formula, highlight its applications, and provide examples to help you master how to calculate the volume of a cylinder efficiently for real-world use.
What is a Cylinder?
Cylinder is defined as a 3-D figure in which the base are circle and both surfaces are connected by a curved surface.
Cylinder is a three-dimensional geometric shape with two parallel and congruent circular bases connected by a curved surface. It has a fixed height, which is the perpendicular distance between the two circular bases, and a radius, which measures the distance from the center to the edge of each base.
For example, Gas cylinders and Rolling Pin in our houses resemble cylindrical shapes.
Volume of Cylinder
Volume of a cylinder is a crucial mathematical concept used to determine the space a cylindrical object occupies. It can also define the total capacity of any cylinder, i.e. the total amount of liquid any cylinder can hold. It is generally measured in liters, volume can also be measured in m3, cm3, etc. Volume of Cylinder is calculated by multiplying area of circular base (πr2) of cylinder by its height (h).

Volume of Cylinder
Volume of Cylinder is equal to πr2h. Where, r is the radius of radius of the base and h is the height of the cylinder.
V Cylinder = πr2h
Volume of Solid Cylinder
A Solid cylinder is a three-dimensional geometric cylinder with the space inside the cylinder completely filled. Unlike a hollow cylinder, where the interior has empty space, a solid cylinder has no empty space inside, meaning it is completely made up of material throughout its volume.
Volume of a Solid cylinder is also equal to the amount of space taken by it in a three-dimensional space. In other words, it determines the space or region enclosed by the cylinder is its volume, and the unit of volume is cubic unit i.e., the number of unit cubes (cubes of unit length) that may fit into an object.
Volume of Solid Cylinder : V Solid Cylinder = πr2h
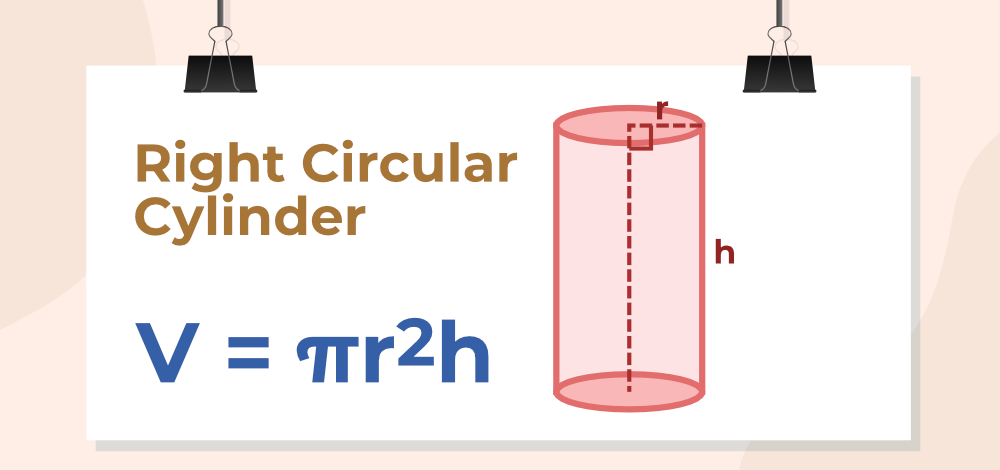
Volume of a Right Circular Cylinder
In a Right Circular Cylinder angle between the plane of the base or top and the curved surface is a right angle. For a right circular cylinder, the base is a circle with radius r, thus its area is πr2, and the height of the cylinder is h then the volume of the cylinder is:
Volume of Right Circular Cylinder : V Right Circular Cylinder = πr2h
Where,
- r = radius of the base
- h= height of the cylinder
Volume of Right Circular Cylinder
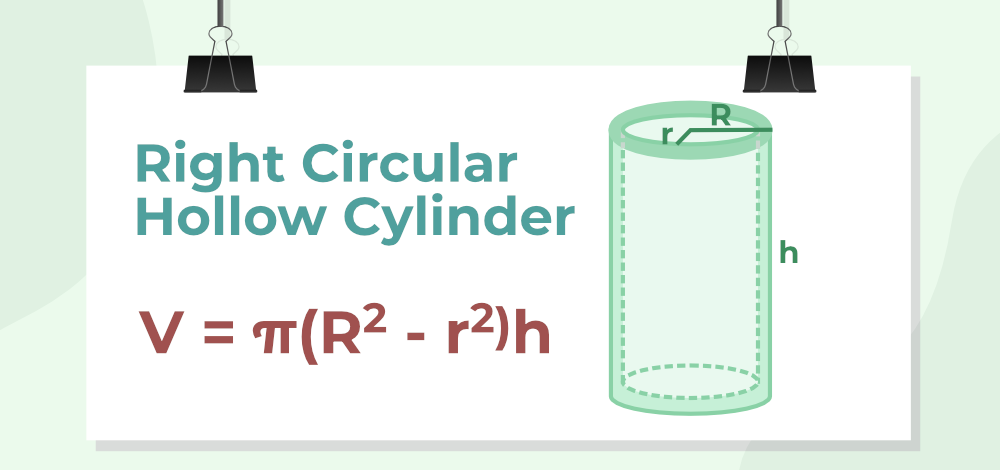
Volume of a Hollow Cylinder
A hollow cylinder is a three-dimensional geometric shape that consists of two concentric cylindrical surfaces, where the space between the outer and inner surfaces is filled with material, but the interior part is hollow. thus, its bases form a ring with two radii, inner radius and an outer radius. In simple words a hollow cylinder is a cylinder, which is empty from the inside and has some difference between the internal and external radius. Suppose a hollow cylinder is taken with its inner radius as r and outer radius as R and the height of the cylinder is h then volume of hollow cylinder is given as:
Volume of Solid Cylinder : V Hollow Cylinder = π( R2 – r2)h
Where,
- R = Outer Radius
- r = inner radius
- h= height of the cylinder
Volume of a Hollow Cylinder
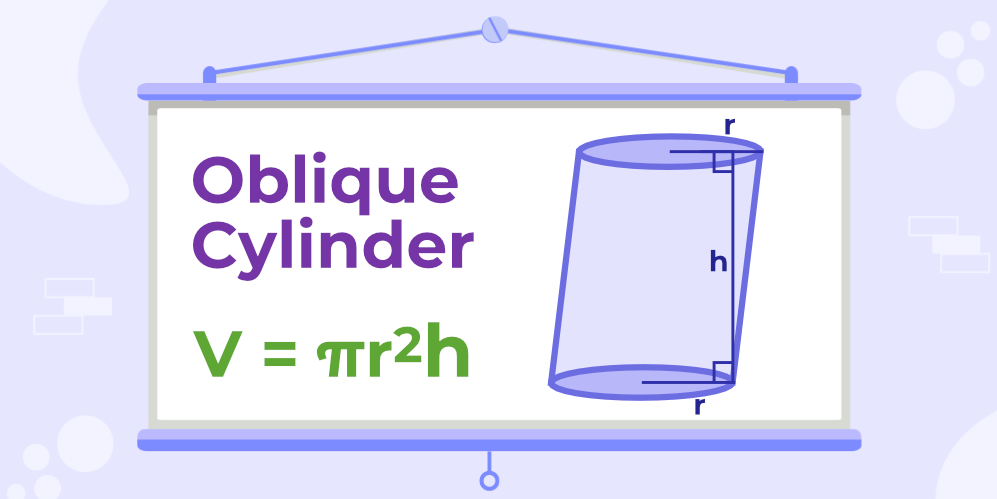
Volume of an Oblique Cylinder
An oblique cylinder is a type of cylinder where the sides are slanted, meaning the axis that connects the centers of the two circular bases is not perpendicular to the bases. In contrast to a right cylinder, where the axis is at a 90-degree angle to the bases, an oblique cylinder appears tilted.
In an Oblique Cylinder angle between the plane of the base or top and the curved surface is not a right angle.
But volume of oblique cylinder is given by the same formula as a product of the area of the base and the height of the cylinder.
Volume of Oblique Cylinder : V Oblique Cylinder = πr2h
Where,
- r = radius of the base
- h= height of the cylinder
Volume of Oblique Cylinder
Volume of an Elliptic Cylinder
An Elliptic cylinder is a three-dimensional geometric shape similar to a regular cylinder but with elliptical (oval-shaped) bases instead of circular ones. In an elliptic cylinder, the cross-section along its length is an ellipse, and the sides are perpendicular to the bases.
Volume of Elliptic Cylinder : V Elliptic Cylinder = πabh
Where,
- a = semi major axis of ellipse
- b = semi minor axis of ellipse
- h = height of the cylinder

Volume of Elliptic Cylinder
Volume of Cylinder in Liters
Generally, the volume of a cylinder is calculated in cubic meters or cubic centimeters but we can change them in litres by using the conversion factor discussed below i.e.,
1 cm3 = 1 ml
1000 cm3 = 1 litre
1 m3 = 1000000 cm3 = 1000 litres
Example: If a cylinder has a volume of 32 m3 it can be written as 32×1000 litres = 32000 litres.
Some formulas for cylinder other than volume formulas are,
Surface Area of a Cylinder
|
|
CSA ( Curved Surface Area)
|
Area of the surface except the base and top
|
CSA of Cylinder = 2πrh
|
|
TSA ( Total Surface Area)
|
Area of the complete surface of the cylinder
|
TSA of Cylinder = 2πr(r+h)
|
Volume of a Cylinder
|
|
Volume of a Cylinder
|
πr2h ( r= radius of base, h= height of cylinder)
|
|
Volume of Hollow Cylinder
|
π( R2 – r2)h ( r= inner radius, R= outer radius, h= height of cylinder)
|
|
Volume of Oblique Cylinder
|
πr2h ( r= radius of base, h= height of cylinder)
|
|
Volume of Elliptic Cylinder
|
πa b h ( a = semi major axis of ellipse, b = semi minor axis of ellipse, h= height of cylinder)
|
Read More
Examples Questions on Volume of Cylinder
Example 1: Calculate the volume of a cylinder of radius 3 m and a height of 4 m. (take π = 3.14)
Solution:
We have, r = 3 and h = 4
Using the formula we have,
V = πr2h
⇒ V = 3.14 × (3)2 × 4
⇒ V = 113.04 m3
Example 2: Calculate the volume of a cylinder of radius 4 m and a height of 7 m.
Solution:
We have, r = 4 and h = 7
Using the formula we have,
V = πr2h
⇒ V = 3.14 × (4)2 × 7
⇒ V = 351.68 m3
Example 3: Calculate the radius of a cylinder if its volume is 300 m3 and height is 7 m.
Solution:
We have, V = 300 and h = 7
Using the formula we have,
V = πr2h
⇒ r2 = V/πh
⇒ r2 = 300/(3.14 × 7)
⇒ r = 3.68 m
Example 4: Calculate the radius of a cylinder if its volume is 450 m3 and its height is 9 m.
Solution:
We have, V = 450 and h = 9
Using the formula we have,
V = πr2h
⇒ r2 = V/πh
⇒ r2 = 450/(3.14 × 9)
⇒ r = 12.52 m
Example 5: Calculate the height of a cylinder if its volume is 570 m3 and its radius is 4 m.
Solution:
We have, V = 570 and r = 4
Using the formula we have,
V = πr2h
⇒ h = V/πr2
⇒ h = 570/(3.14 × 4 × 4)
⇒ h = 11.34 m
Example 6: Calculate the height of a cylinder if its volume is 341 m3 and its radius is 6 m.
Solution:
We have,
V = 341 m3
r = 6 m
Using the formula we have,
V = πr2h
⇒ h = V/πr2
⇒ h = 341/(3.14 × 6 × 6)
⇒ h = 3.01 m
Practice Questions on Volume of Cylinder
Q1: Find Volume of Cylinder whose diameter is 14 cm and height is 12 cm.
Q2: Find Volume of Cylinder whose surface area of base is 84 cm2 and height is 11 cm.
Q3: Find the height of cylinder whose radius is 7 cm and volume is 770 cm3
Q4: Find the volume of a hollow cylinder of height 13 cm whose inner radius is 6 cm and outer radius is 1 cm.
Q5: A hollow cylindrical tube has an outer radius of 10 cm, an inner radius of 8 cm, and a height of 30 cm. Calculate the volume of the material used to make the tube.
Q6: Find the volume of an elliptic cylinder with a semi-major axis of 6 cm, a semi-minor axis of 4 cm, and a height of 10 cm.
Q7: The volume of an elliptic cylinder is 3,000 cm³, and the semi-major axis is 10 cm, while the height is 15 cm. Find the semi-minor axis.
Q8: Calculate the volume of an oblique cylinder whose base has a diameter of 14 cm and a perpendicular height of 12 cm.
Q9: The volume of an oblique cylinder is 5,000 m³, and the radius of its base is 20 m. What is the perpendicular height of the cylinder?
Q10: Find the volume of a hollow elliptic cylinder with an outer semi-major axis of 10 cm, an inner semi-major axis of 8 cm, and a height of 12 cm. The semi-minor axes are 6 cm and 4 cm, respectively.
|
Answers to Practice Questions
|
|
1. 1848.83 cm³
|
2. 923.02 cm³
|
3. 5 cm
|
4. 1429.97 cm³
|
5. 3393.05 cm³
|
|
6. 753.98 cm³
|
7. 6.37 cm
|
8. 1848.83 cm³
|
9. 3.98 m
|
10. 1055.58 cm³
|
Conclusion
Volume of a cylinder is essential for various real-world applications, from calculating the capacity of containers and tanks to solving problems in engineering and architecture. The formula V=πr2h provides a straightforward method to determine how much space a cylinder occupies, whether it’s a standard, hollow, or even an oblique or elliptic cylinder.
Learning this concept not only enhances your problem-solving skills but also offers practical benefits in everyday scenarios where accurate volume measurements are necessary.
Volume of Cylinder – FAQs
What is Volume of a Cylinder?
Volume of a cylinder is defined as the capacity of the cylinder, i.e. the amount of substance a cylinder can hold. It can also be defined as the total material required for making a cylinder.
Volume of the cylinder (V) is given by the formula, V = (Area of Circular Base) × (Height) Or V = πr2h
What is Volume of a Cylinder if its Radius is Doubled?
As the volume of the circle is proportional to the square of the radius. So if the radius of the circle is doubled then its volume becomes four times.
What is Volume of Cylinder if its Radius is halved?
As the volume of the circle is proportional to the square of the radius. So if the radius of the circle is halved then its volume becomes one by four times.
What is unit of Volume of a Cylinder?
Volume of a cylinder is measured in cubic units, i.e. cubic centimeters (cm3), cubic meters (m3), cubic feet (ft3) and so on for mathematical purposes. In general usage, it is also measured in Litters (l), milliliters (ml), etc.
What is Volume of a Hollow Cylinder?
If R is the external radius and r is the internal radius, then the formula for calculating the cylinder’s volume is given by: V = π (R2 – r2) h cubic units.
What is Volume of an Oblique Cylinder?
Formula to find the volume of an oblique cylinder is same as the volume of the cylinder and the volume of the oblique cylinder is calculated using the formula. V = π r2 h cubic units.
What is Volume of an Elliptical Cylinder?
Formula to find the volume of an Elliptical Cylinder is, V = πabh cubic units. Where, a and b are the radii of bases of the cylinder and h is the height of the cylinder.
What is Volume of a Solid Cylinder?
Volume of Solid Cylinder is the volume of material used in making the cylinder. For any solid cylinder of height ‘h’ and radius ‘r’ its volume is given by the formula, V = πr2h.
Similar Reads
Mensuration in Maths | Formulas for 2D and 3D Shapes, Examples
Mensuration is a branch of mathematics concerned with the calculation of geometric figures and their parameters such as weight, volume, form, surface area, lateral surface area, and so on. Let's learn about all the mensuration formulas in maths. Mensuration MeaningMensuration is the branch of mathem
6 min read
Introduction to Mensuration
Geometric Shapes in Maths
Geometric shapes are mathematical figures that represent the forms of objects in the real world. These shapes have defined boundaries, angles, and surfaces, and are fundamental to understanding geometry. Geometric shapes can be categorized into two main types based on their dimensions: 2D Shapes (Tw
2 min read
Visualizing Solid Shapes
Visualizing Solid Shapes: Any plane or any shape has two measurements length and width, which is why it is called a two-dimensional(2D) object. Circles, squares, triangles, rectangles, trapeziums, etc. are 2-D shapes. If an object has length, width, and breadth then it is a three-dimensional object(
8 min read
Volume of Combination of Solids
When two or more two solids are combined and the combination comes out useful, a shape that can be found in reality is called a combination of solids. When Solids are taught, the major focus is always on the point of their real-life use and applications, For example, a cylinder can be seen in Pipes
9 min read
Mensuration Formulas
Mensuration is the branch of geometry that deals with the measurement of area, length, or volume in 2D and 3D shapes. The 2D and 3D shapes are often called geometric shapes. In this article, we have curated all the mensuration formulas for various 2-D and 3-D shapes in detail. Mensuration Definition
12 min read
Perimeter
Perimeter of Triangle
The perimeter of a triangle is the total length of its three sides. A triangle is a polygon with three sides, three vertices, and three angles. It is the simplest closed polygon in geometry, as it is the first possible closed figure. Any polygon can be divided into triangles. For instance, a quadril
5 min read
How to find the perimeter of an equilateral triangle?
The perimeter of an equilateral triangle is equal to 3 x a, where a is the length of any sideAn Equilateral triangle is a triangle in which all three sides are equal and the angles are also equal. The value of each angle of an equilateral triangle is 60 degrees therefore, it is also known as an equi
6 min read
Perimeter of an Isosceles Triangle
A triangle can be considered as an isosceles triangle if and only if two sides of the triangle have the same length and two equal angles. The perimeter of an isosceles triangle comes under the parent topic mensuration which is a branch of geometry that deals with measurements of 2D/3D figures. Perim
5 min read
Perimeter of Rectangle
A rectangle is a two-dimensional plane quadrilateral, with opposite sides equal and all four angles equal. The perimeter of a rectangle can be defined as the sum of the length of all four sides in a rectangle. In this article, we are going to learn how to find the perimeter of rectangles using formu
7 min read
Perimeter of Square | Formula, Derivation, Examples
A square is a four-sided polygon (quadrilateral) with the following properties. All sides are of equal length, and each angle is a right angle (90°). It is a type of rectangle where the length and width are the same.A square also has the property that its diagonals are equal in length and bisect eac
4 min read
Perimeter of a Parallelogram
The perimeter of a parallelogram is the sum of the length of its boundaries/sides. A parallelogram is a type of quadrilateral with four equal sides with opposite sides equal. Its sides do not intersect each other. There are two diagonals of a parallelogram that intersect each other at the center. A
9 min read
Perimeter of A Rhombus
Perimeter of a Rhombus is the sum of all the sides of the rhombus. In a rhombus, all the sides are equal so the perimeter of the rhombus is 4 times its side. The perimeter of Rhombus is calculated by the formula P = 4a where a is the side length of the perimeter. Now we can also find the perimeter o
6 min read
How to Find the Perimeter of a Trapezium?
Suppose the sides of a Trapezium are a, b, c, and d then the Perimeter of the Trapezium is (a + b + c + d) units.Trapezium is a quadrilateral in which one pair of opposite sides are parallel. The perimeter of the trapezium is the sum of the boundaries of the trapezium. Suppose the sides of the trape
12 min read
Circumference of Circle - Definition, Perimeter Formula, and Examples
The circumference of a circle is the distance around its boundary, much like the perimeter of any other shape. It is a key concept in geometry, particularly when dealing with circles in real-world applications such as measuring the distance traveled by wheels or calculating the boundary of round obj
8 min read
Area
Area of a Triangle | Formula and Examples
The area of the triangle is a basic geometric concept that calculates the measure of the space enclosed by the three sides of the triangle. The formulas to find the area of a triangle include the base-height formula, Heron's formula, and trigonometric methods. The area of triangle is generally calcu
6 min read
Area of Equilateral Triangle
The area of an equilateral triangle is the amount of space enclosed within its three equal sides. For an equilateral triangle, where all three sides and all three internal angles are equal (each angle measuring 60 degrees), the area can be calculated using the formula [Tex]\frac{\sqrt{3}}{4}\times a
6 min read
Right Angled Triangle | Properties and Formula
Right Angle Triangle is a type of triangle that has one angle measuring exactly 90 degrees or right angle (90°). It is also known as the right triangle. In a right triangle, the two shorter sides called the perpendicular and the base, meet at the right angle (90°), while the longest side, opposite t
7 min read
Heron's Formula
Heron's formula is a popular method for calculating the area of a triangle when the lengths of its three sides are known. It was introduced by Heron of Alexandria in his book "Metrica". This formula applies to all types of triangles, including right-angled, equilateral, and isosceles. According to t
9 min read
Area of Square
Area of a Square is defined as the space enclosed by the boundary of the square. Measurement of the area is done in square units. The unit for measurement of the area is m2. Let's understand the Area of square with the following illustration: To calculate square's area we need to know the length of
9 min read
Area of Rectangle
Area of the Rectangle is the region covered inside the boundaries of the rectangle. The area of a rectangle is calculated using its dimensions length and breadth similar to the square in which the side is both the length and breadth. To find the area, you multiply the length of the rectangle by its
10 min read
Area of Parallelogram | Definition, Formulas & Examples
The area of a Parallelogram is the space or the region enclosed by the boundary of the parallelogram in a two-dimensional space. It is calculated by multiplying the base of the parallelogram by its height. In this article, we will learn more about the Area of Parallelogram Formulas, and how to use t
10 min read
Area of Rhombus: Formula, Derivation and Examples
Rhombus is a parallelogram in which all four sides are equal and opposite pairs of lines are congruent. The opposite angles in a rhombus are equal. The area of Rhombus is the total space occupied by a Rhombus in a 2d Plane. It is a special type of parallelogram in which all sides are equal to each o
9 min read
Area of Trapezoid Formula
Area of a trapezoid is a concept in geometry that helps to calculate the space enclosed by the unique quadrilateral. Basically it is measured in square units. In this article we will discuss in detail how to find the area of a trapezoid. Before going to the formula of the area of the trapezoid let's
9 min read
Area of a Circle: Formula, Derivation, Examples
Area of a Circle is the measure of the two-dimensional space occupied by a circle. It is mostly calculated by the size of the circle's radius which is the distance from the center of the circle to any point on its edge. The area of a circle is proportional to the radius of the circle. Area of Circle
10 min read
How to Calculate Area of Sector of Circle?
Area of the sector is easily calculated by using various formulas of geometry. In this article, we have covered a definition of sector circles, types of sectors, and others in detail. Table of Content Sector DefinitionTypes of SectorsFormula for Area of a SectorExamples on Area of Sector of CircleFA
5 min read
Segment of a Circle
Segment of a Circle is one of the important parts of the circle other than the sector. As we know, the circle is a 2-D shape in which points are equidistant from the point and the line connecting the two points lying on the circumference of the circle is called the chord of the circle. The area form
7 min read
Find the area of the shaded region formed by the intersection of four semicircles in a square
Given the length of the side of a square a, the task is to find the area of the shaded region formed by the intersection of four semicircles in a square as shown in the image below: Examples: Input: a = 10 Output: 57Input: a = 19 Output: 205.77 Approach: Area of the shaded region will be: Area(semic
4 min read
3D Shape
Volume of a Cube
Volume of a Cube is defined as the total number of cubic units occupied by the cube completely. A cube is a three-dimensional solid figure, having 6 square faces. Volume is nothing but the total space occupied by an object. An object with a larger volume would occupy more space. The volume of the cu
9 min read
Diagonal of a Cube Formula
Diagonal of a cube is the line segment joining the two non-adjacent vertices of a Cube. The diagonal of a cube formula helps us to calculate the length of diagonals in a cube. There are primarily two diagonals in a cube, namely face diagonals and body diagonals. In this article, we will learn the ty
8 min read
Volume of Cuboid | Formula and Examples
Volume of a cuboid is calculated using the formula V = L × B × H, where V represents the volume in cubic units, L stands for length, B for breadth, and H for height. Here, the breadth and width of a cuboid are the same things. The volume signifies the amount of space occupied by the cuboid in three
8 min read
Volume of a Sphere
The volume of a sphere helps us understand how much space a perfectly round object occupies, from tiny balls to large planets. Using the simple volume of sphere formula, you can easily calculate the space inside any sphere. Whether you're curious about the volume of a solid sphere in math or science
8 min read
Volume of Hemisphere
Volume of a shape is defined as how much capacity a shape has or we can say how much material was required to form that shape. A hemisphere, derived from the Greek words "hemi" (meaning half) and "sphere," is simply half of a sphere. If you imagine slicing a perfectly round sphere into two equal hal
6 min read
Volume of Cone- Formula, Derivation and Examples
Volume of a cone can be defined as the space occupied by the cone. As we know, a cone is a three-dimensional geometric shape with a circular base and a single apex (vertex). Let's learn about Volume of Cone in detail, including its Formula, Examples, and the Frustum of Cone. Volume of ConeA cone's v
10 min read
Volume of a Cylinder| Formula, Definition and Examples
Volume of a cylinder is a fundamental concept in geometry and plays a crucial role in various real-life applications. It is a measure which signifies the amount of material the cylinder can carry. It is also defined as the space occupied by the Cylinder. The formula for the volume of a cylinder is π
11 min read
Mensuration 3D - Hollow sphere
In this article we shall calculate the volume, Curved surface area (CSA) and Total surface area of a hollow sphere or a spherical shell. Below shown is a diagram of a hollow sphere. As we can see in the figure, the outer radius of hollow sphere is ‘R’ and the inner radius is ‘r’. Volume of hollow sp
2 min read
Volume of a Hollow Cylinder
A cylinder is a three-dimensional object that is formed when a rectangle is rotated along any of its sides. A hollow cylinder is one type of cylinder that is hollow from the inside. A hollow cylinder can be defined as a three-dimensional geometric object that is empty from the inside. A hollow cylin
8 min read
Convert Cubic Meter To Liter (m³ to l)
Cubic meter and Liter are the two most common metric units of volume. A mathematical quantity that is used to measure the amount of three-dimensional space that is occupied is called volume. It is usually measured numerically by using SI-derived units (like the cubic meter and liter) or various impe
6 min read
Total Surface Area
Surface Area of Cube | Curved & Total Surface Area
Surface area of a cube is defined as the total area covered by all the faces of a cube. In geometry, the cube is a fascinating three-dimensional object that we encounter daily, from dice to ice cubes. But have you ever wondered about the total area that covers a cube? This is what we call the surfac
15 min read
Surface Area of Cuboid
The surface area of a cuboid is the total space occupied by all its surfaces/sides. In geometry, a three-dimensional shape having six rectangular faces is called a cuboid. A cuboid is also known as a regular hexahedron and has six rectangular faces, eight vertices, and twelve edges with congruent, o
12 min read
Surface Area of Sphere | Formula, Derivation and Solved Examples
A sphere is a three-dimensional object with all points on its surface equidistant from its center, giving it a perfectly round shape. The surface area of a sphere is the total area that covers its outer surface. To calculate the surface area of a sphere with radius r, we use the formula: Surface Are
8 min read
Surface Area of a Hemisphere
A hemisphere is a 3D shape that is half of a sphere's volume and surface area. The surface area of a hemisphere comprises both the curved region and the base area combined. Hemisphere's Total Surface Area (TSA) = Curved Surface Area + Base Area = 3πr² square units.Curved Surface Area (CSA) = 2πr² sq
13 min read
Surface Area of Cone
Surface Area of a Cone is the total area encompassing the circular base and the curved surface of the cone. A cone has two types of surface areas. If the radius of the base is 'r' and the slant height is 'l', we use two formulas: Total Surface Area (TSA) of the cone = πr(r + l)Curved Surface Area (C
8 min read
Surface Area of Cylinder | Curved and Total Surface Area of Cylinder
Surface Area of a Cylinder is the amount of space covered by the flat surface of the cylinder's bases and the curved surface of the cylinder. The total surface area of the cylinder includes the area of the cylinder's two circular bases as well as the area of the curving surface. The volume of a cyli
10 min read
Lateral or Curved Surface Area