Spectrophotometric Determination of the pKa, Isosbestic Point and Equation of Absorbance vs. pH for a Universal pH Indicator ()
1. Introduction
The value of the acid dissociation constant (pKa) is an important parameter that indicates the degree of ionization of molecules in solution at different pH values. Many chemical, physical and biological properties of natural and synthetic compounds are governed by the interactions of acidic and basic groups. In such compounds, the pKa controls many aspects of metabolism and even transport across membranes; therefore, its study is of significant interest in biology, pharmaceutics, medicine, and numerous other scientific fields. Zscherp et al. [1] determinedthe transient pKa changes of a single amino acid side chain in bacteriorhodopsin, a protein important in photosynthesis. Baran et al. [2] investigated the pKa of amino acid complexes and their relationship to atomic orbitals. Several groups [3] - [6] have investigated the behaviors of different drugs, including those used against cancer, and the dependence of their behaviors on their pKa values. Novel pKa determination methods that use pH colorimetric indicators have also been reported [7] - [9] .
Two main methods exist for determining the pKa of a compound: potentiometric titration and spectrophotometric titration. The main advantage of the second method is the ability to obtain a titration curve, which allows for estimation at any point without necessitating an experiment. In contrast, potentiometric titration requires knowledge of the equilibrium concentrations of the reagents, which are not necessary in spectrophotometric titration because the ratio of the concentrations of the basic and acidic parts is obtained from the results of absorbance measurements. When the mixing of a solution and an indicator is investigated spectrophotometrically, the mixture absorbs in the UV region (250 - 380 nm), whereas the indicator, depending on the pH of the solution, absorbs in the visible region (380 - 700 nm). The pKa can be determined from the spectrophotometric data using different methods. Meloun et al. [10] [11] describe multiwavelength analysis and nonlinear least squares regression methods. Computational methods, such as SQUAD (84) 16 and SPECFIT/32, and some new methods [12] - [15] have also been developed.
Theoretical Foundations
When an acid HA is dissolved in water, equilibrium is established:
 (1)
(1)
The acid HA transfers a proton to water, and it becomes the anion A−. This anion tends to retrieve the proton and behave as a base; A− is therefore referred to as the “conjugate base” of acid HA, and HA and A− are referred to as a “conjugate pair” [16] [17] . A shift of the equilibrium in Equation (1) to the right or left depends on the relative strengths of the HA and H3O+ acids. The “strength” of an acid refers its tendency to transfer protons, and one method of standardizing its force is to compare the protonation state when it interacts with water. The result of this comparison is expressed as the “acid dissociation constant,” Ka, as follows:
 (2)
(2)
Ka is a constant of the stoichiometric equilibrium defined in terms of the concentration ratio [A−]/[HA], which can be determined spectrophotometrically [18] . If a solution with a total concentration of indicator CT becomes very acidic, all indicator exists as HA. The absorbance of the solution at a given wavelength l is given by
 (3)
(3)
where eHA is the molar absorptivity of HA at wavelength l and b is the width of the cell containing the solution. If the solution is too basic, the same concentration of indicator is converted entirely into A− and the absorbance at the same wavelength is given by
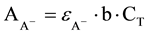 (4)
(4)
where  is the molar absorptivity of A−. At an intermediate pH, the absorbance is given by
is the molar absorptivity of A−. At an intermediate pH, the absorbance is given by
 (5)
(5)
where the total concentration is defined in any condition as
 (6)
(6)
For a given CT, Equations (3)-(6) can be combined to obtain
 (7)
(7)
This relationship must be evaluated at multiple wavelengths, including one where HA absorbs appreciably but A− does not, one where A− is much more absorbent than HA, and another where the absorbance of the two species is approximately the same. The pH (−log[H3O+]) of the solutions must be in the transition range of the indicator so that both HA and A-are present in appreciable concentrations. Ka can be evaluated graphically by converting Equation (2) to logarithmic form:
 (8)
(8)
In addition, the combination of Equation (7) and the definition of pKa = −log Ka results in
 (9)
(9)
Therefore, a graph of  vs. pH has an ideal slope of one. If this condition holds, the y-axis intercept gives the negative pKa directly. However, the experimental slope is not usually exactly 1, necessitating that the pH values of the measurements be extrapolated to pH = 0 (which is typically a factor of 106 in concentration), resulting in a very large error for the pKa value. In contrast, if the least-squares equation is solved for the intercept with the x-axis, where
vs. pH has an ideal slope of one. If this condition holds, the y-axis intercept gives the negative pKa directly. However, the experimental slope is not usually exactly 1, necessitating that the pH values of the measurements be extrapolated to pH = 0 (which is typically a factor of 106 in concentration), resulting in a very large error for the pKa value. In contrast, if the least-squares equation is solved for the intercept with the x-axis, where , the pKa results are obtained with very little uncertainty. At this intercept, [A−]/[HA] = 1, indicating that the concentrations of the acid and its conjugate base are equal. When HA is a strong acid, a value for Ka in aqueous solutions cannot be defined, because HA molecules cannot be detected; the value of Ka is therefore very high or infinite. In contrast, a very low value indicates that the dissociation Ka involves a very small fraction of the total acid present. The isosbestic point is the point on the graph of absorbance vs. pH where the molar absorption coefficients of the species in equilibrium are the same.
, the pKa results are obtained with very little uncertainty. At this intercept, [A−]/[HA] = 1, indicating that the concentrations of the acid and its conjugate base are equal. When HA is a strong acid, a value for Ka in aqueous solutions cannot be defined, because HA molecules cannot be detected; the value of Ka is therefore very high or infinite. In contrast, a very low value indicates that the dissociation Ka involves a very small fraction of the total acid present. The isosbestic point is the point on the graph of absorbance vs. pH where the molar absorption coefficients of the species in equilibrium are the same.
2. Experimental Methods
Preparation of solutions. Three families of buffers covering the pH range between 2.3 and 10.85 were prepared using four solutions of citrate buffer at pH 2.3, 3.05, 4.37 and 5.4 containing citric acid and its conjugate base. Table 1 shows the reactants and the proportions used to prepare the citrate buffer.
The calculation model is based on the Henderson-Hasselbach Equation (10) and on the final concentration Cf of the acid and its conjugate base (Equation (4)):
 (10)
(10)
 (11)
(11)
To calculate the mass of the reagents, Equations (10) and (11) are solved simultaneously by setting the desired pH and concentration (Cf). These equations were solved using a program developed in LabVIEW for greater agility. The results for the formulations are shown in Table 2.
The calculated pH values exhibited some differences, especially at higher pH levels, as illustrated in Figure 1. The standard deviation of the data was 0.728 pH units.
A second phosphate buffer was prepared using two stock solutions: 100 ml of 0.2 M monosodium phosphate and disodium phosphate (Table 3), which were mixed to obtain buffer solutions with pH 6.5, 7.08 and 7.7.
The preparation of stock solutions required 2.84 g of monosodium phosphate (monohydrate) and 2.76 g of disodium phosphate, which were then diluted with distilled-and-deionized water to a volume of 100 ml. Finally, these stock solutions were combined in the proportions listed in Table 4.
![]()
Table 1. Reagents used in the preparation of the citrate buffer.
![]()
Table 2. Preparation of the citrate buffer at different pH levels and concentrations.
![]()
Table 3. Reagents used to prepare the phosphate buffers.
![]()
Table 4. Preparation of phosphate buffer at different concentrations and pH.
![]()
Figure 1. Comparison between the calculated and obtained pH values for citrate buffers.
Figure 2 shows the curves of the calculated and obtained pH values for the prepared phosphate buffers. The obtained pH values were higher than expected, with a standard deviation of 0.434 pH units.
The third set of solutions consisted of ammonium buffers composed of a mixture of two stock solutions: ammonia and ammonium chloride. The calculations were similar to those used for the preparation of the phosphate buffers. Table 5 shows the details of the preparation.
Figure 3 shows the curves for the calculated and experimental pH values for the ammonium buffer solutions. The obtained values were higher than expected, with a standard deviation of 0.624 pH units.
Even though the buffers did not have pH values equal to the calculated values, they were very stable and retained their constant pH value for months. The most important factor for the spectrophotometric determination of pKa is that the pH be known and stable.
3. Results and Discussion
Spectrophotometric measurements. Absorbance measurements were performed using a Thermo Scientific Evolution 300 UV-Vis spectrophotometer in the wavelength range between 380 and 700 nm (visible spectrum). In this study, universal indicator pH 1 - 11 (catalog number 45712, Carlo Ebra, Milano, Italy) was used. The pH measurements were performed using an Oakton pH 2700 pH meter. Plots of absorbance against l for the universal indicator in buffers with different pH levels are illustrated in Figure 4.
Neither the chemical composition of this indicator nor its dissociation constant was provided by the manufacturer. Therefore, spectrophotometric measurements were performed to determine its pKa, and two graphical and two analytical methods were applied. The results obtained using the different methods were compared, and the
![]()
Figure 2. Comparison of the calculated and measured pH values for the phosphate buffers.
![]()
Table 5. Preparation of ammonium buffer at different concentrations and pH.
![]()
Figure 3. Comparison of the measured and calculated pH values for ammonium buffers.
![]()
Figure 4. Absorbance spectra of the universal indicator pH 1 - 11 at different pH levels.
most suitable pKa value was determined. The first method was to take the spectra of the species with extreme pHlevels (pH = 2.3 and pH = 10.85 in this case) and determine the wavelengths of maximum absorbance, as illustrated in Figure 5.
As illustrated in Figure 5, the absorbance spectrum of the solution of pH = 2.3 exhibited a peak at 434 nm; the peak of the basic solution occurred at 600 nm. The plot of the absorbance vs. pH at these wavelengths is presented in Figure 6.
The pKa was obtained by determining the pH of the point of intersection of the two linear curves as shown in Figure 6. To determine this point, the linear equations of the two points closest to the crossing at each curve were solved:
![]() (12)
(12)
where
![]() (13)
(13)
Thus,
pKa = 8.0792
The second method was to plot ![]() vs. pH (Equation (9)), where AHA is the ab-
vs. pH (Equation (9)), where AHA is the ab-
![]()
Figure 5. Absorbance spectra of universal indicator solutions at extreme pH levels.
![]()
Figure 6. Plot of absorbance vs. pHat wavelengths of 434 and 600 nm.
sorbance of the acid solution, Ai is each of the intermediate absorbances and AA is the absorbance of the basic solution. For the best results, the wavelength where the difference between the absorbance curves of the acidic and basic solutions is greatest must be chosen, which, in this case, corresponds to l = 600 nm.
When linear regression of the data is performed, the resulting equation is
![]() (14)
(14)
A graph of the data and the linear regression are shown in Figure 7.
The pKa is obtained from the intercept of the line with the x-axis according to the equation
![]() (15)
(15)
In addition, ![]()
We have
![]()
Figure 7. Linear regression of log[(AHA − Ai)/(Ai − AA)] vs. pH.
![]() (16)
(16)
resulting in ![]()
The third method is to use Equation (8) solved for pKa:
![]() (17)
(17)
where pHi is the intermediate value between the acid and alkaline extreme values. The intermediate pH selected was pH = 7.7, which was chosen because of the substantial coincidence of its isosbestic point with those of the extreme-pH solutions, as illustrated in Figure 8.
Equation (16) was then applied to all wavelengths, resulting in the graph shown in Figure 9.
The average of all of the data gives pKa = 8.26272. The isosbestic point was determined by taking the wavelength value where the standard deviation of absorbance was minimal. The isosbestic point appeared at a wavelength of 494 nm.
The fourth method, which is similar to the third, was based on the absorbance of three solutions with different pH values (i.e., a very acidic, a very basic and one intermediate-pH solution) at the same wavelength. The pKa was obtained by substituting these values into the following equation:
![]() (18)
(18)
where I represents the lowest pH value (in this case, 2.3); a corresponds to the difference between the intermediate pH and the I value (a = 7.7 − 2.3 = 5.4); b is the difference between the most alkaline pH and the most acidic (b = 10.85 − 2.3 = 8.55); A1, A2 and A3 are the absorbances at each pH value for a given wavelength. The calculated pKa data are plotted as a function of wavelength in Figure 10.
The average of all of the data gave pKa = 8.26466, which is very similar to that determined using the third method. Table 6 shows a summary of the results obtained using the four methods.
The average value obtained using the four methods correspond to a pKa of 8.277. The results obtained using these equations were similar, with a standard deviation of only 0.00137; thus, the average of these two values was taken as the most appropriate result for the pKa of the indicator in this report:
![]()
Finally, an equation for the relationship between the absorbance and the pH at a wavelength of 600 nm was obtained. This wavelength was selected because it is the value where the absorbance is highest and produces the greatest rate of change in Equations (17) and (18). The absorbance as a function of pH was obtained via non-
![]()
Figure 8. Absorbance curves for the acidic, basic, and intermediate pH solutions.
![]()
Figure 9. Curve of values obtained from Equation (17).
![]()
Figure 10. Graph of pKa vs. l for pKa values calculated using Equation (18).
linear curve fitting in OriginProusing the Gaussian equation, which provided the best fit to the data. Figure 11 shows the data and the curve of best fit.
The data from the best-fit equation are shown in Table 7.
Therefore, the equation can be written as
![]()
Table 6. Comparison of the pKa data obtained using the different methods.
![]()
Table 7. Equation modeling of absorbance as a function of pH.
![]()
Figure 11. Equation for the absorbance vs. pH at λ = 600 nm.
![]() (19)
(19)
4. Conclusion
The application of spectrophotometric titration allowed the acid dissociation constant of universal pH indicator 1 - 11 from Carlo Ebra to be determined. Four methods of analysis―two graphical and two mathematical methods―were used and produced results with good similarity, especially in the cases of the two mathematical methods. Some colorimetric pH indicators, such as bromothymol blue in acidic solutions, are unstable over long periods of time, whereas the studied universal indicator retains its color even months after being exposed to highly acidic solutions. This property and the significant color variation make the development of a technique for spectrophotometric measurement of pH interesting. An equation was obtained for the relationship between absorbance and pH with a very low dispersion, and the isosbestic point was determined.