1. Introduction
The quantum Hall effect is derived by the total Hamiltonian  of a many-electron system which is composed of the single electron Hamiltonian
of a many-electron system which is composed of the single electron Hamiltonian  of the i-th electron and the Coulomb interaction between electrons as follows:
of the i-th electron and the Coulomb interaction between electrons as follows:
 (1)
(1)
 (2)
(2)
where , e,
, e,  and N are the effective mass, the elementary charge, the momentum and the total number of electrons. Therein
and N are the effective mass, the elementary charge, the momentum and the total number of electrons. Therein 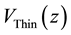 indicates the potential of the z-direction which confines the electrons to an ultra- thin conducting layer. Also
indicates the potential of the z-direction which confines the electrons to an ultra- thin conducting layer. Also  is the electric potential along the Hall voltage (y-direction). The vector potential,
is the electric potential along the Hall voltage (y-direction). The vector potential,  , has the components,
, has the components,
 (3)
(3)
where B is the strength of the magnetic field. The last term of Equation (2) indicates the Zeeman energy where  is the effective g-factor,
is the effective g-factor,  is the Bohr magneton
is the Bohr magneton  and
and  is the z-component of the i-th electron spin operator. The details have been explained in the previous papers [1] -[14] . When the Coulomb interaction between electrons is ignored in the quasi-2D electron system, the Hamiltonian of the single electron is exactly diagonalized same as in the Landau solution. At a filling factor
is the z-component of the i-th electron spin operator. The details have been explained in the previous papers [1] -[14] . When the Coulomb interaction between electrons is ignored in the quasi-2D electron system, the Hamiltonian of the single electron is exactly diagonalized same as in the Landau solution. At a filling factor , all the electrons are placed in the Landau orbitals with the Landau level number
, all the electrons are placed in the Landau orbitals with the Landau level number . The residual Landau orbitals (
. The residual Landau orbitals (![]() ) are empty. So there are various electron-configurations in the Landau orbitals. We divide the total Hamiltonian
) are empty. So there are various electron-configurations in the Landau orbitals. We divide the total Hamiltonian ![]() into the diagonal part
into the diagonal part ![]() and the non-diagonal part
and the non-diagonal part ![]() as follows;
as follows;
![]()
We define two symbols W and C which are the expectation values of ![]() and the Coulomb energy, respectively. We call C “classical Coulomb energy” (the expectation value of the Coulomb interaction). Then the classical Coulomb energy becomes a minimum for only one electron configuration in the Landau orbitals. This property has been proven in the previous paper [9] . That is to say W becomes a minimum at the only one electron configuration. The electron configuration gives the single ground state for each value of
and the Coulomb energy, respectively. We call C “classical Coulomb energy” (the expectation value of the Coulomb interaction). Then the classical Coulomb energy becomes a minimum for only one electron configuration in the Landau orbitals. This property has been proven in the previous paper [9] . That is to say W becomes a minimum at the only one electron configuration. The electron configuration gives the single ground state for each value of![]() . The residual Coulomb interaction HI (non diagonal part of HT) produces many quantum transitions from the ground state. We have examined the perturbation energy via these quantum transitions in details in the previous papers [1] -[14] .
. The residual Coulomb interaction HI (non diagonal part of HT) produces many quantum transitions from the ground state. We have examined the perturbation energy via these quantum transitions in details in the previous papers [1] -[14] .
Then all the electron pairs placed in the nearest Landau orbitals can transfer to all the empty orbitals at the specific filling factors![]() . When the filling factor
. When the filling factor ![]() deviates from the fractional numbers
deviates from the fractional numbers ![]() the number of the transitions abruptly decreases. The reason comes from the combined effects of the momentum conservation along the x direction (current direction), the most uniform electron configuration and the Pauli exclusion-principle. The abrupt decreasing of the transition number yields the valley structure in the perturbation energy. That is to say the energy
the number of the transitions abruptly decreases. The reason comes from the combined effects of the momentum conservation along the x direction (current direction), the most uniform electron configuration and the Pauli exclusion-principle. The abrupt decreasing of the transition number yields the valley structure in the perturbation energy. That is to say the energy ![]() of the nearest electron pair takes a minimum at
of the nearest electron pair takes a minimum at ![]() and the energy
and the energy
![]() for
for ![]() is higher than
is higher than![]() . Then
. Then ![]() gives the energy gap (valley depth) as proven in the previous papers [9] [12] [13] .
gives the energy gap (valley depth) as proven in the previous papers [9] [12] [13] .
The total energy of the quantum Hall system is the sum of W (expectation value of![]() ) and all the pair energy of electrons (placed in nearest orbital pairs and more distant orbital pairs), because the Coulomb interaction works between two electrons. We will study the function form of the expectation value W in the next section. Then we get the energy spectrum of the quantum Hall system which is quite different from the Halperin result [15] . The valley depth in the pair energy and the function form of W give the quantum Hall plateaus at the specific filling factors
) and all the pair energy of electrons (placed in nearest orbital pairs and more distant orbital pairs), because the Coulomb interaction works between two electrons. We will study the function form of the expectation value W in the next section. Then we get the energy spectrum of the quantum Hall system which is quite different from the Halperin result [15] . The valley depth in the pair energy and the function form of W give the quantum Hall plateaus at the specific filling factors![]() . The theoretical results are in good agreement with the experimental data.
. The theoretical results are in good agreement with the experimental data.
2. Expectation Value of the Total Hamiltonian and Its n-Dependence
We describe the expectation value of the total Hamiltonian ![]() by the symbol W which is the sum of the single electron energies and the expectation value of the classical Coulomb energy as follows;
by the symbol W which is the sum of the single electron energies and the expectation value of the classical Coulomb energy as follows;
![]() (4)
(4)
where ![]() is the single electron energy of the i-th electron and
is the single electron energy of the i-th electron and ![]() is the expectation value of the Coulomb interaction between electrons. The total number of Landau states with
is the expectation value of the Coulomb interaction between electrons. The total number of Landau states with ![]() is equal to
is equal to ![]() where
where ![]() and d are the length and the width of a quantum Hall device respectively. The total charge of electrons at the filling factor
and d are the length and the width of a quantum Hall device respectively. The total charge of electrons at the filling factor ![]() is the product of
is the product of ![]() and the total number of Landau states. The total charge is divided by the area
and the total number of Landau states. The total charge is divided by the area![]() , and then we obtain the charge density as
, and then we obtain the charge density as
![]() (5)
(5)
Figure 1 shows one of the experimental data [16] . The upper figure indicates the Hall resistance ![]() divided by the Klitzing constant
divided by the Klitzing constant![]() . The value of
. The value of ![]() is equal to
is equal to ![]() which is almost proportional to the magnetic field strength B except the Hall plateau regions. This property is easily seen by comparing the data with the red line. That is to say,
which is almost proportional to the magnetic field strength B except the Hall plateau regions. This property is easily seen by comparing the data with the red line. That is to say, ![]() is nearly equal to the constant value. Equation (5) means the charge density
is nearly equal to the constant value. Equation (5) means the charge density ![]() to be proportional to
to be proportional to![]() . Accordingly the macroscopic Coulomb energy
. Accordingly the macroscopic Coulomb energy ![]() may be treated to be a constant value in the experiment of Figure 1.
may be treated to be a constant value in the experiment of Figure 1.
We next examine the microscopic charge-distribution in more details. Figure 2 shows the electron configuration with a minimum classical-Coulomb-energy at![]() . Therein the bold lines express the occupied orbitals with electron and the dashed lines indicate the empty orbitals. The electron pair located at the orbitals AB is one example of the nearest-electron-pairs. Two electrons placed at B and C show the second nearest pair. The electron pair at A and C is the third nearest pair. The pair at A and D is the fourth nearest pair and so on. The classical Coulomb energy between two electrons is expressed by the symbols
. Therein the bold lines express the occupied orbitals with electron and the dashed lines indicate the empty orbitals. The electron pair located at the orbitals AB is one example of the nearest-electron-pairs. Two electrons placed at B and C show the second nearest pair. The electron pair at A and C is the third nearest pair. The pair at A and D is the fourth nearest pair and so on. The classical Coulomb energy between two electrons is expressed by the symbols ![]() and
and ![]() respectively as in Figure 2. Therein
respectively as in Figure 2. Therein ![]() is the largest one of the classical Coulomb pair energy,
is the largest one of the classical Coulomb pair energy, ![]() is the second largest,
is the second largest, ![]() is the third largest,
is the third largest, ![]() is the fourth largest and so on.
is the fourth largest and so on.
The classical Coulomb energy between the pair (A, C) is weakened by the screening (shielding) effect of electron B. Also the classical Coulomb energy between the pair (A, D) is weakened by the screening effect of electrons B and C. Accordingly the n-dependence of the classical Coulomb energy mainly comes from the first nearest and the second nearest pairs. The number of the more distant pairs (third, fourth, fifth and so on) are enormous many. The total number of electron pairs is![]() . On the other hand the total number of the first and second nearest pairs is N. Accordingly the residual energies (namely the sum of all the more distant pair
. On the other hand the total number of the first and second nearest pairs is N. Accordingly the residual energies (namely the sum of all the more distant pair
![]()
Figure 1. Hall resistance RH and diagonal resistance R in ultrahigh- mobility device in [16] .
![]()
Figure 2. Electron configuration with the minimum classical Coulomb energy at![]() .
.
energies) may be approximated by the macroscopic Coulomb energy ![]() which is a constant value mentioned above. On the other hand the sum of the first and second nearest pair-energies is strongly dependent upon the filling factor. The n-dependence is examined for various filling factors as follows:
which is a constant value mentioned above. On the other hand the sum of the first and second nearest pair-energies is strongly dependent upon the filling factor. The n-dependence is examined for various filling factors as follows:
(Case of![]() )
)
We can ignore the boundary effect in both ends for a macroscopic electron-number N. Then the number of the first nearest pairs is equal to ![]() and the number of the second nearest pairs is equal to
and the number of the second nearest pairs is equal to ![]() for the configuration of Figure 2. Then the sum of the classical Coulomb energies between the first nearest pairs is equal to
for the configuration of Figure 2. Then the sum of the classical Coulomb energies between the first nearest pairs is equal to ![]() and that between the second nearest pairs is equal to
and that between the second nearest pairs is equal to![]() . Accordingly, the total classical Coulomb energy
. Accordingly, the total classical Coulomb energy ![]() is approximated by
is approximated by
![]() (6)
(6)
Next we estimate the classical Coulomb energy for n = 3/5, 4/7, 5/7 where the electron-configurations with the minimum classical Coulomb energy are shown in Figures 3-5.
(Case of![]() ) The number of the first nearest pairs is equal to
) The number of the first nearest pairs is equal to ![]() and the number of the second nearest pairs is equal to
and the number of the second nearest pairs is equal to ![]() at
at ![]() as seen in Figure 3. Then the total classical Coulomb energy is nearly equal to
as seen in Figure 3. Then the total classical Coulomb energy is nearly equal to
![]() (7)
(7)
(Case of![]() ) Figure 4 shows that the number of the first nearest pairs is equal to
) Figure 4 shows that the number of the first nearest pairs is equal to ![]() and the number of the second nearest pairs is equal to
and the number of the second nearest pairs is equal to ![]() at
at![]() . The total classical Coulomb energy is given by
. The total classical Coulomb energy is given by
![]() (8)
(8)
(Case of![]() ) The number of the first nearest pairs is equal to
) The number of the first nearest pairs is equal to ![]() and the number of the second nearest pairs is equal to
and the number of the second nearest pairs is equal to ![]() at
at ![]() as easily seen in Figure 5. Then the total classical Coulomb energy is
as easily seen in Figure 5. Then the total classical Coulomb energy is
![]() (9)
(9)
(Any case of![]() )
)
We calculate the classical Coulomb energy for a general case of ![]()
![]() . As it is proven in Ref. [9] the electron-configuration with the minimum classical Coulomb energy is constructed by repeating the representative unit-configuration where r electrons exist in sequential q Landau orbitals. The number of empty orbitals per unit-configuration is
. As it is proven in Ref. [9] the electron-configuration with the minimum classical Coulomb energy is constructed by repeating the representative unit-configuration where r electrons exist in sequential q Landau orbitals. The number of empty orbitals per unit-configuration is![]() . All the empty orbitals are separated by one or more filled-orbitals at
. All the empty orbitals are separated by one or more filled-orbitals at![]() . That is to say, all the empty orbitals are isolated as seen in Figures 2-5. Therefore the
. That is to say, all the empty orbitals are isolated as seen in Figures 2-5. Therefore the ![]() second-nearest pairs exist per unit-configuration due to the presence of the
second-nearest pairs exist per unit-configuration due to the presence of the ![]() empty orbitals. The total numbers of the first and the second nearest pairs is equal to the total number of electrons as easily seen in Figures 2-5. Therefore the number of nearest pairs becomes
empty orbitals. The total numbers of the first and the second nearest pairs is equal to the total number of electrons as easily seen in Figures 2-5. Therefore the number of nearest pairs becomes ![]() per unit-configuration. The total number of nearest electron pairs is equal to
per unit-configuration. The total number of nearest electron pairs is equal to ![]() and the total number of second nearest electron pairs is equal to
and the total number of second nearest electron pairs is equal to ![]() for the filling factor
for the filling factor![]() . Consequently the total classical Coulomb energy is given by
. Consequently the total classical Coulomb energy is given by
![]()
Figure 3. Electron configuration with the minimum classical Coulomb energy at![]() .
.
![]()
Figure 4. Electron configuration with the minimum classical Coulomb energy at![]() .
.
![]()
Figure 5. Electron configuration with the minimum classical Coulomb energy at![]() .
.
![]()
This equation is expressed by using ![]() as
as
![]() (10)
(10)
From Equations (4) and (10) the expectation value of the total Hamiltonian is obtained as
![]() (11)
(11)
The single electron eigenenergy ![]() has been investigated in the previous papers and the result is the following form.
has been investigated in the previous papers and the result is the following form.
![]() (12)
(12)
where ![]() expresses the ground state energy along the z direction (direction of the thickness in the thin conducting electron channel). Also
expresses the ground state energy along the z direction (direction of the thickness in the thin conducting electron channel). Also ![]() is the potential along the y direction (Hall voltage direction). Substitution of Equation (12) into Equation (11) yields the expectation value of the total Hamiltonian as follows:
is the potential along the y direction (Hall voltage direction). Substitution of Equation (12) into Equation (11) yields the expectation value of the total Hamiltonian as follows:
![]() (13a)
(13a)
We put together the constant parts as follows;
![]() (13b)
(13b)
Therein f is the constant value as
![]() (14a)
(14a)
![]() (14b)
(14b)
where ![]() is the mean value of the potential along the y-direction. Equation (13b) gives the function-form of W, which is illustrated in Figure 6.
is the mean value of the potential along the y-direction. Equation (13b) gives the function-form of W, which is illustrated in Figure 6.
The function W depends linearly upon ![]() and the proportional coefficient
and the proportional coefficient ![]() is negative, because the classical Coulomb energy between the first nearest electron pair,
is negative, because the classical Coulomb energy between the first nearest electron pair, ![]() , is larger than that between the second nearest pair,
, is larger than that between the second nearest pair,![]() . Thus the expectation value of the total Hamiltonian W changes continuously with
. Thus the expectation value of the total Hamiltonian W changes continuously with ![]() as in Figure 6. Accordingly the classical Coulomb energy has no energy-gap and so cannot produce the plateaus of Hall resistance. The confinement of the Hall resistance comes from another reason as studied in the previous papers [6] [9] [12] [13] . The allowed transitions of electron-pairs decrease abruptly when the filling factor
as in Figure 6. Accordingly the classical Coulomb energy has no energy-gap and so cannot produce the plateaus of Hall resistance. The confinement of the Hall resistance comes from another reason as studied in the previous papers [6] [9] [12] [13] . The allowed transitions of electron-pairs decrease abruptly when the filling factor ![]() deviates from the specific filling factor
deviates from the specific filling factor![]() . This structure is named “valley structure”. Summation of the valley energy and W gives the energy spectrum of the quasi 2D-electron system as examined in the next section.
. This structure is named “valley structure”. Summation of the valley energy and W gives the energy spectrum of the quasi 2D-electron system as examined in the next section.
3. n-Dependence of the Total Energy
We already calculated the energy of electron pairs placed in the nearest orbitals by employing the perturbation calculation in the previous papers [1] - [13] . The exact