Tax Evasion Dynamics via Non-Equilibrium Model on Directed Small-World Networks ()
Received 26 July 2016; accepted 23 August 2016; published 26 August 2016

1. Introduction
Over the past three decades, the Ising model (IM) was used successfully in the study of biological, social, behavioral, opinion dynamic and economic systems [1] . This model, also, has been applied to study social and economic behavior of a people community close to its critical points [1] - [9] . The tax evasion on the community of people is a difficult problem for governments (see [10] - [13] ). Following R. Wintrobe and K. Gërxhani [14] , higher levels of tax evasion occur in less developed countries because people have less trust in governmental institutions. Empirical evidence indicates that taxpayers make their decisions on tax evasion considering the opinion of taxpayers who are members of your group or neighborhood [15] [16] . In this context, Zaklan et al. [7] [8] developed an economics model to study the problem of tax evasion dynamics on a people community. However, this model deals with illegal tax evasion only. These studies were used the equilibrium IM on a square lattice (SL) and Monte-Carlo simulations with the Glauber algorithms as a dynamic of temporal evolution.
Zaklan et al. [8] considered a large number of agents (people) who interact locally with their neighbors and base their decision whether to evade taxes or not on the behavior of their neighbors. They used the IM on a SL in the study of tax evasion. Furthermore, a tax enforcement mechanism is presented in this model. The enforcement mechanism consists of two components: probability ( ) of an audit and punishment length (k) of time. Once evaders are detected, these two mechanisms are applied to tax evaders to become honest. Their results are in excellent agreement with analytical and experimental results obtained by [14] .
) of an audit and punishment length (k) of time. Once evaders are detected, these two mechanisms are applied to tax evaders to become honest. Their results are in excellent agreement with analytical and experimental results obtained by [14] .
Lima [17] proposed non-equilibrium model where each agent or person did have an opinion in the presence of a social noise (q) as in the original Majority-Vote Model (MVM) [18] [19] . This model is based on the knowledge that people do not live in a social equilibrium, and any rumor or gossip can lead to a government or market chaos. Then, the MVM explains better events of non-equilibrium and makes this model more realistic to explain the social and economic behavior of a community of people.
Many social and economic networks in the real world exhibit directed relations. This may be modeled by including directed links in the corresponding complex network model. The motivation of this work is to study tax evasion on directed SW networks (DSW) via a non-equilibrium dynamics model (SLR) with the objective to make this model as realistic as possible.
In this work, we study the behavior of the tax evasion on an agent community of honest citizens and tax evaders, where the agents are positioned on sites of DSW networks, but now using a non-equilibrium SLR model proposed by Sánchez, López and Rodríguez (SLR) [20] . Our simulations aim to see how a model community of tax payers and tax cheaters (Zaklan model) [7] [8] behaves on these SLR networks.
2. Small-World (SW) Networks
Small-world (SW) networks have typical distance between two randomly selected nodes which increases only logarithmically with increasing number of nodes. SW networks are intermediate between the regular local networks and the random networks. They have two interesting features as high clustering which is the characteristic of regular networks, and the other is short path length that is characteristic of random networks. The combination these two features suggests that SW networks can be used to describe the behavior of various real systems that present interactions between nodes, agents or people as social and economic systems. Here we briefly describe the DSW and undirected SW (USW) networks used in this study as previously mentioned.
・ DSW networks
In the SW networks, we can introduce an asymmetric disorder, in such a way that we redirect a fraction p of the links. This redirecting results in a directed network, preserving the outgoing node of the redirected link but changing the incoming node, i.e., when A is tied to B, B may not be linked to A but to someone else instead. When  we have the SL in two-dimension, while for
we have the SL in two-dimension, while for 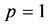 we have something similar to random networks [21] .
we have something similar to random networks [21] .
・ USW networks
In the USW networks, different from DSW networks, there exists a reciprocity or symmetry of redirected links, i.e., if node A selects node B as incoming neighbor then A is also an incoming neighbor of B. The neighbor relations were such that if A has B as a neighbor; B has A as a neighbor.
3. Zaklan Model via Non-Equilibrium Dynamics of SLR
The original Zaklan model [7] consists of some homogeneous agents or people located on the regular structure like a square lattice. In every time period each network site (node) is inhabited by an agent or people, spin , who can either be an honest taxpayer
, who can either be an honest taxpayer  or a cheater
or a cheater . In this model is assumed that initially everybody is honest. In each period, individuals can rethink their behavior and have the opportunity of becoming the opposite individual that they were in the previous period. The network neighborhood of every agent is composed of z people, agents to network nodes. Each agent’s social network may either prefer tax evasion or reject it. Individual decision making depends on two factors: First, the type of network every agent is connected with exerts influence on what type of citizen she becomes in the respective period. Second, people’s decisions are partly autonomous, i.e., they are not only influenced by the constitution of their neighborhood. The autonomous part of individual decision-making is responsible for the emergence of the tax evasion problem because some initially honest taxpayers decide to evade taxes and then exert influence on others to do so as well. Applied to tax evasion we can interpret the model as follows: tax evaders have the greatest influence to turn honest citizens into tax evaders if they constitute a majority in the respective neighborhood. If the majority evades, one is likely also to evade. On the other hand, if most people in the vicinity are honest, the respective individual is likely to become a taxpayer if she was a tax evader before. When the tax evasion is detected this model also presents an enforcement mechanism that consists of two components: a probability of an efficient audit
. In this model is assumed that initially everybody is honest. In each period, individuals can rethink their behavior and have the opportunity of becoming the opposite individual that they were in the previous period. The network neighborhood of every agent is composed of z people, agents to network nodes. Each agent’s social network may either prefer tax evasion or reject it. Individual decision making depends on two factors: First, the type of network every agent is connected with exerts influence on what type of citizen she becomes in the respective period. Second, people’s decisions are partly autonomous, i.e., they are not only influenced by the constitution of their neighborhood. The autonomous part of individual decision-making is responsible for the emergence of the tax evasion problem because some initially honest taxpayers decide to evade taxes and then exert influence on others to do so as well. Applied to tax evasion we can interpret the model as follows: tax evaders have the greatest influence to turn honest citizens into tax evaders if they constitute a majority in the respective neighborhood. If the majority evades, one is likely also to evade. On the other hand, if most people in the vicinity are honest, the respective individual is likely to become a taxpayer if she was a tax evader before. When the tax evasion is detected this model also presents an enforcement mechanism that consists of two components: a probability of an efficient audit  and punishment represented by a number k of time periods for the individual becoming honest. One time unit is one sweep through the entire system. The temporal evolution of this model can be performed by using an equilibrium or non-equilibrium dynamics. In the SLR on SW network, the system dynamics traditionally is as follows. We assign a spin variable
and punishment represented by a number k of time periods for the individual becoming honest. One time unit is one sweep through the entire system. The temporal evolution of this model can be performed by using an equilibrium or non-equilibrium dynamics. In the SLR on SW network, the system dynamics traditionally is as follows. We assign a spin variable  with values
with values  to each node of the network. At each step, we try to spin flip a node. The flip is accepted with some probability explained below. Here, we consider a simple non-equilibrium spin- like SLR model on directed SW networks. We put spin variables
to each node of the network. At each step, we try to spin flip a node. The flip is accepted with some probability explained below. Here, we consider a simple non-equilibrium spin- like SLR model on directed SW networks. We put spin variables  taking the values
taking the values  and situated on every site i of a directed SW network with
and situated on every site i of a directed SW network with  sites, where L is the side of the square lattice. In this network proposed by Sánchez et al. [20] , we start from a two-dimensional square lattice consisting of sites linked to their
sites, where L is the side of the square lattice. In this network proposed by Sánchez et al. [20] , we start from a two-dimensional square lattice consisting of sites linked to their  nearest neighbors by both outgoing and incoming links. Then, with rewiring probability p, we replace the nearest neighbor outgoing links by new outgoing links to different sites chosen at random, leaving the incoming links unchanged. After repeating this process for every link, we are left with a network with a density p of SW directed links. Therefore, with this procedure, every site will have
nearest neighbors by both outgoing and incoming links. Then, with rewiring probability p, we replace the nearest neighbor outgoing links by new outgoing links to different sites chosen at random, leaving the incoming links unchanged. After repeating this process for every link, we are left with a network with a density p of SW directed links. Therefore, with this procedure, every site will have  outgoing links and a varying (random) number of incoming links as shown in Figure 1 (left). Then, the spins (people, economic agents) are placed at the network sites. Thus, any agent is connected by l outgoing links to other agents or mates and can be in one of two possible
outgoing links and a varying (random) number of incoming links as shown in Figure 1 (left). Then, the spins (people, economic agents) are placed at the network sites. Thus, any agent is connected by l outgoing links to other agents or mates and can be in one of two possible  states taking the values
states taking the values![]() . Depending on the state of its mates in the neighborhood, an agent may change its state according to a majority rule (ferromagnetic). To implement this, we introduce the payoff function:
. Depending on the state of its mates in the neighborhood, an agent may change its state according to a majority rule (ferromagnetic). To implement this, we introduce the payoff function:
![]() (1)
(1)
where the sum is carried out over the l mates of agent i. The external noise or social temperature T is included allowing some degree of randomness in the time evolution. Then, for a given value of the external social tem- perature, the update of the model is performed as follows: at each step, an agent (network site) is randomly chosen and its corresponding ![]() is computed according to Equation (1).
is computed according to Equation (1).
・ 1): If![]() ,
, ![]() , the agent i opposes the neighborhood majority of its mates and the change of its actual state is accepted,
, the agent i opposes the neighborhood majority of its mates and the change of its actual state is accepted,
・ 2): If![]() , the change of its actual state is accepted with the probability
, the change of its actual state is accepted with the probability
![]() (2)
(2)
which depends on temperature, i.e., an unfavorable change. Therefore, this model is a non-equilibrium model, since detailed balance is not satisfied, due to the directedness of the links.
![]()
Figure 1. The left we show a DSW network in two-dimensions where the dotted lines represent rewired links [20] . The right, we show two types of phase transition. For rewiring probabilities ![]() (black line) the SLR model presents a continuous phase transition and a discontinuous phase transition for
(black line) the SLR model presents a continuous phase transition and a discontinuous phase transition for ![]() (red line).
(red line).
Phase transition theory distinguishes between first-order or discontinuous transitions and second-order or continuous transitions. For first-order transitions, the quantity of main interest, like the density of liquids in equilibrium with their vapour at fixed pressure, jumps discontinuously at some boiling temperature if the liquid is heated. Ferromagnets if heated show a second-order transition: at a sharp Curie temperature their magneti- sation goes to zero continuously though with infinite slope. Tricritical points in more complicated systems separate continuous transitions, at one side of the tricritical point, from discontinuous transitions at the other side. For our DSW networks, the tricritical point lies at a rewiring probability ![]() with a continuous transition for
with a continuous transition for ![]() and a discontinuous transition for
and a discontinuous transition for![]() . This difference is shown in the right part of Figure 1; due to the finiteness of our network size, as usual in simulations, the transitions are not infinitely sharp. At
. This difference is shown in the right part of Figure 1; due to the finiteness of our network size, as usual in simulations, the transitions are not infinitely sharp. At![]() : tricritical point, occurs a change in the phase transition form. It changes from second-order to first-order transition with increasing p. The simulations have been performed on different directed SW networks sizes comprising a number
: tricritical point, occurs a change in the phase transition form. It changes from second-order to first-order transition with increasing p. The simulations have been performed on different directed SW networks sizes comprising a number ![]() and 1600 of sites. The opinion of a people commu- nity per total number of people is given by
and 1600 of sites. The opinion of a people commu- nity per total number of people is given by
![]() . (3)
. (3)
In order to model tax evasion, we further use for all agents one probability of an efficient audit![]() . If tax evasion,
. If tax evasion, ![]() , is detected by this audit, the agent must remain honest,
, is detected by this audit, the agent must remain honest, ![]() , for a number k of time steps. Again, one time-step is one sweep through the entire network. In Figure 1 (right) we show two types of phase transitions due Equation (3). For rewiring probabilities
, for a number k of time steps. Again, one time-step is one sweep through the entire network. In Figure 1 (right) we show two types of phase transitions due Equation (3). For rewiring probabilities ![]() (black line) the SLR model presents a con- tinuous phase transition, while for
(black line) the SLR model presents a con- tinuous phase transition, while for ![]() (red line) we see a discontinuous phase transition. In the case
(red line) we see a discontinuous phase transition. In the case ![]() the opinion of the agents or people about some subject change smoothly, with growing temperature close to a critical point, from an organized state to another disorganized one with the growth of social tem- perature (T). Here, for organized state means that all agents are of the same opinion (honest or dishonest) and disorganized state means that half of the agents are honest and the other half are dishonest. Instead, for case
the opinion of the agents or people about some subject change smoothly, with growing temperature close to a critical point, from an organized state to another disorganized one with the growth of social tem- perature (T). Here, for organized state means that all agents are of the same opinion (honest or dishonest) and disorganized state means that half of the agents are honest and the other half are dishonest. Instead, for case ![]() the phase transition occurs abruptly, i.e. a jump from a organized to disorganized state near the critical temperature (
the phase transition occurs abruptly, i.e. a jump from a organized to disorganized state near the critical temperature (![]() ) of
) of![]() , i.e., the opinion of a people community jumps to other next to the
, i.e., the opinion of a people community jumps to other next to the![]() .
.
4. Controlling the Tax Evasion Dynamics
The fraction of tax evaders is
![]() (4)
(4)
where N is the total number and ![]() is the honest number of agents. The tax evasion is calculated at every time to step t of system evolution.
is the honest number of agents. The tax evasion is calculated at every time to step t of system evolution.
For SLR it is known that for![]() , half of the people are honest and the other half cheat, i.e., a jump occurs where all honest people flip for cheat (see Figure 1 for
, half of the people are honest and the other half cheat, i.e., a jump occurs where all honest people flip for cheat (see Figure 1 for![]() ). While for
). While for ![]() either one opinion or the other opinion dominates (see Figure 1 for
either one opinion or the other opinion dominates (see Figure 1 for![]() ). Because of this behavior we set a fixed noise (p) to some values slightly below
). Because of this behavior we set a fixed noise (p) to some values slightly below![]() , where the case that agents distribute in equal proportions onto the two alternatives is excluded. We set
, where the case that agents distribute in equal proportions onto the two alternatives is excluded. We set ![]() with
with ![]() (
(![]() ) and
) and ![]() (
(![]() ) such that we see flips of the whole system in the baseline cases
) such that we see flips of the whole system in the baseline cases ![]() and
and![]() . Then we vary the degrees of punishment (
. Then we vary the degrees of punishment (![]() , 10 and 50) and audit probability rate (
, 10 and 50) and audit probability rate (![]() , 10% and 90%). Therefore, if tax evasion is detected, the enforce- ment mechanism
, 10% and 90%). Therefore, if tax evasion is detected, the enforce- ment mechanism ![]() and the time of punishment k are triggered to control the tax evasion level. The punished individuals remain honest for a certain number k of periods, as explained before in section 3.
and the time of punishment k are triggered to control the tax evasion level. The punished individuals remain honest for a certain number k of periods, as explained before in section 3.
5. Results and Discussion
Here, we follow the same steps as we did in a previous work [17] . Therefore, we first will present the baseline case ![]() and
and![]() , i.e., no use of enforcement, at
, i.e., no use of enforcement, at ![]() and with
and with ![]() sites for
sites for ![]() (a) and 0.9 (c). All simulation for
(a) and 0.9 (c). All simulation for ![]() sites is performed over 25,000 time steps, as shown in Figure 2. For very low T the part of autonomous decisions almost completely disappears. The individuals then base their decision solely on what most of their neighbors do. A rising noise has the opposite effect. Individuals then decide more autonomously. Therefore, Figure 2 was expanded to two examples (Figure 2(b) and Figure 2(d)), to show how much the results change if one uses various random numbers, average on 40 different seeds. Error bars cannot describe this randomness properly (for the later figures, the error bars are visible from the fluctu- ations in time which show a band of fractions). Although everybody is honest initially, it is impossible to predict roughly which level of tax compliance will be reached at some time step in the future.
sites is performed over 25,000 time steps, as shown in Figure 2. For very low T the part of autonomous decisions almost completely disappears. The individuals then base their decision solely on what most of their neighbors do. A rising noise has the opposite effect. Individuals then decide more autonomously. Therefore, Figure 2 was expanded to two examples (Figure 2(b) and Figure 2(d)), to show how much the results change if one uses various random numbers, average on 40 different seeds. Error bars cannot describe this randomness properly (for the later figures, the error bars are visible from the fluctu- ations in time which show a band of fractions). Although everybody is honest initially, it is impossible to predict roughly which level of tax compliance will be reached at some time step in the future.
Figure 3 and Figure 4 illustrate different simulation settings for ![]() and
and ![]() on DSW networks, for each considered combination of degree of punishment (
on DSW networks, for each considered combination of degree of punishment (![]() , 10 and 50) and audit probability (
, 10 and 50) and audit probability (![]() , 10% and 90%), where the tax evasion is plotted over 25,000 time steps. Both a rise in audit probability (greater
, 10% and 90%), where the tax evasion is plotted over 25,000 time steps. Both a rise in audit probability (greater
![]() ) and a higher penalty (greater k) work to flatten the time series of tax evasion and to shift the band of possible non-compliance values towards more compliance. However, the simulations show that even extreme enforcement measures (
) and a higher penalty (greater k) work to flatten the time series of tax evasion and to shift the band of possible non-compliance values towards more compliance. However, the simulations show that even extreme enforcement measures (![]() and
and![]() ) cannot fully solve the problem of tax evasion.
) cannot fully solve the problem of tax evasion.
In Figure 5 and Figure 6, we plot tax evasion for ![]() and
and ![]() rewiring probabities, but now with
rewiring probabities, but now with ![]() and over 70,000 time steps, again for different enforcement k and audit probability
and over 70,000 time steps, again for different enforcement k and audit probability![]() . Now the fluctuations are much smaller since the network is nearly four times larger. For case Figure 5(a) and Figure 6(a)
. Now the fluctuations are much smaller since the network is nearly four times larger. For case Figure 5(a) and Figure 6(a)
![]()
Figure 4. The same as Figure 3, but now for![]() .
.
![]() and
and ![]() (c), we present the average over forty different seeds.
(c), we present the average over forty different seeds.
![]()
Figure 6. The same of the Figure 5, but now for![]() .
.
we plot the baseline case ![]() and
and![]() , i.e., no use of enforcement for
, i.e., no use of enforcement for ![]() and
and![]() . In these, we observed that the tax evasion fluctuates around 0.495 for
. In these, we observed that the tax evasion fluctuates around 0.495 for ![]() and 0.127 for
and 0.127 for![]() , respectively. The large reduction in the tax evasion for
, respectively. The large reduction in the tax evasion for ![]() is due to the SW network effect, because the SW network has long-range interaction and can influence agents community that others networks can not. These networks have in common the formation of small agents block that is unachievable. Then, for rewiring probability
is due to the SW network effect, because the SW network has long-range interaction and can influence agents community that others networks can not. These networks have in common the formation of small agents block that is unachievable. Then, for rewiring probability ![]() the rogue agents are more connected and influenced by honest agents. Cases 5(b) and 6(b) with
the rogue agents are more connected and influenced by honest agents. Cases 5(b) and 6(b) with ![]() show a strong reduction of tax evasion on DSW similar to the case 6(a). In case 5(c) and 6(c), we show the tax evasion level decreases, on DSW, for a more realistic set of possible values for the degree of punishment
show a strong reduction of tax evasion on DSW similar to the case 6(a). In case 5(c) and 6(c), we show the tax evasion level decreases, on DSW, for a more realistic set of possible values for the degree of punishment ![]() and audit probability
and audit probability ![]() [7] [14] . In case 5(d) and 6(d) we also show that the tax evasion level decreases much more for an extreme set of punishment
[7] [14] . In case 5(d) and 6(d) we also show that the tax evasion level decreases much more for an extreme set of punishment ![]() and audit probability
and audit probability ![]() [7] for both rewiring probabilities
[7] for both rewiring probabilities ![]() and
and![]() . Therefore, our model also works for large networks.
. Therefore, our model also works for large networks.
To understand statistical errors, in Figure 5 and Figure 6 we plot tax evasion for DSW networks with
![]() now for the case
now for the case ![]() and
and![]() . We found from 40 samples in part (c) that the tax evasion remains at around 12.5% (
. We found from 40 samples in part (c) that the tax evasion remains at around 12.5% (![]() ) and 8% (
) and 8% (![]() ), but with fluctuations in time larger than from sample to sample: the probable errors are much smaller than the fluctuations seen in part (c).
), but with fluctuations in time larger than from sample to sample: the probable errors are much smaller than the fluctuations seen in part (c).
6. Conclusions
In this work, we used the SLR model as a temporal evolution dynamics of the Zaklan model. This model explores the effect of a directed small-world topology. On DSW networks, the SLR model presents two types of phase transition dependent rewiring probability p. And ![]() and
and ![]() have continuous and discontinuous phase transition, respectively. Zaklan model of tax evasion can be evolved by dynamic equilibrium as the Ising modle, non-equilibrium Majority-vote and SLR model are also on various topologies. Many social and economic networks in the real world exhibit directed bounds or relations and as the model of Zaklan incorporates concepts from both sociophysics and econophysics, we argue here that DSW networks are a good framework for simulating this kind of model where citizens always make a prior consultation with their nearest neighbors before making any final decisions. Also here we found same in the baseline case (
have continuous and discontinuous phase transition, respectively. Zaklan model of tax evasion can be evolved by dynamic equilibrium as the Ising modle, non-equilibrium Majority-vote and SLR model are also on various topologies. Many social and economic networks in the real world exhibit directed bounds or relations and as the model of Zaklan incorporates concepts from both sociophysics and econophysics, we argue here that DSW networks are a good framework for simulating this kind of model where citizens always make a prior consultation with their nearest neighbors before making any final decisions. Also here we found same in the baseline case (![]() and
and![]() ) for
) for![]() , the tax evasion is enough diminished on a large people community. Here, we also found the plausible result that tax evasion is diminished by higher audit probability
, the tax evasion is enough diminished on a large people community. Here, we also found the plausible result that tax evasion is diminished by higher audit probability ![]() and stronger punishment k on DSW networks.
and stronger punishment k on DSW networks.
What is needed in the future are more quantitative empirical data how tax cheaters react to punishment. For example, some governments bought bank account data stolen from Swiss banks, and as a result, lots of people admitted to the tax authorities that they did not report the income from the investments on these account to them. But details on amount of income, amount of punishment, and behavior in later years are hidden behind tax secrecy.
Acknowledgements
The author thanks Dietrich Stauffer for many suggestions and fruitful discussions during the development of this work. He thanks CNPq and FUNCAP for financial support. This work also was supported by the system SGI Altix 1350, the computational park CENAPAD, UNICAMP-USP, SP-BRAZIL.