Evidences for a Unified Physics, in Full Accordance with the Newtonian Laws ()
1. Introduction
This paper is based on following assumptions:
I. Finite mass of the universe, (implying a finite value of the total gravitational potential U).
II. Light composed of longitudinal-extended elastic particles moving at speed c = u.
On above bases we obtain, among others, these following results:
a) The relation u= (−2U)1/2, where u is total escape speed, that is the escape speed from all the masses (in space); then we assumed c = u.
b) The measured constancy of c, under a constant potential U, (like on Earth), for every Observer.
c) Doppler effect (DE) equations for the light, slightly different from the relativistic ones.
d) Regarding the Harvard Tower Experiment, the compensating velocity, (to restore the resonance source-absorber), see Section 2.4, has same value but contrary direction with respect to the one predicted by the Relativity.
e) On Earth, at height h, (e.g. at GPS satellites level), it is shown that a source (of light) emits at a lower frequency, inducing atomic clocks to run faster.
f) High redshifts, related to far sources, depend on the increase of c (as well as the increase of the photons length λ) during the path of light toward the Earth, (where |Uo| ? |U→∞|).
In the 2nd part, we show the interaction between our particles and circling electrons; we revised the electron structure, assuming the electron charge as a point-particle, (facing the atom nucleus during the electron orbit), which turns out to be the impact point between photons and electron; some results:
g) On H atom there are only  electron circular orbits; along each of them, n is also the number of admitted photons. We show that 2n0/ne0 =α(cmr/2hRH)1/2 = 1 (exactly) with n0 the photons admitted frequency along the ground-state orbit r0 (different, because of our new atom structure, from the Bohr radius) and ne0 the frequency of the electron reduced mass mr along r0, with α the fine structure constant.
electron circular orbits; along each of them, n is also the number of admitted photons. We show that 2n0/ne0 =α(cmr/2hRH)1/2 = 1 (exactly) with n0 the photons admitted frequency along the ground-state orbit r0 (different, because of our new atom structure, from the Bohr radius) and ne0 the frequency of the electron reduced mass mr along r0, with α the fine structure constant.
h) On Photoelectric effect, the number of photons hitting the electron varies from nf to 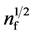 where nf is related to the specific threshold frequency nf (=Wf/h). For instance, it is shown that, as for Caesium (having Wf @ 2 eV), one gets nf = 361.
where nf is related to the specific threshold frequency nf (=Wf/h). For instance, it is shown that, as for Caesium (having Wf @ 2 eV), one gets nf = 361.
i) On Compton Effect, the number of photons admitted is one We point out that we got the Compton equation via our Doppler effect for the light, different from the relativistic Doppler Effect.
2. Part 1
2.1. Total Escape Speed
This argument has been widely treated on Section 2 of our previous paper [1] . Here we remember:
 (1)
(1)
escape speed of a particle m at a distance s from the mass M, with U the gravitational potential acting on m; if M is a real mass, s becomes the distance m-Cp with Cp the Centre of Potential of M, that is the point where |U| has the max value), while the escape velocity of m which has to be referred to Cp,
 (2)
(2)
may be called as absolute escape velocity of m, absolute as referred to Cp; then
 (3)
(3)
is the potential due to two masses; then the escape speed from two masses becomes
 (4)
(4)
yielding
 (5)
(5)
thus
 (6)
(6)
is the total escape speed, with U the total potential; u(absolute escape velocity)has to be referred to the centre of potential of all the masses, Cp. Now, if S is a Source emitting a particle m, we may call
 (7)
(7)
as relative escape velocity of m from S, (relative as u is referred to S). We assume now
 (8)
(8)
showing that c depends on U; on Earth, U is practically constant, see the final “Conclusions”.
Equation (8) is supported by a cosmological reason, as better explained between the Equation (5) and Equation (6) on [1] : in short, c > u implies the masses dispersion, c < u implies a gravitational collapse, where as c = u, appears to be the right speed of the light to avoid the two said events (collapse or dispersion).
2.2. Invariance of c for Every Observer, Under the Newtonian Laws
-This chapter is a deep revision/improvement of chapter4 of our previous paper [1] -
We assume now the light as composed of particular particles (photons), giving a Newtonian reason to the (apparent) constancy of c, defined as follows:
“Longitudinally-extended, elastic and massive particles having speed equal to the total escape speed u, and moving along rays, (continuous succession of photons)”.
(More photons emitted by a source, during an emission time T, need an equal number of rays).
Along each ray, every tail of a photon corresponds to the front of the next photon.
Now, on Figure 1, let S be a Source, (moving from an Observer O with velocity vOS), starting to emit, at t = 0, (when S is coincident with O), a photon (with front A), along the direction S-O; therefore
 (9)
(9)
represents, see Equation (7), at t = 0, the relative escape velocity of the front A from O, while the velocity of the front A, with respect to S, that is vSA, turns out to be
 (10)
(10)
![]()
Figure 1. The source S, in motion from the observer O, emits the photon AB.
Now, being ST be the position of S at t = T, we get
 (11)
(11)
where λO is the photon AB emitted with S in motion from O, whereas λ (ºuT) would be the photon AO if, during T, vOS= 0. Thus, at t =T, if the source is receding from the front A, as in Figure 1, the photon length λO, (for the Observer O), from Equation (11), becomes
 , (with
, (with ) (12)
) (12)
where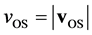 , with Δλ (=vOST) the path O-ST covered by S during T. For instance, if vOS = 0, the (12) gives
, with Δλ (=vOST) the path O-ST covered by S during T. For instance, if vOS = 0, the (12) gives  with λ the photon length as seen by S.
with λ the photon length as seen by S.
The Equation (12) shows that the length of a photon during its emission, given u and T, depends on vOS, meaning that Observers in reciprocal motion state different length for the same photon.
Now, referring to an Observer O, the speed of a photon, (since its length could vary), has to be defined considering its length λO referred to its transit time TO, leading to
![]() (13)
(13)
Returning to Figure 1, the transit time TO of the photon AB, for the Observer O, is given by the time the front A needs to cover the path λ, that is T (=λ/u), plus the time the tail B needs to cover the path ST − O = Δλ (=vOST) which is ΔT = vOST/u, giving
![]() (with
(with![]() ) (14)
) (14)
Thus, see Equation (13), referring to the Observer O, the speed of the photon AB becomes
![]() (15)
(15)
showing that c is invariant, under the Newtonian laws, in spite of any speed Source- Observer.
Anyhow, a photon, once emitted, has a constant length for every Observer, hence its speed has to vary for Observers in reciprocal motion, but we point out that the measurements of c via the method d/t implies that the light has to cross (or to be reflected by) an Observer O (taking the initial time); this means that O becomes the source S of photons emitted by a source at rest with O(vOS = 0) who will state a length λO = λ, a transit timeTO = T giving to c a constant measured value.
Along one ray, the frequency of photons, for an Observer O, is their number crossing O during a time t, that isn = n/t; thus for t = TO, (transit time of one photon, for an Observer O), from Equation (14),
![]() (16)
(16)
with the sign + when S and O, during the emission, are receding from each other. We point out that for β = 0, (vOS/u = 0), the photons frequency as stated by a source S (ns), has to be equal to the one stated by O (nO), which is also valid if O and S belong to different potential, (e.g., the equality of the number of balls falling from the top of a tower with respect to an Observer at the tower base), and this, for vOS = 0, always implies ns = nO. Figure 1, as well as Equations ((12) and (16)) represent the longitudinal Doppler effect for the light while, in general, this effect, with α the angle between the direction of S and OS, (and with S receding from O), see Figure 2, can be written as
![]() (with
(with![]() ) (17)
) (17)
![]()
Figure 4. Source circling around the observer O.
As for the Transverse Doppler effect, see Figure 3, in general, we have
![]() (18)
(18)
As for a source circling around an Observer O, see Figure 4, it is always λO > λ as follows:
![]() (19)
(19)
while their photons transit time is
![]() (20)
(20)
where r is the orbit radius, ω the angular speed, yielding, to every photon, the speed cO = c.
2.3. Physical Characteristics of These Photons
Also this argument has been widely treated on our previous paper [1] : here we point out that as the energy of light ![]() is valid for any mass, it has to be also valid for the light (massive on our bases) and therefore, writing
is valid for any mass, it has to be also valid for the light (massive on our bases) and therefore, writing ![]() we have to argue that each photon is provided with an internal energy
we have to argue that each photon is provided with an internal energy ![]() equal to its kinetic energy Kc. Toward the infinity, (where c∞ → 0) both Kc and Ki → 0 and therefore, since E = hn,
equal to its kinetic energy Kc. Toward the infinity, (where c∞ → 0) both Kc and Ki → 0 and therefore, since E = hn,
![]() (21)
(21)
represents the energy of one ray of light, (where photons are flowing) and where
![]() (22)
(22)
is the mass of light, having frequency ν, passing along one ray in 1s, while the constant
![]() (23)
(23)
where
![]() (24)
(24)
thus the Planck’s constant turns out to be the energy of one photon.
Now, since m is the mass of light passing along one ray in 1s, the term![]() , with nr the number of rays emitted by a source S, becomes the energy emitted by S in 1s; this unitary (unit of time) energy shall be equal to the supplied power P during 1s,yielding
, with nr the number of rays emitted by a source S, becomes the energy emitted by S in 1s; this unitary (unit of time) energy shall be equal to the supplied power P during 1s,yielding
![]() , (25)
, (25)
therefore
![]() (26)
(26)
is the total mass lost per second by a source of light; e.g. for a 1W lamp, we get![]() , while the number nr of rays is
, while the number nr of rays is
![]() (27)
(27)
in our case, nr ≅ 3 ´ 1018 rays. Then, for a given power P, the higher is the frequency, the lower is the number of rays, as shown by (27) written as nrν = P/h. The number of photons emitted in 1s is
![]() (28)
(28)
which, for P = 1 W, gives nγ = h−1 (=1.5 ´ 1033 photons/s), thus the inverse of Planck’s constant turns out to be the number of photons emitted in 1s by a source of unitary power, and this great number of photons allows the light to be treated as a wave.
Now, during inelastic impacts, (like on absorption or photoelectric effetcs), both kinetic and internal energy of the light are involved, so the momentum transferred to the electron is
![]() (29)
(29)
while, during elastic impacts, the momentum transferred to the electron is
![]() (30)
(30)
either for incident or for reflected photons, with Tʹ the total impact time for this interaction, as we show on Section 3.7, Compton effect; at this regard, via the (30), as well as via our Doppler effect equation, see (12), we get the Compton equation, which can not be obtained by the Relativity via their Doppler effect equations.
2.4. Re-Visitation of the Harvard Tower Experiment (HTE), Time Dilation, Gravitational Redshift
Also this argument has been widely treated on our previous paper [1] . Here the main results: referring to Figure 5(a), where h is the tower height, and ME is the mass of Earth, writing the Equation (8) as c2 = −2U, we can obtain
![]() (valid for
(valid for![]() ) (31)
) (31)
that is the variation of c from the tower base to its top, where c0 and ch are the corresponding values of c, ![]() is the variation of potential; hence ch < c0, with
is the variation of potential; hence ch < c0, with![]() .
.
Let now S be a Mossbauer source and A a related absorber, both, for instance, at the tower base, therefore in resonance. Then, see Figure 5(b), taking A to the top, and since A and S are now at rest, the frequency of the photons emitted by S, has to be equal to the one observed by A, that isnh=n0, therefore the Equation (31), as for the photons emitted by S, can be written as
![]() (32)
(32)
and since ch < c0, it must be λh < λ0, so, contrary to Relativity, A observes a blue-shift effect.
![]()
Figure 5. Harvard tower experiment (HTE) scheme; source at the base, our results.
Thus, to restore the resonance via Doppler Effect (DE), S and A, see Figure 5(c), have to recede from each other, in order that the photon length should increase, see Equation (12), from λh to ![]() with β = vAS/c º v/c, so, equating the photon length variation (Δλ/λ = −v/c due, see (17), to DE), to Δλ/λ, due to the altitude, as given by (32), we get
with β = vAS/c º v/c, so, equating the photon length variation (Δλ/λ = −v/c due, see (17), to DE), to Δλ/λ, due to the altitude, as given by (32), we get![]() , yielding
, yielding
![]() (for
(for![]() ). (33)
). (33)
This value is also predicted by GR which, implying a decrease of n for the light moving from the base to the top, predicts an opposite direction of v with respect to the one shown on Figure 5(c); at this regard, Pound-Rebka and Pound-Snider, [2] [3] [4] , gave no clear indication about the direction of the compensating velocity.
Moreover, see Figure 5(b), with S on the base, emitting upward, A goes out of resonance and since on our basesnh = n0, the non-resonance is physically related to a variation of λ.
Now, see Figure 6(a), with A on the base, taking S to the top, the experience shows that the absorber goes out of resonance. Indeed, with S on the top, the (31) shows ch < c0, but what about the initial parameters of the photons emitted in altitude, nh and λh?
Well, see Figure 6(b), since S and A are at reciprocal rest, the frequency of the photons arriving to the base, is nh-0 = nh, hence along the path top-base, the photon length λh-0 has to increase (following the increase of c from ch to c0); thus we have to argue that taking the source on top, see Figure 6(a), the photons initial length must be λh = λ0, in order that after the path top-base, it becomes λh-0 > λ0 (inducing the absorber to go out of resonance).This implies
![]() (34)
(34)
showing that, taking S to the top, nh < n0. Now, along the path top-base, Δλ/λ has opposite sign with respect to (32), yielding![]() , and since λh = λ0, we get
, and since λh = λ0, we get
![]() (35)
(35)
showing an increase of λ from the top to the base. Hence the absorber, on the base, will state a red-shift so, to compensate it via Doppler shift, see Figure 6(c), S and A have now to move relative to each other; on the contrary, according to Relativity, A and S should recede from each other.
![]()
Figure 6. Harvard tower experiment scheme; source on the top, our results.
Time dilation: Atomic clocks in altitude (h-clocks) are ticking faster than identical clocks on the ground (g-clocks): indeed, on our bases, at height h, see (30), we have![]() , while taking a clock to a GPS satellite, see also Figure 6(a), from (34), one finds
, while taking a clock to a GPS satellite, see also Figure 6(a), from (34), one finds
![]() (36)
(36)
with Th = 1/nh the counted time of one photon emitted by a h-clock, while T0 to a g-clock. Thus the variation of the counted time between the two clocks, for every emitted photon, becomes
![]() (37)
(37)
Now, the frequency of photons is their number emitted in 1 s along one ray, there- fore the term
![]() (with
(with![]() ) (38)
) (38)
is the number of photons (atomic transition of Cs 137) that constitute a one-second, so that
![]() (39)
(39)
is the variation ofn1s emitted in1 s by a h-clock; now, see (31), ΔU = MEGh/rhr0 and sincerh ≅ 26,600 km , r0 ≅ 6400 km, with h ≅ 20,200 km , the increase of counted time in one day (![]() ) of a h-clock, with respect to a g-clock, becomes
) of a h-clock, with respect to a g-clock, becomes
![]() (40)
(40)
Now, being v = 2(2πrh/86,400 = 3870 m/s the orbital speed of GPS satellites, (corres- ponding to two orbits/day), it turns out that the variation of the counted time, between a h-clock and an Observer E at the centre of Earth, due to their relative motion, is given by Equation (20) which, in our case, with β = v/c, becomes
![]() (valid for
(valid for![]() ) (41)
) (41)
with TE the photon counted time for the Observer E; then, with v0 the Earth’s rotational speed, and since![]() , we can write T0 ≅ TE with T0 the counted time of one photon for a g-clock; so, the difference between the two transit times Th and T0 ≅ TE given by (41), is
, we can write T0 ≅ TE with T0 the counted time of one photon for a g-clock; so, the difference between the two transit times Th and T0 ≅ TE given by (41), is
![]() (42)
(42)
Then, as above, ![]() is the variation of the number of photons emitted by a h-clock in 1 s; so the variation of the counted time, in one day, becomes
is the variation of the number of photons emitted by a h-clock in 1 s; so the variation of the counted time, in one day, becomes
![]() (43)
(43)
showing a decrease of the counted time for a g-clock due to the clocks relative motion; hence
![]() (44)
(44)
which is also predicted, (with different reason), by GR. To prevent the two said effects, before launching, the daily counted time (T1d) of clocks, has to be decreased by ≅38 μs; this adjustment is sufficient to obtain synchronization between h-clocks and g-clocks: indeed, on our bases, the frequency of photons, emitted by a h-clock, does not change along the straight path satellite-ground, whereas as for the Relativity, because of its predicted increase of the frequency along the straight path satellite-ground, a g-clock should go, (for this 3rd effect), out of synchronization.
Gravitational redshif
Referring to our previous paper [1] , we summarize, hereafter, the differences between the Relativity and our results: as for the Relativity, the only way to explain high cosmological redshifts is the Doppler effect, (which implies an incredible universe expansion at a speed vu ≅ c), whereas, on our results, disregarding the reciprocal motion between a (far) source and an Observer on Earth, which impliesn = n0, we get c/λ = c0/λ0, where n0, c0 and λ0 are the values on Earth, showing that for c0 > c it has to be λ0 > λ, that is a red shift. In general, the shifts observed on Earth can be therefore expressed as
![]() (45)
(45)
with U0 the potential on Earth, U the one on the source. Thus, apart from Doppler effects, z turns out to be the variation of c (as well as λ) during the path of light toward a different potential. For s < ≅ 45 Mpc, [5] , (corresponding to −0.01 < z < ≅0.01) if U (potential on the source) is, in absolute value, higher than the potential on Earth U0, the (45) gives, on Earth, z < 0 (blue shift), and vice versa for |U| < |U0|; thus, for s < ≅45 Mpc, these red/blue shifts indicate that the potential, may increase or decrease. In the range ≅0.01 < z < ≅0.20, (where z follows the Hubble’s law), the (45), written as
![]() (valid for
(valid for![]() ) (46)
) (46)
shows that, for![]() , U depends linearly on z, as Hubble’s law;then, for s→∞, U→0, hence z→∞, see Table 1.
, U depends linearly on z, as Hubble’s law;then, for s→∞, U→0, hence z→∞, see Table 1.
![]()
Table 1. Calculated values of U and c related to the observed shifts on Earth.
For s > ≅45 Mpc, z is always positive, hence we may argue that our galaxy is close to the centre of potential Cp of all the masses, (where |UCp| has the max value), practically close to the middle of the masses of universe.
3. Part 2―Interaction Light-Matter
3.1. Electron Structure and Photon-Electron Impact Point
On our basis, (light composed of our photons), the interaction light-matter requires that to move a circling electron toward outer orbits, the impact photon-electron has to occur, see Figure 7(a), in a radial way, (giving origin to the radial velocity w), otherwise, some impacts could cause the electron fall into the nucleus, due, for instance, to an impact where photons-electron have contrary direction.
![]()
Figure 7. Photon-electron Impact point (Ip) and electron radial velocity w.
To be radial, the impact must occur in a specific point (Impact Point, fixed to the electron) which, during the electron revolution, has to face its nucleus, (up to its removal), giving to the electron one rotation every revolution. Thus we can infer that the electric charge of the electron, has to correspond to the Impact Point (Ip), and we have also to argue that each photon front is provided with a positive charge, while its tail with an equal negative one.
Moreover, in case of more impacts, as it happens, for instance, on Absorption/ Emission effect, where the impacts move a circling electron toward higher orbits, the impacts photons-electron have to occur all around the electron orbit, thus the impacting photons have to approach the nucleus, as shown on Figure 7(b), perpendicularly to the electron orbit plane, providing, to the electron, a radial velocity w.
3.2. H Atom Parameters (On Our Bases) and Meaning of Its Quantum Numbers
![]()
Figure 8. H atom configurations, (on our bases), at constant total energy. (a) Observed from the electron-proton common centre of gravity B; (b) Ditto, with the electron barycentre now coincident with its proper charge; (c) Observed from the proton fixed as origin, orbited by the electron having now a reduced mass.
The Figure 8(a) represents an H atom with both the electron mass me and the proton mass mp circling around B (centre of mass of the system electron-proton), and where, on our bases:
rB is the ground-state orbit of the electron charge,
re the electron radius,
rp the proton ground-state orbit,
ve = |ve|, the electron ground-state (orbital) speed,
vp the proton ground-state speed.
Hence, the ground-state orbit of the electron barycentre turns out to be
![]() , (with
, (with![]() ). (47)
). (47)
Now, to apply properly the equality between the electron centrifugal force and the Coulomb force, we have to consider the configuration (c) where the electron reduced mass
![]() (with
(with![]() ), (48)
), (48)
is circling around the proton fixed as origin (O). Now, the total energy of the configurations (a) and (b) are:
Configuration (a), where![]() ;
;
![]()
Configuration (b), where![]() ;
;
![]()
hence for Ta = Tb we get ![]() = ve and
= ve and ![]() = vp. (As for Tc = (Ta = Tb), next chapter).
= vp. (As for Tc = (Ta = Tb), next chapter).
Now, see Figure 8(b), ![]() can be found from the relation
can be found from the relation ![]() yielding
yielding
![]() (49)
(49)
thus
![]() (50)
(50)
Now, see Figure 8(c), equating along a circular orbit, the electron centrifugal force to the Coulomb one
![]() , (51)
, (51)
and calling ![]() the necessary and sufficient energy to move the electron charge from r toward ¥, and assuming U¥ = 0, we get
the necessary and sufficient energy to move the electron charge from r toward ¥, and assuming U¥ = 0, we get
![]() (52)
(52)
where ![]() is the potential due to electrostatic attraction. So the (51) becomes
is the potential due to electrostatic attraction. So the (51) becomes
![]() (53)
(53)
Now, the orbital kinetic energy of mr is![]() , thus, with W the related ionization energy, (that is the electron extraction work), we may write
, thus, with W the related ionization energy, (that is the electron extraction work), we may write
![]() (54)
(54)
The term E = hn, see (21), is the energy of light passing along one ray, thus, if the ionization energy W (![]() ) is supplied by one ray of light (with energy E = hn) it must be
) is supplied by one ray of light (with energy E = hn) it must be
![]() (55)
(55)
Therefore, substituting 2hn (![]() ) into (51) and solving by r we get
) into (51) and solving by r we get
![]() (56)
(56)
Now, plugging into (56) the value of the highest H-atom spectrum frequencyn0 = c/λ0 = cRH where RH (=1/λ0) is the Rydberg constant, whose experimental value is RH = 10,967,758 m-1, we get, as for H atom, see Figure 8(c), the ground-state orbit (referred to the proton) of the reduced electron
![]() (57)
(57)
with α = e2/2εohc the fine structure constant. Then writing the (50) as![]() , we get
, we get
![]() (58)
(58)
corresponding to the Bohr radius, with![]() , whereas, on our bases, rB corresponds, see Figure 8(b), to the ground-state orbit of the electron around the electron-proton centre of mass B.
, whereas, on our bases, rB corresponds, see Figure 8(b), to the ground-state orbit of the electron around the electron-proton centre of mass B.
Now, the speed of mr along the orbit r0, from (55),becomes
![]() (59)
(59)
and given the frequency of the electron mr along r0 that is ne0 = vr0/2πr0, the ratio 2n0/ne0, with r0 (=α/4πRH) as given by (57), becomes
![]() (60)
(60)
hence it is consistent to assume 2n0/ne0 = 1 (exactly). This ratio, written 2Te0 = T0, implies that, on H atom, the light-electron impact time T0 lasts for two electron orbits.
Now, it is known that the admitted wavelengths, along circular orbits, have to satisfy the relation λn = n2λ0, with ![]() an integer, so we can also write
an integer, so we can also write
![]() (61)
(61)
withnn the photons admitted frequency along circular orbits. Then from (56) we can write
![]() (62)
(62)
representing the radius of each circular orbit. Then, see (59), the orbital speed of the electron mr along any circular orbit is
![]() (63)
(63)
while its frequency is
![]() . (64)
. (64)
Then, dividing (61) by (64) and because of the ratio 2no/neo = 1, we get
![]() (65)
(65)
Now, asn (= n/t) is the number n of photons passing along one ray during t, for t = 2Te one gets n = n/2Te = nne/2, which equals the (65), so the integer n of (65) also represents the number of photons (of the same ray) absorbed (or emitted) by the electron during 2Te and this number, for all the n circular orbits of H atom, is an integer starting with 1 along the two orbits related to the photon n0. [Between two circular orbits, the photons frequency are shown on Section 3.5].
The (65) written as nTn = 2Ten shows that the impact time nTn of n photons (with frequency nn) equals the time needed by the electron, along the orbit rn, for two orbits.
For n = 1, the Equation (65) corresponds to 2n02πr0/vr0 = 1 and substituting here r0 (=e2/8πε0hn0), as given by (57), we get 4πn0e2/8πε0hn0vr0 = 1, that is
![]() (66)
(66)
same value given by (59). Now, comparing (59) to (66) we find
![]() (67)
(67)
matching the RH experimental value.
3.3. Impact Photon-Electron and Electron Radial Speed
![]()
Figure 9. H atom configurations. (a) observed from the common centre of gravity B. (b) ditto, with the electron centre now coincident with its proper charge. (c) observed from the nucleus fixed as origin, with the reduced electron massmr. (d) observed from the nucleus, with mi (here called electron impact mass) circling along the effective orbit![]() , with two reduced charges (−er, +er).
, with two reduced charges (−er, +er).
Still referring to H atom, to apply properly the Conservation of momentum (CoM) to the impact photon-electron, we need, see Figure 9, a proper configuration where its nucleus should be fixed in the common centre of gravity B orbited by an equivalent electron mass m, as shown on Figure 9(d).
The Figure 9 shows the necessary passages, from Figures 9(a)-(d), to obtain such a configuration.
Referring to Figure 9(c), with mr circling around![]() , with orbital speed v0, the (51) gives
, with orbital speed v0, the (51) gives
![]() (68)
(68)
and substituting, see (50), ![]() , we can write
, we can write
![]() (69)
(69)
then calling
![]() (70)
(70)
electron impact mass, we get
![]() (71)
(71)
and therefore
![]() (72)
(72)
we can now write
![]() where
where ![]() (73)
(73)
showing, see Figure 9(d), m circling along the orbit![]() , implying an electron/proton reduced charge er. Now, referring to Figure 9(c), we have
, implying an electron/proton reduced charge er. Now, referring to Figure 9(c), we have![]() , and since, see (66), vr0 = αc, the orbital speed of mr along the orbit
, and since, see (66), vr0 = αc, the orbital speed of mr along the orbit ![]() becomes
becomes
![]() (74)
(74)
which, given εm and εr, leads to v0 = c/137, as shown on next chapter.
We compare now the total energy T of the system electron-proton along the configurations of Figure 9: as for Figure 9(a) and Figure 9(b), the total energy of the system is![]() ; as for Figure 9(c), along r0, we get
; as for Figure 9(c), along r0, we get![]() ; on 6(d),
; on 6(d),![]() .
.
The conservation of momentum, applied before and after an inelastic impact photon-electron, since photon and w, see Figure 7, have same direction, the (29), for a generic atom, gives
![]() (75)
(75)
representing the electron radial speed originated by an impact of one photon during the impact time T, while for n photons (with frequency ν) we have
![]() . (76)
. (76)
Regarding now the H atom and referring to Figure 9(c), meaning to consider the electron reduced mass mr circling along r0, the (75) forn = n0, becomes
![]() (77)
(77)
and since, see (59), ![]() , as shown by (66), we get
, as shown by (66), we get
![]() (78)
(78)
while considering the configuration 9(d), where the electron mi is circling along![]() , we get
, we get
![]() (valid for
(valid for![]() ) (79)
) (79)
close to c/1372 = 15,972.743 m s-1; on next chapter, via re, we get w0 = c/1372 and v0 = c/137.
3.4. Ionization Condition, Number of Electron Circular Orbits (H Atom), and Electron Radii
Now, referring to Figure 10, let us consider an electron (me) circling, with velocity v around its nucleus with mass![]() , which can therefore be considered as fixed in the atom centre of gravity B; its removal may happen when its radial speed w equals v (=|v|) that is
, which can therefore be considered as fixed in the atom centre of gravity B; its removal may happen when its radial speed w equals v (=|v|) that is
![]() (80)
(80)
In particular, as for H atom, and referring to Figure 9(c), let us consider the electron mr circling along r0; comparing (78), that is w0 = α2c, with (66) that is vr0 = αc, we get![]() .
.
Now, along r0, (ground-state orbit), we have n = 1 (meaning one photon along the double orbit r0), hence the ionization, requiring w0/vr0 = 1, cannot happen along r0. Referring now to Figure 9(d), along the ionization double orbit (in short d-orbit) # ni, where the photons incident frequency, see (61), is![]() , the impact due to niphotons would produce, considering the electron impact mass mi, an electron radial speed, see (76), equal to
, the impact due to niphotons would produce, considering the electron impact mass mi, an electron radial speed, see (76), equal to
![]() (81)
(81)
and since along this orbit (ni), see (63), is vi = v0/ni, where v0 is given by Equation (74), we find
![]() (82)
(82)
hence the ionization, which requires wni = vi, with only ni photons along the nithd-orbit, would never happen; thus we must infer that there are 137 progressive d-orbits, where the electron is circling n times along every d-orbit; thus the number of photons admitted along n d-orbits turns out to be n2, yielding to the radial speed, along n ionization d-orbits ni, the value ![]() which becomes
which becomes
![]() (83)
(83)
giving the same radial speed wo for any circular d-orbit, see Table 2.
![]()
Table 2. Ionization parameters of H atom (on the last column, 3rd line, change ![]() according to the new value
according to the new value![]() ).
).
Now we can obtain the radius (re) of the electron: indeed, along the ionization d-orbit ni, the ionization condition ![]() becomes w0 = v0/ni, and plugging v0 as giving by (74) we get
becomes w0 = v0/ni, and plugging v0 as giving by (74) we get
![]() (84)
(84)
where w0 is given by (77) and since![]() , see (67), we have
, see (67), we have
![]() (85)
(85)
but ni has to be an integer so we can infer ni = 137, giving
![]() (86)
(86)
yielding
![]() (87)
(87)
Now, the correct values of mi, wo and vo from (70), (79), (74), with εr given by (86), become
![]() (88)
(88)
![]() (89)
(89)
![]() (90)
(90)
Now, the (84), that is ni = v0/w0, through (89) and (90) yields
![]() (91)
(91)
where ni, on Absorption effect, is a specific constant representing the number of circular orbits as well as the numbers of admitted photons along theionization orbit.
3.5. Absorption/Emission Effect: Photons Admitted Frequencies, Claimed Fall of Circling Electron
Referring to Figure 11, where we represent the Absorption of photons from acircling electron, let us assume the nucleus mass![]() , so to consider the nucleus fixed in the atom centre of gravity B. Now, the expression of the total energy of the system photon-electron is given by
, so to consider the nucleus fixed in the atom centre of gravity B. Now, the expression of the total energy of the system photon-electron is given by
![]() (92)
(92)
where E(=mc2) is the energy of the incident light, Ur(=−e2/4πε0r) is the potential due to electrostatic attraction acting on the electron, Ke(=![]() mev2) is the electron orbital kinetic energy, and Kr(=
mev2) is the electron orbital kinetic energy, and Kr(=![]() mew2) its radial kinetic energy (related to its radial speed w).
mew2) its radial kinetic energy (related to its radial speed w).
![]()
Figure 11. Absorption effect: Incident photons are absorbed by the electron which moves toward higher orbits; when re-emitted, (electron moving toward inner orbits), have contrary direction.
Regarding the Absorption/Emission effect (elements on gaseous form), see Figure 11, along circular orbits it is Kr = 0 and since, at the end of absorption, along the orbit r2, (where the photons have been absorbed), it is E2 = 0, between two circular orbits r1 and r2, the (92) gives
![]() . (93)
. (93)
Now, from (53), Ur = −mev2, and since Ke = ½mev2, we get ![]() so from (93) we have
so from (93) we have![]() ; thus, as E1 = hν, and since mev2 = e2/4πε0r we find
; thus, as E1 = hν, and since mev2 = e2/4πε0r we find
![]() (94)
(94)
Then, according to (62) we have r1 = r0n2 and r2 = r0k2 (with k > n as r2 > r1), thus
![]() (95)
(95)
and plugging the (57) written as n0 = e2/8πε0hr0, we find
![]() (96)
(96)
which is the photons frequency between two circular orbits, where n, as showed on Section 3.2, is an integer representing the (progressive) number of each circular orbit, and where k turns out to have the values ![]() which is, one by one, the number of the remaining external circular orbits.
which is, one by one, the number of the remaining external circular orbits.
Claimed fall of a circling electron into its nucleus: an electrical current emits an electro-magnetic radiation and therefore it is claimed that the circulating electrical charge of an electron should also emit an e.m. radiation yielding the electron, in a short time, to fall into the nucleus; but on our results, a free electron, moving, for instance, along a copper wire under an electrical potential difference, when entering into an atom influence, (at that moment the electron charge will return to face the atom nucleus), will release the necessary photons to reach the atom energy level corresponding to the energy previously received (during the absorption effect). Indeed, along circular orbits, it is w = 0, therefore the absorption/emission of photons may only start/finish along these orbits, thus the circling electrons are absorbing/emitting photons only between circular orbits, so the e.m. radiation related to an electrical current is due to the emitted photons during their re-entry to an atom; by the way, the photons emission is necessary for the electron not to fall into the nucleus.
3.6. Photoelectric Effect: Number of Photons Necessary for the Atom Ionization
Between the electron ground-state orbit r0 and its extraction orbit r ® ¥ (intending on microscopic scale), the (92), valid for every interaction light-matter, gives
![]() (97)
(97)
with E' the energy of re-emitted light, wae the electron radial speed after its extraction, (![]() its kinetic energy), while the other terms have been defined referring to (92).
its kinetic energy), while the other terms have been defined referring to (92).
On ground-state, as also shown between Equations ((93) and (94)), it is
![]() (98)
(98)
with v0 the electron speed along r0 and with Wf the Work function (electron extraction
work); now, at the start of impact, w = 0 giving![]() , while for r ® ¥, the
, while for r ® ¥, the
electron orbital speed v¥![]() ® 0, so
® 0, so![]() , and (97) gives
, and (97) gives
![]() (99)
(99)
where ![]() is the total kinetic energy transferred from light to elec- tron. On Photoelectric Effect (PhE), the light scatters off an electron (Kae ≥ 0), but it is not re-emitted, (E' = 0), so the (99), with nf (=Wf/h) the specific threshold frequency, becomes
is the total kinetic energy transferred from light to elec- tron. On Photoelectric Effect (PhE), the light scatters off an electron (Kae ≥ 0), but it is not re-emitted, (E' = 0), so the (99), with nf (=Wf/h) the specific threshold frequency, becomes
![]() (100)
(100)
showing that forn = nf there is ionization with wae = 0.
At frequency nf the electron radial speed wf, due to the impact of one photon, see Equation (75), is![]() , and writing the (55) as v0 = (2Wf/me)1/2, we get
, and writing the (55) as v0 = (2Wf/me)1/2, we get
![]() (101)
(101)
and since the values of Wf are in the range 2 - 6 eV, the (101) gives wf/v0 @ 0.0028 - 0.0048 meaning that the ionization, requiring w = v0, at frequencynf needs nf photons, as follows: the electron radial speed due to nf photons with frequency nf, see (76), is![]() , so the ionization condition (w = v) becomes wnf = vo leading to
, so the ionization condition (w = v) becomes wnf = vo leading to ![]() giving
giving
![]() (102)
(102)
that is, on PhE, the number of photons at frequency nf necessary for ionization(wae = 0). Now, if nf photons, at frequencynf, are sufficient for ionization, then the frequency
![]() (103)
(103)
is sufficient for ionization (wae = 0) with one photon only, meaning that ν1 is the threshold between PhE and Compton effect which requires one photon only, as shown on next chapter.
Now let us find the number n1 (giving wae max) of the impacting photons at frequency n1: writing the (100) as hn = 1/2mew2, (energy transferred from a ray of light to an electron), one gets w = (2hn/me)1/2 which has to be equal to the electron radial speed due to n photons, wn = 2nhn/cme, see (76); so, forn = n1 and n = n1, we get ![]() yielding
yielding
![]() (104)
(104)
with n1 the number of impacting photons at frequency n1 and plugging nf given by (102), we find
![]() (105)
(105)
meaning that on PhE, the number of impacts photons-electron varies from nf related to the frequency nf to ![]() related to the max admitted frequencyn1 (=nfnf). For instance as for caesium (Cs), having Wf @ 2 eV, since
related to the max admitted frequencyn1 (=nfnf). For instance as for caesium (Cs), having Wf @ 2 eV, since![]() , we may infer nf = 361 leading to n1 =19, while as for Pt, having Wf @ 6 eV, we may infer nf = 196 leading to n1 = 14.
, we may infer nf = 361 leading to n1 =19, while as for Pt, having Wf @ 6 eV, we may infer nf = 196 leading to n1 = 14.
3.7. Compton Effect: Number of Photons Involved and Compton Equation via Doppler Effect
Here, see Figure 12, the incident photon (length λ, frequency n), while ejecting a circling electron is also reflected (λ', n') so the recoiling electron, emitting a photon λ' toward the Observer A, represents a source in motion from A along the direction w, implying an undoubted Doppler effect.
![]()
Figure 12. Compton effect (CE). φ: angle between the direction of the incident photon and the scattered one (λ'); θ: angle between the direction of the incident photon λ and the recoiled electron; θ' (= π ? φ − θ): it will be shown that θ' = θ.
Now, on the basis that the scattered photon starts to be reflected at the same time when the incident photon starts to hit the electron, and since ![]() is the emission time of the reflected photon, it turns out that
is the emission time of the reflected photon, it turns out that ![]() is also the whole interaction time, meaning that there is not a complete absorption of the incident photon followed by an emission. Now, with
is also the whole interaction time, meaning that there is not a complete absorption of the incident photon followed by an emission. Now, with ![]() the whole impact time photon-electron, the momentum transferred from the incident light to the electron, as per (30), is
the whole impact time photon-electron, the momentum transferred from the incident light to the electron, as per (30), is ![]() and the same value is then transferred from the reflected photon to the electron, so the Conservation of Momentum (CoM) along the direction normal to w, becomes
and the same value is then transferred from the reflected photon to the electron, so the Conservation of Momentum (CoM) along the direction normal to w, becomes
![]() (106)
(106)
giving![]() . Then, the length of the reflected photon, for the Observer A, see Equation (12) is
. Then, the length of the reflected photon, for the Observer A, see Equation (12) is
![]() (107)
(107)
where Δλ = wAT' and where wA = wcosθ is the component of the electron speed along the direction of the Observer A and ![]() is, for A, the photon transit time, so we get
is, for A, the photon transit time, so we get
![]() (108)
(108)
Now the CoM along w is
![]() (109)
(109)
giving
![]() . (110)
. (110)
Then, plugging this value into (108) we get
![]() (111)
(111)
Now, ![]() ,
, ![]() , hence
, hence ![]() and therefore
and therefore
![]() (112)
(112)
and since![]() , we get the Compton equation:
, we get the Compton equation:
![]() (113)
(113)
which cannot be obtained via the Doppler effect relativistic equations regarding the light.
Then, the (110), for cosθ = 1, equals the (75), implying the impact of one photon only.
Now, the (111), for cosθ = 1 gives![]() , or
, or ![]() which plugged into (110) gives
which plugged into (110) gives
![]() (114)
(114)
yielding, see Figure 13, w → c for n → ∞, whereas, for inelastic impacts, w is proportional ton.
Then, as for the electron radial speed after its extraction, here indicated v, from (100) we have Wf + Kae = 1/2mew2 where Kae = 1/2men2 and since ![]() as shown by (98), we get
as shown by (98), we get
![]() (115)
(115)
which for w = v0, (ionization condition) gives n = 0, as represented on the Figure.
![]()
Figure 13. Relation between the incident frequencynand the electron radial speed (w) due to one photon.
4. Conclusions
The Relativity arose as a result of attempts to explain the (apparent) constancy of the speed of light which was supported by many experiments: in fact, on our bases/results, there is, on Earth, a continuous variation of co (c on Earth) which, from Equation (8), can be written Δc = −ΔU/co with ΔU the variation of the total potential on Earth (mainly due to the variable distance (d) Earth-Sun); indeed, between Aphelion and Perihelion (ΔdPA @ 5 ´ 109 m), we get ![]() well lower than the accuracy of the measured value of co. Anyhow, under the assumption that the light is composed of longitudinal-extended elastic and massive particles (photons) emitted at a speed equal to the total escape speed, we showed that the speed of light, under a constant total potential, is constant for every Observer, in accordance with the Newtonian laws.
well lower than the accuracy of the measured value of co. Anyhow, under the assumption that the light is composed of longitudinal-extended elastic and massive particles (photons) emitted at a speed equal to the total escape speed, we showed that the speed of light, under a constant total potential, is constant for every Observer, in accordance with the Newtonian laws.
Moreover, the Relativity Theory may last until a contrary experiment: well, an update experiment, similar to the Harvard tower experiment, would show that the direction of the compensating velocity, (between the source and the absorber), is contrary, as per our results, to the one predicted by the Relativity.
The Quantum Mechanics arose as a result of attempts to explain the discrete spectrum of the hydrogen atom: here, a revised electron structure, an H atom new configuration, and the introduction of these photons for the interaction light-matter, gave a Newtonian answer to that question.
On the Appendix, we have described, in short, the main differences between the Relativity and our results, as well as between QM and our results.
Appendix
Comparison sheet, (short summary), between the Relativity Theory and our results.
Abbreviations: S = source (of light); n = freq. of light stated by S; O = Observer; nO = freq. stated by O; U = totalgrav. potential.
![]()
Symbols
E (= mc2) energy of the light flowing along one ray,
m = mass of light passing along one ray in 1s,
n = photons frequency (number of photons of the same ray, crossing an Observer, in 1s),
T = photon transit time (time for one photon to cross an Observer),
γ (= mT) mass of light passing along one ray during T,
n0 = photon admitted frequency along the electron ground-state orbit, on H atom
nn = photon admitted frequency along the nth circular electron orbit on H atom,
nf (= Wf/h): specific threshold frequency on Photoelectric effect (PhE),
n1 = max admitted frequency on PhE; also minimum frequency able to produce the Compton effect,
εm º me/mP (where me= electron mass and mp = proton mass),
r0 = ground-state electron orbit on H atom: orbit of mr referred to mp (see Figure 8),
rB = Bohr radius: electron charge orbit, referred to common centre of gravity (CCG) electron-proton,
![]() = adjusted Bohr radius: electron centre of mass referred to the CCG electron-proton,
= adjusted Bohr radius: electron centre of mass referred to the CCG electron-proton,
εr º re/rB (where re = electron radius),
ne = electron frequency,
ne0 = electron frequency on ground-state orbit r0, H atom,
nen = electron frequency along the nth orbit, H atom,
v = generic speed, also electron orbital speed,
w = electron radial speed, due to the impact of one photon, referred to the atom centre of gravity,
w0 = electron radial speed due to the impact of one photon with frequency n0,
wf = electron radial speed due to the impact of one photon with frequency nf,
wn = electron radial speed due to the impact of n photons,
wn0 = electron radial speed due to the impact of n photons with frequency n0,
ni = number of admitted photons along the ionization orbit,
wni = electron radial speed due to the impact of n photons with frequency ![]()
![]() = electron radial speed due to the impact of n2 photons,
= electron radial speed due to the impact of n2 photons,
Kr (=![]() mew2) = electron radial kinetic energy,
mew2) = electron radial kinetic energy,
wae = electron radial speed after extraction (on macroscopic scale), on photoelectric effect (PhE),
Kae = electron radial kinetic energy after extraction (on macroscopic scale), on PhE,
v = electron radial speed after extraction (on macroscopic scale), on Compton effect.