Introduction to Logarithm
Last Updated :
31 Dec, 2024
Logarithm is a mathematical function that represents the exponent to which a fixed number, known as the base, must be raised to produce a given number. In other words, it is the inverse operation of exponentiation.
Mathematical Expression for Log
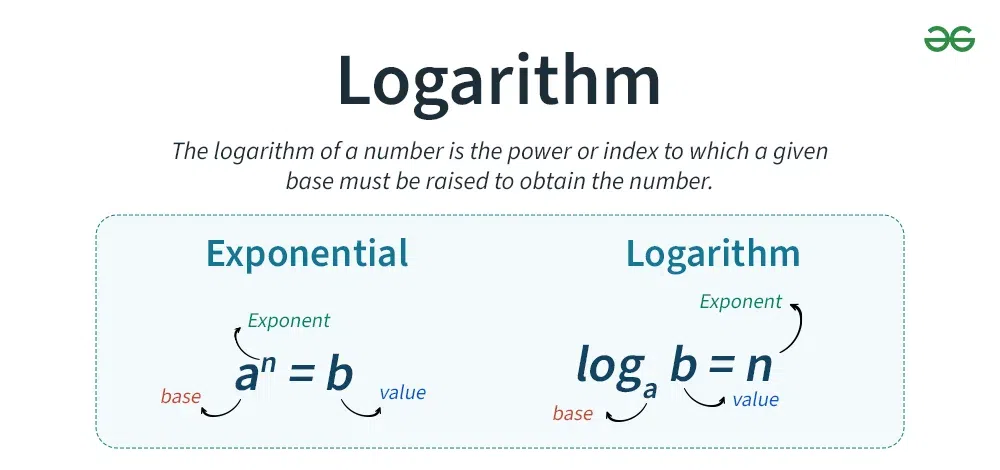
If an = b then log or logarithm is defined as the log of b at base a is equal to n. It should be noted that in both cases base is 'a' but in the log, the base is with the result and not the power.
an = b ⇒ logab = n
- a is Base
- b is Argument
- a and b Positive Real Numbers
- n is Real Number
Conversion from Exponential to Log Form
If a number is expressed in the exponential form for example an = b where a is the base, n is the exponent and b is the result of the exponent then to convert it into the logarithmic form the base 'a' remains base in logarithm, the result 'b' becomes an argument and the exponent 'n' becomes the result here.
an = b ⇒ logab = n
Conversion from Log to Exponential Form
If the expression is in logarithmic form then we can convert it into exponential form by making the argument as a result and the result of logarithm becomes the exponent while the base remains the same. It can be better understood from the expression mentioned below:
logab = n ⇒ an = b
Types of Logarithm
Depending upon the base, there are two types of logarithm, which are,
Common Logarithm
The logarithm with base 10 is known as Common Logarithm. It is written as log10X. The common logarithm is generally written as log only instead of log10.
Let's see some examples.
- log10 = log1010 = 1
- log1 = log101 = 0
- log1000 = log101000 = 3
Natural Logarithm
The logarithm with base e, where e is a mathematical constant is called Natural Logarithm. It is written as logeX. The natural logarithm is also written in the abbreviated form as ln i.e. logeX = ln X.
Let's see some example:
- loge2 = X ⇒ eX = 2
- loge5 = y ⇒ ey = 5
Difference between Common Logarithm (Log ) and Natural Log (ln)
The basic difference between log and ln is tabulated below:
| Log | Ln |
|---|
| Log is the logarithmic expression at base 10. | Ln is the logarithmic expression at base e. |
| It is given as log10X | It is given as logex |
| It is called a common log and is represented as log x | It is called natural log and is represented as ln x |
| It is mostly used for solving large numbers and simplifying calculations. | It is less commonly used |
Learn More: Difference Between Log and Ln
Rules of Logarithm | Log Rules
The common properties or rules of Log are :
- Product Rule
- Division Rule
- Power Rule
- Change of Base Rule
- Base Switch Rule
- Equality of Log
- Number raised to Log Power
- Negative Log Rule
Read in Detail: Logarithm Rules | List of all the Log Rules with Examples
Apart from the above-mentioned properties, there are some other properties of Log. Using these properties we can directly put their values in any equation. These properties are mentioned below:
- Log 1 =0 This property of log states that the value of Log 1 is always zero, no matter what the base is. This is because any number raised to power zero is 1. Hence, Log 1 = 0.
- Logaa This Property of log states that if the base and augment of a logarithm are the same then the logarithm of that number is 1. This is because any number raised to power 1 results in the number itself. Hence, ln e = logee = 1 r.
- The value of log of zero is not defined as there is no such number when raised to any power that results in zero. Hence, log 0 = Not defined.
Log and Antilog Table
Log Table
Log Table is used to find the value of the log without the use of a calculator. The log table provides the logarithmic value of a number at a particular base.
A log table has mainly three columns. The first column contains two-digit numbers from 10 to 99, the second column contains differences for digits 0 to 9 and hence called the difference column and the third column contains mean difference from 1 to 9 and hence called mean difference column.
The log table for base e is called the natural logarithm table and that for base 2 is called the binary log table.
The logarithmic value of a number contains two parts named characteristics and mantissa both separated by a decimal. Characteristic is the integral part written o the left side of the table and can be positive or negative while the mantissa is the fraction or decimal that is always positive.
Read More : Log Table
Anti Log Table
Antilog is the process of finding the inverse of the log of the number. This is used when the number is already given in log value and we need to find out the number for which log value is given. If log a = b then a = antilog (b).
Antilog table is helpful in finding the Antilog value without using the calculator. The antilog table also consists of 3 columns among which the first column contains numbers from .00 to .99, the second block which is the difference column contains digits from 0 to 9, and the third block which is the mean difference column contains digits from 1 to 9.
Read More: Antilog Table
Logarithmic Function
A logarithmic function is the inverse of an exponential function and is defined for positive real numbers with a positive base (not equal to 1). The logarithmic function to the base b is represented as f(x) = logb(x), where x>0 and b>0. In this function, X is the argument of the logarithm, and b is the base.
Graph of Logarithmic Function
A logarithmic function is the inverse of an exponential function and is defined for positive real numbers with a positive base (not equal to 1). The logarithmic function to the base b is represented as f(x) = logb(x), where x>0 and b>0. In this function, X is the argument of the logarithm, and b is the base.
We know that the domain of Logarithmic Function is (0, ∞) and its range is a set of all real numbers. If we plot the graph using the set of domain and range we find that the graph of the logarithmic function is just the inverse of the graph obtained for the exponential function.
.png) Logarithmic Graph
Logarithmic GraphThis indicates the inverse relationship between exponential and logarithmic functions. Also, the logarithmic graph is symmetric around the line y = x. We know that the value of log 1 is zero at any base value. Hence it has an intercept (1,0) on the x-axis and no intercept on the y-axis as log 0 is not defined.
Articles Related to Logarithms
Solved Examples on Logarithms
Example 1: Find loga16 + 1/2 loga225 - 2loga2
Solution:
loga16 + 1/2 ✕ 2loga15 - loga22
⇒ loga16 + loga15 - loga4
⇒ loga(16 ✕ 15) - loga4
⇒ loga(16 ✕ 15/4) = loga60
Example 2: Solve logb3 - logb27
Solution:
log23 - log248
⇒ log2(3/48)
⇒ log2(1/16)
⇒ log2(-16)
⇒ -log224
⇒ -4log22 = -4
Example 3: Find x in logbx + logb(x - 3) = logb10
Solution:
Given logbx + logb(x - 3) = logb10
⇒ logb(x)(x - 3) = logb10
⇒ (x)(x - 3) = 10
⇒ x2 - 3x - 10 = 0
⇒ x2 - 5x + 2x - 10 = 0
⇒ x(x - 5) + 2(x - 5)
⇒ (x - 5)(x + 2) = 0
⇒ x = 5, -2
People Also Read,
Logarithm - FAQs
What are Logarithms?
Logarithm is the inverse of exponential which is used to find out to what power a base must be raised to yield a particular value.
What are Different Types of Logarithms?
There are two types of Logarithms:
- Common Logarithm (Base 10)
- Natural logarithm (Base e)
Who Invented Logarithm?
Logarithm was invented by Scottish Mathematician John Napier in 1614.
Can Logarithm be Negative?
No the argument of logarithm can't be negative, however, log of any number can give a negative value.
What are Logarithms used for?
Logarithm is used to find the power a number must be raised to yield a particular result. It is useful in finding pH level, exponential growth or decay, etc.
What is the value of loga0?
Logarithm does not take 0 and negative numbers as its input hence loga0 is not defined.
Similar Reads
Logarithmic Function
Logarithmic functions are referred to as the inverse of the exponential function. In other words, the functions of the form f(x) = logbx are called logarithmic functions where b represents the base of the logarithm and b > 0. Concept of logarithm in mathematics is used for changing multiplication
6 min read
Logarithmic Differentiation
Method of finding a function's derivative by first taking the logarithm and then differentiating is called logarithmic differentiation. This method is specially used when the function is type y = f(x)g(x). In this type of problem where y is a composite function, we first need to take a logarithm, ma
8 min read
Solving Logarithmic Equations
A logarithm is a mathematical function that helps to determine the power to which a given number, called the base, must be raised to obtain another number. The logarithm of a number x to the base b is denoted as logbx and logarithmic equation is an equation that involves a logarithm with a variable
7 min read
Logarithmic Functions Practice Problems
Logarithmic Functions Practice Problems help students grasp the concept of logarithms through hands-on exercises and examples. These problems are designed to deepen understanding and proficiency in applying logarithmic functions in various mathematical contexts. Logarithmic functions are essential i
4 min read
Exponential and Logarithmic Functions
Logarithmic functions are the inverses of exponential functions. The inverse of the exponential function y = ax is x = ay. The logarithmic function y = logax is defined to be equivalent to the exponential equation x = ay. y = logax only under the following conditions: x = ay, a > 0, and a≠1. In t
8 min read
Derivative of Logarithmic Functions in Calculus
Derivative or Differentiation of Logarithmic Function as the name suggests, explores the derivatives of log functions with respect to some variable. As we know, derivatives are the backbone of Calculus and help us solve various real-life problems. Derivatives of the log functions are used to solve v
10 min read
Applications of Logarithms in Real Life
Logarithms represent the inverse operation of exponentiation. They answer the question, "To what power must a given base be raised to produce a specific number?" Mathematically, if bx = y after, then logb(y) = x. We can see logarithms in daily life through scales that measure various things, such as
3 min read
Laws of Logarithms
The logarithm is the exponent or power to which a base is raised to get a particular number. For example, 'a' is the logarithm of 'm' to the base of 'x' if xm = a, then we can write it as m = logxa. Logarithms are invented to speed up the calculations and time will be reduced when we are multiplying
4 min read
Logarithm Formulas
Logarithm is defined as the power to which a number is raised to yield some other values. Logarithms are the inverse of exponents. There is a unique way of reading the logarithm expression. For example, bx = n is called as 'x is the logarithm of n to the base b. There are two parts of the logarithm:
6 min read
Logarithms
In mathematics, a logarithm is the inverse operation of exponentiation. It is defined as the power to which the base number must be raised to get the given number. Logarithms serve as mathematical tools that help simplify complex calculations involving exponential relationships. If you know that bx
3 min read
Find a and b for a given Logarithmic Equation
Given a number n. Find positive numbers a(0 < a <= 109) and b(0 < b <= 109) such that they satisfy the equation log2a + log3b = n. Return any possible integer array of a and b, if the equation can be satisfied otherwise return the single integer array containing -1 which means there is n
8 min read
Tips and Tricks to Logarithm Question
Logarithms are a crucial topic to master for the Quantitative Aptitude section of competitive exams, which requires not only understanding but also speed and accuracy. This article provides essential tips and tricks for tackling logarithm questions effectively, covering key concepts, formulas, and r
4 min read
Change of base rule for Logarithm
The change of base formula is a useful concept in mathematics. That allows you to convert a logarithm from one base to another. Change of base formula in logarithm allows us to rewrite a logarithm with a different base. It allows us to compute logarithms using calculators or computational tools that
4 min read
Logarithmic Scale
Logarithmic Scale: On a chart, a logarithmic or log scale can be used to present big data or statistics clearly and understandably. Log scale knowledge is essential if you plan to work in an area like computer science, data analysis, research, finance, or business intelligence. It can help you displ
8 min read
What is the power notation of 343?
In mathematics, the terms "exponents and powers" are used when a number is multiplied by itself several times. A number is raised to the power of a natural number is equivalent to the number of times the number is multiplied by itself. When a number is multiplied by itself n times, the expression ob
6 min read
Program to compare m^n and n^m
Given two positive integers m and n, the task is to write a program that checks whether m^n is greater than, less than, or equal to n^m. Examples : Input: m = 3, n = 10 Output: m^n > n^m Explanation : 3^10=59049 which is greater than 10^3=1000 Input: m = 987654321, n = 123456987 Output: m^n <
4 min read
Logarithm tricks for Competitive Programming
Logarithm: is the inverse function of the exponentiation which means the logarithm value of a given number x is the exponent to another number. [Tex]log_b(x) = y, \to b^{y} = x [/Tex] Below are some tricks using Logarithmic function which can be handy in competitive programming. Checking if a number
15+ min read
Properties of Logarithms
Logarithms serve as essential mathematical tools that help simplify complex calculations, particularly those involving exponential relationships. Understanding the properties of logarithms enables us to solve equations, manipulate algebraic expressions, and better grasp functions in calculus. Here,
4 min read
Logarithmic Differentiation - Continuity and Differentiability
The word continuity means something which is continuous in nature. The flow of water is continuous, time in real life is continuous, and many more instances show the continuity in real life. In mathematics, the Continuous function is the one which when drawn on a graph does not show any breaks and i
5 min read