1. Introduction
The viscous flow and heat transfer in the boundary layer region due to a stretching sheet has wide theoretical and technical applications in manufacturing process and in industries such as extraction of polymer sheet, paper production, wire drawing, glass-fiber production, the cooling and drying of paper and textiles. The study of heat transfer and flow field is necessary for determining the quality of the final products of such process. Crane [1] was first who considered steady boundary layer flow of a viscous incompressible fluid over a linearly stretching plate and gave an exact similarity solution in closed analytical form. The effects of heat and mass transfer and magnetic field under various physical conditions have been investigated by several authors such as Chen and Char [2], Chiam [3], Andersson [4], Ariel et al. [5], Jat and Chaudhary [6,7], Wang [8], Fang et al. [9], Nadeem et al. [10], Bhattacharyya and Layek [11] and recently Bhattacharyya et al. [12] studied the slip effects on boundary layer stagnation-point flow and heat transfer towards a shrinking sheet.
Based on the above mentioned investigations and applications, this paper is concerned with a steady, two dimensional stagnation flow of an electrically conducting fluid past a shrinking sheet in the presence of a magnetic field. The results of velocity and temperature distributions for different parameters such as the velocity ratio parameter, the slip parameter, the Prandtl number, the Eckert number and the magnetic parameter were obtained.
2. Formulation of the Problem
Consider the steady two-dimensional laminar flow of a viscous incompressible electrically conducting fluid towards a linearly shrinking sheet such that the sheet is shrinked in its own plane with velocity proportional to the distance from the stagnation point in the presence of an externally applied normal magnetic field of constant strength . The shrinking surface has a linear velocity
. The shrinking surface has a linear velocity  and uniform temperature
and uniform temperature , while the velocity of the flow external to the boundary layer is
, while the velocity of the flow external to the boundary layer is  and temperature
and temperature  as shown in Figure 1. Therefore, under the usual boundary layer approximations, the governing equations of motion are:
as shown in Figure 1. Therefore, under the usual boundary layer approximations, the governing equations of motion are:
 (1)
(1)
 (2)
(2)
 (3)
(3)
where  is the coefficient of kinematic viscosity,
is the coefficient of kinematic viscosity,  the electrical conductivity,
the electrical conductivity,  the magnetic permeability,
the magnetic permeability,  the density,
the density,  the specific heat at constant pressure,
the specific heat at constant pressure,  the thermal conductivity and
the thermal conductivity and  the coefficient of viscosity. The other symbols have their usual
the coefficient of viscosity. The other symbols have their usual

Figure 1. Physical model of two-dimensional stagnation point flow past a shrinking sheet.
meanings.
The boundary conditions are:
 (4)
(4)
where  is a proportionality constant of the velocity of shrinking sheet,
is a proportionality constant of the velocity of shrinking sheet,  is a slip length and
is a slip length and  is a constant proportional to the free stream velocity for away from the sheet.
is a constant proportional to the free stream velocity for away from the sheet.
3. Analysis of the Velocity and the Thermal Boundary Layers
The continuity Equation (1) is identically satisfied by stream function , defined as
, defined as
 (5)
(5)
For the solution of the momentum and the energy Equations (2) and (3), the following dimensionless variables are defined:
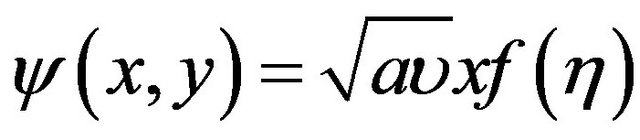 (6)
(6)
 (7)
(7)
 (8)
(8)
Equations (5) to (8), transform Equations (2) and (3) into
 (9)
(9)
 (10)
(10)
where a prime ( ) denotes differentiation with respect to
) denotes differentiation with respect to
 ,
,  is the Magnetic parameter,
is the Magnetic parameter,
 is the Prandtl number and
is the Prandtl number and 
is the Eckert number.
The corresponding boundary conditions are:
 (11)
(11)
where  is the velocity ratio parameter and
is the velocity ratio parameter and
 is the slip parameter.
is the slip parameter.
For numerical solution of the Equations (9) and (10), we apply the following power series in a small magnetic parameter  as:
as:
 (12)
(12)
 (13)
(13)
Substituting Equations (12) and (13) and its derivatives in Equations (9) and (10) and then equating the coefficients of like powers of , we get the following set of equations:
, we get the following set of equations:
 (14)
(14)
 (15)
(15)
 (16)
(16)
 (17)
(17)
 (18)
(18)
 (19)
(19)
with the boundary conditions:
 (20)
(20)
4. Results and Discussion
The Equation (14) is that obtained by Bhattacharyya et al. [12] for the non-magnetic case and the remaining equations are ordinary linear differential equations and have been solved numerically by Newton’s shooting method with fourth-order Runge-Kutta integration scheme for various values of the parameters. The velocity and temperature distributions for various values of parameters are shown in Figures 2-6 respectively.
The velocity profiles  for different values of the velocity ratio parameter
for different values of the velocity ratio parameter , the slip parameter
, the slip parameter 
and the magnetic parameter  are shown in Figures 2-4. It is observed that the velocity boundary layer thickness increases with the increasing values of the velocity ratio parameter
are shown in Figures 2-4. It is observed that the velocity boundary layer thickness increases with the increasing values of the velocity ratio parameter  and the slip parameter
and the slip parameter , whereas it decreases as the magnetic parameter
, whereas it decreases as the magnetic parameter  increases for a fixed
increases for a fixed .
.
The temperature profiles  for different values of the velocity ratio parameter
for different values of the velocity ratio parameter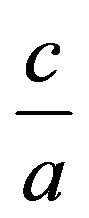 , the slip parameter
, the slip parameter the Prandtl number
the Prandtl number , the Eckert number
, the Eckert number  and the magnetic parameter
and the magnetic parameter  are plotted in Figures 5 and 6. It is observed that for the slip parameter
are plotted in Figures 5 and 6. It is observed that for the slip parameter  the thermal boundary layer thickness decreases with the increasing values of the velocity ratio parameter
the thermal boundary layer thickness decreases with the increasing values of the velocity ratio parameter , the Prandtl number
, the Prandtl number  and the Eckert number
and the Eckert number  and the reverse phenomenon is observed for the magnetic parameter
and the reverse phenomenon is observed for the magnetic parameter .
.
5. Conclusions
In this paper, the stagnation flow for two-dimensional electrically conducting fluid past a shrinking sheet with slip boundaries in the presence of a magnetic field is studied. The similarity equations are derived and solved numerically. It is found that the velocity boundary layer thickness increases with the increasing values of the velocity ratio parameter, the slip parameter. Further we observed that the magnetic parameter decreases with the increasing value of the velocity boundary layer thickness but the reverse phenomenon occurs for thermal boundary layer thickness. Also it observed that the thermal boundary layer thickness decreases with increasing values of the velocity ratio parameter, the Prandtl number and the Eckert number.