1. Introduction
Plate-bending is expertly covered by Timoshenko and Krieger [1], 1959, including the contributions of many other authors. Deflection-rating of plates will continue to rely heavily on these works. The treatise of Arthur W. Leissa [2], 1985, Ohio State University for Wright-Patterson Air Force Base-Flight Dynamics Laboratories gives a comprehensive discourse in buckling, encompassing shear-buckling and the Euler one-dimension case and citing the works and results of many others; no new pure shear solution was offered. Additionally an extensive review of shear buckling in isotropic plates by D.L. Johns [3] is available; the important correlation between the results and von Misses shear was not discussed. Mansour and Thayamballi for the Ship Structure Committee [4] in their 1980-document shed light on pure-shear plate-buckling in relation to the use of stiffeners, as in Figure 1. This present study is assuming that a stiffener-line and a simple-support line behind it amounts to zero-slope boundary,
, in effect clamping. By the intervention of stiffeners the basic all-simply supported plate was interrupted.
Timoshenko’s results for Figure 1 case were quoted [4],
this, as the all-simply-supported plate, appears to make no adjustment for the absence of the stiffeners. For a square plate “
”, this formula persists to date.
Piscopo [5] addressed the pure shear solution in the fashion of Timoshenko where the governing equation, “
” is enhanced as Equation (1)
(1)
but a direct equilibrium solution for the latter has never been found. Incidentally, there appear to be some conflicts between Equation (1) and von Misses yield relation of Equation (2)
(2)
, in the “
” plane
A degenerate form of this equation for “
”, is
or in terms of curvature,
(3)
Find “
” and the pure shear problem is solved in the “von Misses/Kirchhoff’s” framework. So Equation (1) is consumed by Equation (3), leading to,
(4)
![]()
Figure 1. All-simply-supported plate (SSSS) with edge-shears, Nxy. and stiffener-lines.
Equation (4) is now the new standard pure-shear equation.
The present study starts with the buckling problem but assumes a great familiarity in the bending-deflection cases. Deflection factors are related to the desired curvatures. The deflection-factors employed emanate from the capacity of the Kirchhoff’s plate differentials which form the basis of all analyses, analytical or numerical finite-elements; these factors, when found, are easily recognizable, confirming that solutions are on track. Also, a fast approximate spot-buckling-solution is necessary for additional checks; Mohr’s loading curvature-circles (Figure 2) are devised to meet this.
The Kirchhoff’s plate capacity is constant whether in shear or axial compression, so there is no need to engage in a new extensive independent analysis in shear where von Misses shear solution can be invoked. The pure-shear plate buckling is hugely significant on account of the heavy demands on heavier and heavier ships and their platting.
2. Applicable Equations
Equation (5), is the existing uniaxial buckling equation. The biaxial case, Equation (6) ensues if “Nxy” = 0
(5)
(6)
The shear loading—Equation (7) is balanced in the same way as the biaxial case.
(7)
Under equivalent uniformly distributed transverse loading,
, Equation (8) ensues
(8a)
Equations (5)-(8) are summarized as,
(8b)
![]()
Figure 2. Buckling curvature-loading-circles; X-compression (useful for spot-solution). (a)
, critical; (b)
, critical.
2.1. Capacity of the Kirchhoff’s Plate Differentials, “H”
By giving finite values of the left-hand differentials, the capacity of a Kirchhoff’s plate ensues; this is achieved through valid deflection shape-functions, “w”
(9)
(10)
(11)
(12)
(13)
; principal curvatures;
(14a)
Or by reference to the Mohr’s circle,
; R = Mohr’s circle radius (14b)
The average curvature,
, is found significant as an intermediate loading curvature when “
” over the range of “
” in uni-axial X-loading. For the bi-axial case,
.
These integrals are the outcomes of criterion of buckling as relative-curvature/ deflection resonance. A typical buckling resistance integral is,
(15)
The ratio, “
” must always be a scalar or else the function-w is inadmissible. The function-w is chosen as to make the ratio, (
), a scalar. The domain compliant factor at resonance, Cxd4, is what is left to be found. Multiply both sides of Equation (16) and integrate to find it.
(16)
2.2. Buckling Potential Limits
Three possibilities are identified relative to X- and Y-axes in emulating the reactive potentials “
;
;
”.
1)
This is first in contention in uni-axial X-compression; this case easily solves Equation (5).
2)
This is out of contention when no load is applied in the Y-axis, whatever the value of “
”.
3)
This “average loading-curvature” situation will always happen and also in contention. (1) and (3) are identified in the Mohr’s diagram, Figure 2.
In effect, two curvature-loading circles (
,
) are operative and the larger circle gives the required solution for “Nx”. This process softens the stiff constraint that the wave numbers “m, n”, must be whole
2.2.1. The Curvature, “
”, in X-Compression
The solution of Equation (5) is easy when
; and
When
“
” may be interpreted as “effective principal loading curvature”.
So, Figure 2, explains Mohr’s loading-curvature, supplying the critical curvatures, exact or near-exact; exact if
. with X-as major direction of compression.
2.2.2. X-Curvature, “
” from Deflection-Rating
Relying on the deflection coefficients,
,
at two consecutive locations, “i” and “i + 1”, Figure 3(a), the curvature at the second location may be found from Equation (17)
(17)
A2/A1 = CA, stressed boundary lengths-ratio representing side areas: Figure 3(b) and Figure 3(c).
Aspect ratio, “s*” gap of 25-percent can be tolerated.
This equation is similar to Equation (5) as “(Force/Area)(curvature) = Constant” = Kirchhoff’s plate-capacity.
![]() (a)
(a) ![]() (b)
(b) ![]() (c)
(c)
Figure 3. (a) Expected buckling stress-aspect ratio curve; (b) Biaxial loading case; (c) X-axis-only loading.
2.3. Deflection-Factor as Part of Buckling Solution
From Equation (5),
For a given plate-function the “LHS” is invariant and once computed can be used for bending, buckling and vibration; “
” is equivalent uniform transverse pressure. That is, deflection,
the primitive value is sufficient. The familiarity of “
-value” gives confidence the solution is on track.
2.4. Buckling in Pure Shear
Elementary Statics and Pure Shear—“CCCC” Plate
Figure 4(a) for a square plate; the applied pure-shear is transformed into compression/tension loading of an inner square.
Invoke the already known solution on the inner square, Figure 4(a), relying on compression, that is, with Ncr.= 10.66𝜌
2Nxycos45 = (10.66) (1/(0.707b)2); in Ref [2], “10.47” replaces “10.66”
Nxy = 15.08; cf. {14.81ρ, [2] }; the minor difference stems from differences in the deflection shape. Figure 4(b) shows the shear and effective normal stress circles.
For automatic values at any aspect-ratio, simply multiply “
” by the von Misses shear factor of “
” and complete Table 1 or, indeed, Table 2 or any tables.
![]()
Table 1. “CCCC” plate and s*; Nx = DKcr.; “
” from “
”; (
).
![]()
Table 2. “SSSS” plate and s*; Nx = DKcr.; “
” from “
”; (
).
![]() (a)
(a) ![]() (b)
(b)
Figure 4. (a) “CCCC” plate in pure-shear; (b) Shear/effective stress circles.
The “SSSS” case for “s* = 1” in Table 2 show results much smaller than those of Timoshenko, 5.66ρ here to 9.35ρ of Ref. [2].
3. Illustration: Axial Compression
3.1. The “SSCS” Plate, Figure 5
1)
;
; G = 4.5; A = 0.977.
2)
3)
4)
5)
6)
7)
;
;
;
;
; so:
.
Confirm that “
” is correct, cf., (0.0028) [1]; this is important.
Since, also, “
” the result found must be exact or near-exact. Ref. [2] includes the result of “(4.85ρ)” from another source.
Check Pure Shear,
Find “
” by von Misses as
for “SSCS at a/b = 1”.
3.2. The “CCCC”:
-Method
;
;
1) Uniaxial compression; Hxx = 1168.8; Hxy = 779.2;
; Hyy = 1168.8; H = 3116.8;
;
; cf, (0.00126) [1]; the nearness of the primitive-
to the final-
, (0.00128 to 0.00126), confirms “H” on which “Nx” depends. Further, the exactness of the w-function is verified.
2) In pure-shear
; cf (14.81ρ), [2]; note
; at “a/b” = 1.
Note that the “CCCC” plate is summarized in Table 1 for the “
-method” to reflect aspect-ratios; using Equation (17) for “
” from “
”.
The pure-shear strength values varied from “15.0ρ” at s* = 1 to “10.3ρ” at s* = 2.5 (near infinity); these compare well with those of Ref. [2], 14.8ρ to 9.8, respectively.
3.3. The “SSSS” Plate, (Nx from Δ) Is Compiled in Table 2
The trend is similar to the “CCCC” in Table 1; pure-shear results are only 60-percent of existing.
Brief Application to Free-Vibration in the “SSSS”
This is elaborated in Columns 10 and 11 in Table 2. Find mass-factor
and,
. Illustration is for m = n = 1, first.
3.4. The “CSCS” Plate in Pure-Shear
;
,
a/b = 1: Hxx = 612; Hxy = 306; Hyy = 114.75; H = 1032.75;
, cf, 0.00192 [1];
;
;
;
, expected to be exact.
Table 3 elaborates the “CSCS” case in the same fashion as the “CCCC” plate in Table 1. The results for pure-shear match the literature “SSSS” values and so the “SSSS” literature-values are untenable. The real “SSSS” values are as given in Table 2 and are about 60-percent of those in Table 3. Comparing ratios,
and
; these ratios are expected to be of the same order; and they are.
3.5. Deflection Limit
In Tables 1-3, the factor,
, exhibits a critical stationary-point, Figure 6, where “
” is sampled. The minimum buckling load, for very long plates,
![]()
Table 3. “CSCS” plate and s*; Nx = DKcr.; “
” from “
”; (
).
![]()
Figure 6. Curvature-displacement-rate (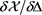 ) versus displacement (
) versus displacement ( ).
).
is indicated at that point, whatever the values of “m, n or s*”. The relative weakness of a plate is indicated in bending-buckling-vibration-analyses [6] - [12]. By Yaghoobi [13] the buckling strength of the “SSSS” at s* = 1.5 is 91-percent of the value at s* = 1; this is the kind of statement sought-after here. This sits well with the ratio of 89-percent in Table 2. In “plate buckling solution based on pre-buckling deflection” [14], relevance of deflection was focused on. Here, the analysis starts with beam-strip solutions that are already fully known; for simply supported strip, c = 5/384 = 0.01302 and the “SSSS” plate ends in this value when it is very long or very short; for example check the  in Table 2 in the “SSSS” at s* = 3.5; so the size of “
in Table 2 in the “SSSS” at s* = 3.5; so the size of “ ” can, also, be used to terminate solutions.
” can, also, be used to terminate solutions.
3.6. Higher Modes in Buckling: The “SSSS”
Table 2 has already solved this problem, relying only on the fundamental wave, m = n = 1, but the question may be posed: what is the failure mode for a given aspect ratio? In combining two neighboring symmetrical waves, the tried Dunkerley’s approximate resultant is used to study this question.
For example: s* = 2.5, try two waves, placed between the actions, 1)  , or C2.5,1; and 2) m = 3,
, or C2.5,1; and 2) m = 3, ; in details:
; in details:
1) C2.5,1: Hxx = 1.54; Hxy = 19.2; Hyy = 60.1; H = 80.8;![]() ;
;![]() ;
;![]() ;
;![]() .
.
2) C2.5,3: Hxx = 374; Hxy = 519; Hyy = 180.2; H = 1073;![]() ;
; ![]()
Combining by Dunkerley’s:
![]() , cf, 2.91 in Table 2 above. This is a fail-safe combination.
, cf, 2.91 in Table 2 above. This is a fail-safe combination.
So, it can be said that the waves “m = 1 and m = 3” combine for the aspect ratio, s* = 2.5; N2.5,1,3 = 2.77ρ. This result is very different from the reference value of “4ρ” [1] [2]. In this way the complementary question of failure-mode is answered after the strength-solution.
4. Conclusions
1) The finite “Capacity” of the Kirchhoff’s plate differentials is constant in shear, in plate-buckling, in pure-shear plate-buckling, among others; compliant deflection functions supply domain relations.
2) The von Misses shear condition was shown to correlate exactly with the behavior of all-clamped rectangular plate in pure shear.
3) Using the same method new results are found for the “SSSS” plate; they are about 60-percent of currently held values. The imposition of stiffeners introduces boundary conditions different from the “SSSS” and so the present results, without stiffeners, appear more realistic.
4) The presently held “SSSS” shear values are, here, found corresponding to those of a plate clamped on Y-Y and simply-supported on the long side, X-X, with very good accuracy.
5) It is, therefore, concluded that the pure-shear results for the “SSSS” plate had not been found until the new results presented here: for “a/b = 1”, Nxy = 5.66ρ and not 9.3ρ. The difference is huge with respect to safety and frequency of maintenance of vessels.
Nomenclature
a, b: rectangular plate dimensions in X, Y
s*: aspect ratio, a/b
E: Young’s modulus of elasticity
![]()
t: thickness of plate
D: flexural rigidity of plate, (isotropic); ![]()
![]() : poisson’s ratio
: poisson’s ratio
w: deflection symbol; wsh = shape-function value
![]() : general value of displacement;
: general value of displacement; ![]() = small change in the displacement
= small change in the displacement
wxx-r; wyy-r: relative curvature in X-direction; Y-direction
wxx-r/w: relative-curvature/deflection ratio; must be a scalar for any solution
XX-SC, YY-CC: plate simply and clamped on X-X; and clamped-clamped on Y-Y
rcap: capacity ratio of axes as, ![]()
![]()
![]() : curvature;
: curvature; ![]() = small change in the curvature;
= small change in the curvature; ![]() = von Misses effective curvature
= von Misses effective curvature
m, n: wave numbers
![]() ; Ncr: critical stress symbol; critical buckling load symbol
; Ncr: critical stress symbol; critical buckling load symbol