The modern matchstick was invented in the early 1800s. The initial iterations suffered from assorted defects like violent flares, rancid chemicals, and a tendency to cause factory workers’ jaws to rot. Once they ironed out the kinks, the little torches spread like wildfire. To distinguish themselves in a new competitive market, many companies began to sell their matchsticks in funky, colorful boxes. Among the attention-grabbing designs that now decorate phillumenists’ collections were boxes with puzzles. Some brands would print games on the box that consumers could play with the matches inside. The matchstick puzzle was born.
Matchstick puzzles typically include a diagram of matches and a challenge prompt, which may involve moving, adding, or subtracting matches to achieve a goal. They often look simple but can be deceptively challenging. The puzzles were a hit—people compiled books of them, and they remain a subgenre that puzzlers still develop to this day. I’ve given you a few enticing ones this week to ignite a fire in your belly.
Did you miss last week’s puzzle? Check it out here, and find its solution at the bottom of today’s article. Be careful not to read too far ahead if you haven’t solved last week’s yet!
Puzzle #12: Matchstick Puzzles
Here are three matchstick puzzles to test your wits.
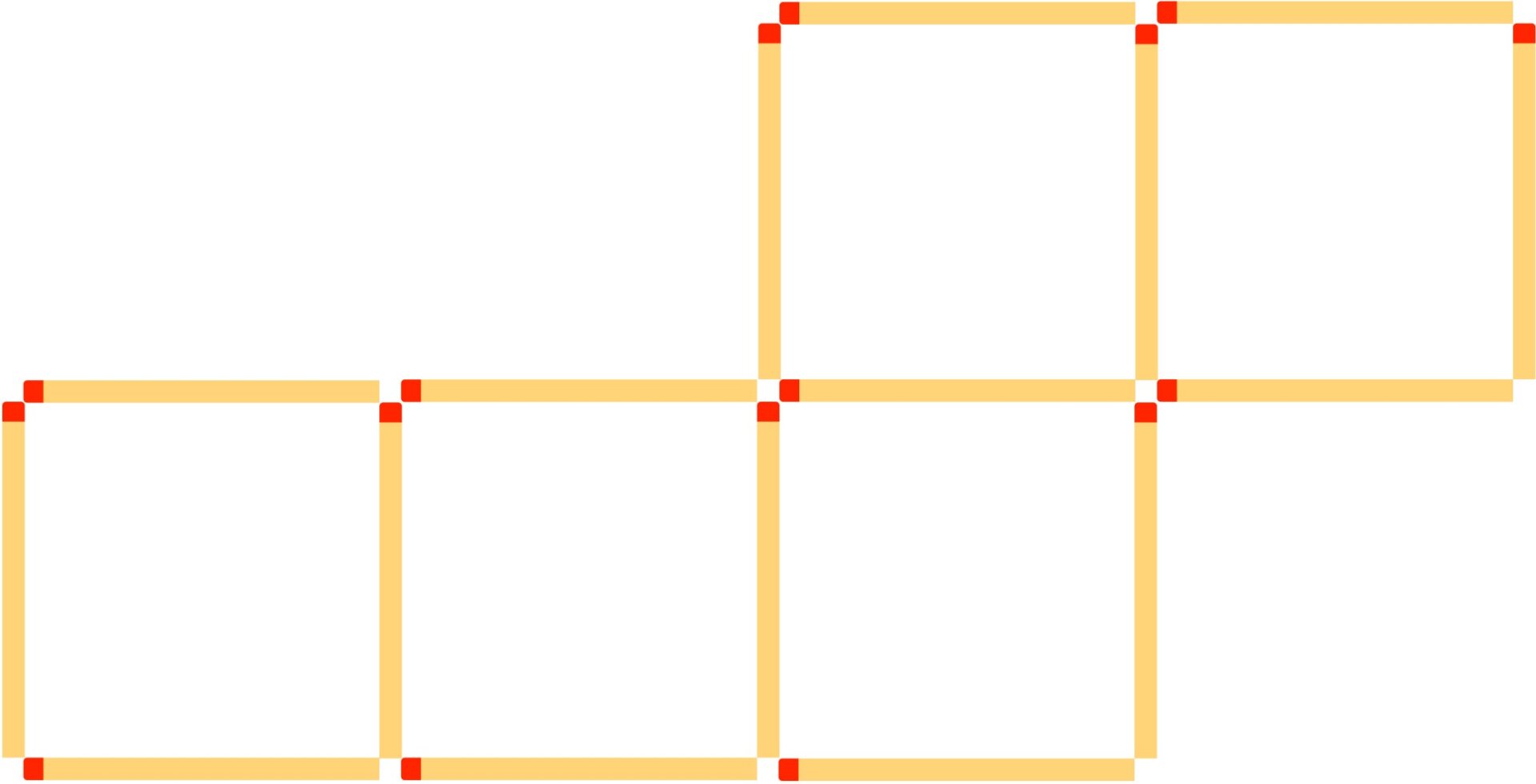
Move two matchsticks so that four one-by-one squares remain. The two matchsticks must be placed somewhere in the diagram and contribute to the final image.
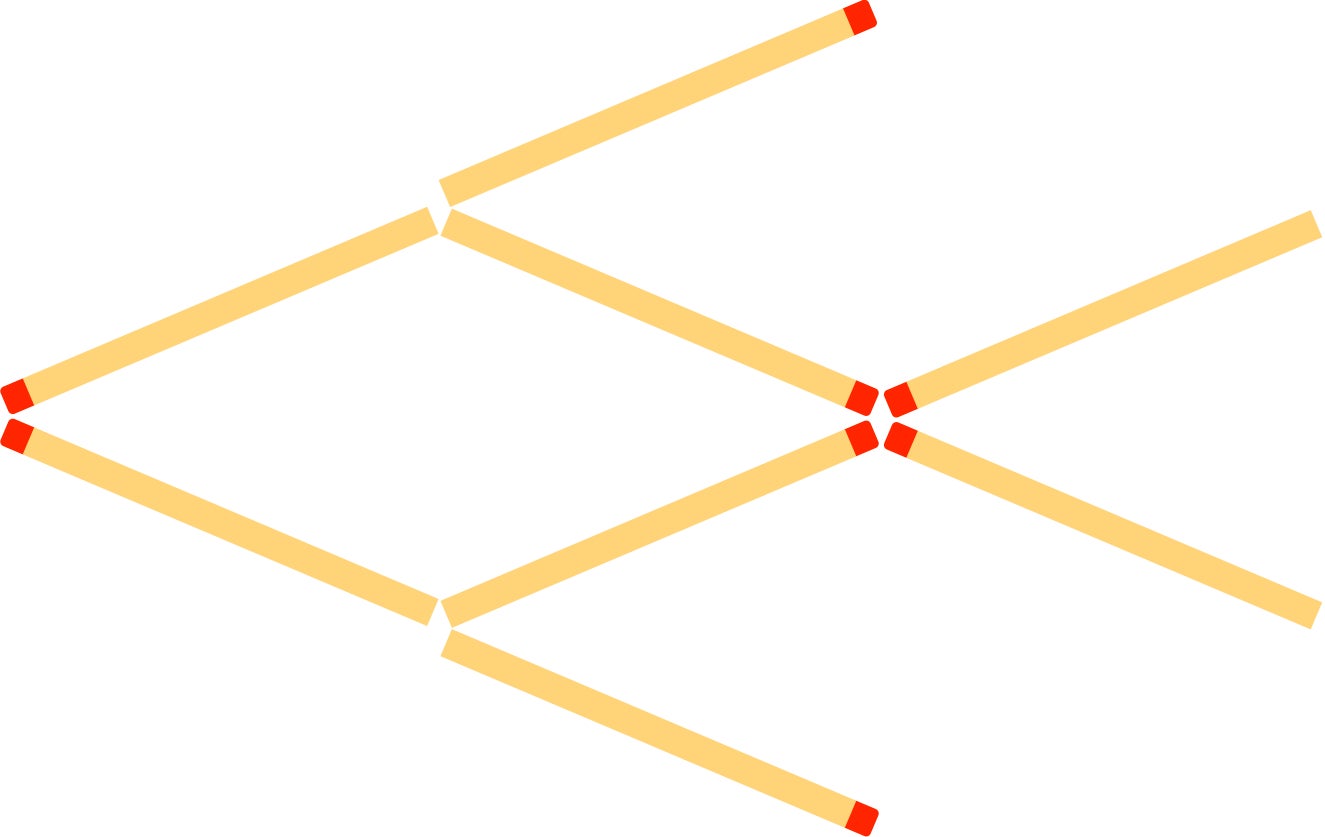
This is a fish swimming to the left. Move three matchsticks so that the same fish pattern swims to the right.
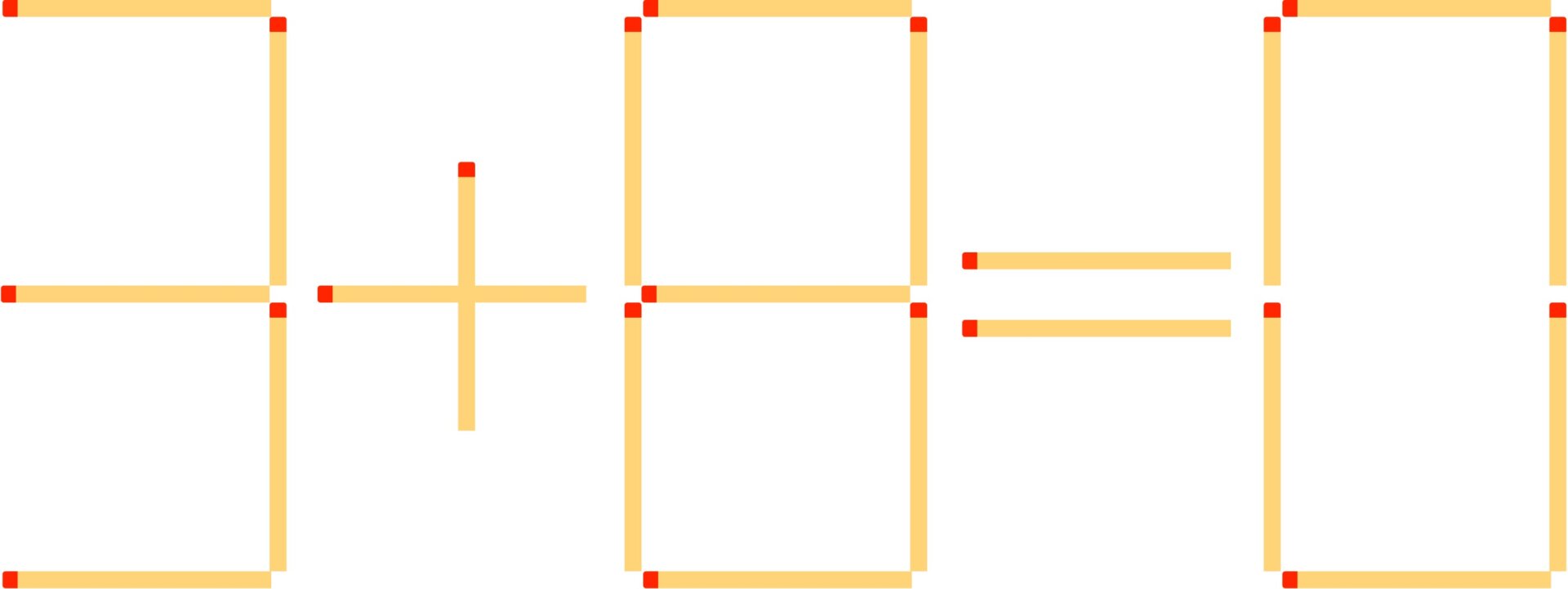
Above, matchsticks depict numbers like on a digital clock. The equation 3+8=0 is incorrect. Remove only two matchsticks to form a true equation.
I’ll be back next week with the solutions and a new puzzle. Do you know a cool puzzle that I should cover here? Send it to me at [email protected]
Solution to Puzzle #11: Smullyan’s Truth Machines
Last week, I posed a difficult logic problem about truth-telling machines in a magic shop. Shout-out to reader bbe for nailing the solution. Let’s call the machines A, B, and C, and suppose that you choose to ask machine A your question. A key idea is to declare to yourself that you will not walk home with machine A. This way, if A happens to be the broken machine and you heed its faulty advice, it won’t matter, because both of the other machines work. With this strategy, you are guaranteed to succeed in the case that A is broken. So we can assume for the rest of the argument that the machine you ask is not the broken machine.
Now you need to ask one question to machine A to determine which of machines B and C is functioning. The question will start with the phrase, “Is exactly one of the following two statements true….” If you haven’t already solved the puzzle, would you like to pause here and attempt to finish the solution?
The question is, “Is exactly one of the following two statements true: the blue light on machine A means ‘true’ and machine B is a functioning machine?” There are four possibilities. The blue light on machine A could mean “true” or “false,” and in each case machine B could be functioning or broken. The table below records what color light a functioning machine A will illuminate in each of these cases:
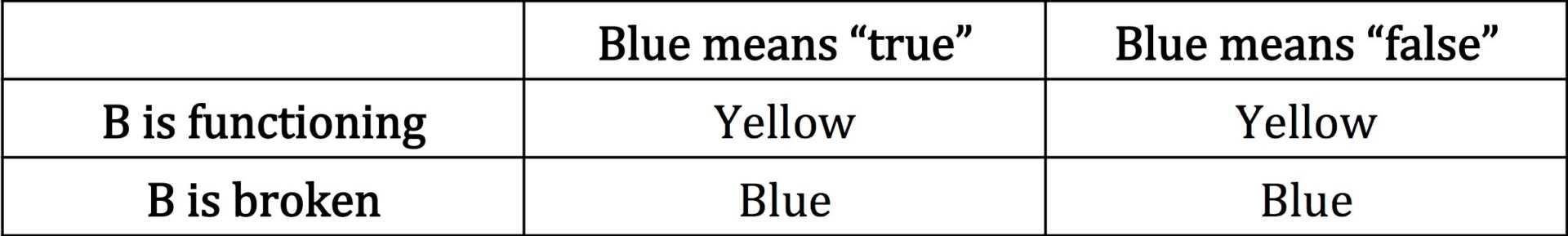
For example, the upper left entry in the table corresponds to the case where the blue light on machine A means “true” and machine B is a functioning machine. In this case, the answer to your question is “false” because the statements “the blue light on machine A means ‘true’” and “machine B is a functioning machine” are both true, so it is not the case that exactly one of the statements is true (there is a lot of logic at work here, so it might be worth rereading the preceding sentences to make sure you understand what the table means and why the entries are as written). Since the answer to your question is false, and A’s blue light corresponds to “true” in this case, that means A will illuminate its yellow light (to communicate “false”). This is why the entry in the upper left cell of the table is “Yellow.”
For another example, consider the upper right entry. Now the answer to your question is “true” because exactly one of your proposed statements is true (B is functioning, but blue does not mean “true”). So machine A will communicate “true,” which in this case corresponds to its yellow light. The final two entries in the table can be verified similarly.
Looking at the table, we see that if A illuminates its yellow light, in both cases it means that B is a functioning machine, whereas a blue light always corresponds to B being broken. So after asking your question, if you see a yellow light, you’ll choose machine B, and if you see a blue light, you’ll choose machine C. Remember, if A is just spouting random answers and you follow its advice, then whether you pick B or C, you’re always a winner. Big kudos if you managed to crack this one.